Question

[Nguyễn Thanh Huyền]

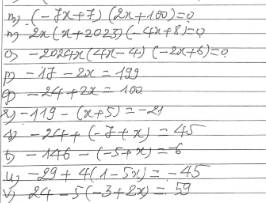
cần giúp gấp vs ạ, em cảm ơn

Answer 1

[Nguyễn Lê Phước Thịnh]

m: \(\left(-7x+7\right)\left(2x+100\right)=0\)

=>\(\left[{}\begin{matrix}-7x+7=0\\2x+100=0\end{matrix}\right.\)

=>\(\left[{}\begin{matrix}-7x=-7\\2x=-100\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-50\end{matrix}\right.\)

n: \(2x\left(x+2023\right)\left(-4x+8\right)=0\)

=>\(2\cdot x\left(x+2023\right)\cdot\left(-4\right)\left(x-2\right)=0\)
=>x(x-2)(x+2023)=0

=>\(\left[{}\begin{matrix}x=0\\x-2=0\\x+2023=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=2\\x=-2023\end{matrix}\right.\)

o: \(-2024x\left(4x-4\right)\left(-2x+6\right)=0\)

=>\(x\left(4x-4\right)\left(-2x+6\right)=0\)

=>\(x\cdot4\left(x-1\right)\cdot\left(-2\right)\left(x-3\right)=0\)

=>x(x-1)(x-3)=0

=>\(\left[{}\begin{matrix}x=0\\x-1=0\\x-3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\\x=3\end{matrix}\right.\)

p: -17-2x=199

=>2x=-17-199=-216

=>x=-216/2=-108

q: -24+2x=100

=>2x=100+24=124

=>\(x=\dfrac{124}{2}=62\)

r: \(119-\left(x+5\right)=-21\)

=>\(x+5=119-\left(-21\right)=119+21=140\)

=>x=140-5=135

s: \(-24+\left(-7+x\right)=45\)

=>\(\left(x-7\right)-24=45\)

=>x-31=45

=>x=45+31=76

t: \(-146-\left(-5+x\right)=-6\)

=>\(x-5=-146-\left(-6\right)=-140\)

=>x=-140+5=-135

u: \(-29+4\left(1-5x\right)=-45\)

=>4(1-5x)=-45+29=-16

=>1-5x=-4

=>5x=1+4=5

=>\(x=\dfrac{5}{5}=1\)

v: \(24-5\left(-3+2x\right)=59\)

=>\(24-5\left(2x-3\right)=59\)

=>5(2x-3)=24-59=-35

=>2x-3=-7

=>2x=-4

=>x=-4/2=-2

Answer 2

[Hoàng Văn Tiến]

-17-2x=199

<=>-2x=216

<=>x=-108

-24+2x=100

<=>2x=124

<=>x=62

-24+(-7+x)=45

<=>-7+x=69

<=>x=76

-146-(-5+x)=-6

<=>-146+5-x=-6

<=>x=-135

-29+4(1-5x)=-45

<=>-29+4+20x=-45

<=>20x=-20

<=>x=-1

24-5(-3+2x)=59

<=>24+15-10x=59

<=>-10x=20

<=>x=-2