cho hai đa thức:A(x)=3x3+5-6x+5x2
B(x)=4x2+6x-2x7-9
a) tìm giá trị của đa thức:A(x),khi x=2
b)tính A(x)+B(x)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
b) x2 – 4x +3
18 tháng 6 2023
a: P(x)=6x^3-4x^2+4x-2
Q(x)=-5x^3-10x^2+6x+11
M(x)=x^3-14x^2+10x+9
b: \(C\left(x\right)=7x^4-4x^3-6x+9+3x^4-7x^3-5x^2-9x+12\)
=10x^4-11x^3-5x^2-15x+21
H9
HT.Phong (9A5)
CTVHS
17 tháng 7 2023
Câu 1:
\(A\left(x\right)+B\left(x\right)\)
\(=\left(6x-4x^3+x-1\right)+\left(-3x-2x^3-5x^2+x+2\right)\)
\(=\left(6x+-3x+x\right)-\left(4x^3+2x^3\right)-5x^2+\left(-1+2\right)\)
\(=-6x^3-5x^2+4x+1\)
\(A\left(x\right)-B\left(x\right)\)
\(=\left(6x-4x^3+x-1\right)-\left(-3x-2x^3-5x^2+x+2\right)\)
\(=\left(-4x^3+2x^3\right)+5x^2+\left(6x+x-x\right)+\left(-1-2\right)\)
\(=-2x^3+5x^2+6x-3\)
29 tháng 10 2023
a: \(A=x^2-4=\left(x-2\right)\left(x+2\right)\)
Khi x=102 thì \(A=\left(102-2\right)\left(102+2\right)=104\cdot100=10400\)
b: \(B=x^2+6x+9=x^2+2\cdot x\cdot3+3^2=\left(x+3\right)^2\)
Khi x=997 thì \(B=\left(997+3\right)^2=1000^2=1000000\)
c: \(C=4x^2-4xy+y^2=\left(2x\right)^2-2\cdot2x\cdot y+y^2=\left(2x-y\right)^2\)
Khi x=39 và y=-2 thì \(C=\left(2\cdot39+2\right)^2=80^2=6400\)
30 tháng 3 2022
a: \(C\left(x\right)=A\left(x\right)+B\left(x\right)\)
\(=3x^4-4x^3+5x^2-4x-3-3x^4+4x^3-5x^2+2x+6\)
=-2x+3
b: Đặt C(x)=0
=>-2x+3=0
hay x=3/2
KT
1
9 tháng 9 2021
\(1,\\ a,A=4x^2\left(-3x^2+1\right)+6x^2\left(2x^2-1\right)+x^2\\ A=-12x^4+4x^2+12x^2-6x^2+x^2=-x^2=-\left(-1\right)^2=-1\\ b,B=x^2\left(-2y^3-2y^2+1\right)-2y^2\left(x^2y+x^2\right)\\ B=-2x^2y^3-2x^2y^2+x^2-2x^2y^3-2x^2y^2\\ B=-4x^2y^3-4x^2y^2+x^2\\ B=-4\left(0,5\right)^2\left(-\dfrac{1}{2}\right)^3-4\left(0,5\right)^2\left(-\dfrac{1}{2}\right)^2+\left(0,5\right)^2\\ B=\dfrac{1}{8}-\dfrac{1}{4}+\dfrac{1}{4}=\dfrac{1}{8}\)
9 tháng 9 2021
\(2,\\ a,\Leftrightarrow10x-16-12x+15=12x-16+11\\ \Leftrightarrow-14x=-4\\ \Leftrightarrow x=\dfrac{2}{7}\\ b,\Leftrightarrow12x^2-4x^3+3x^3-12x^2=8\\ \Leftrightarrow-x^3=8=-2^3\\ \Leftrightarrow x=2\\ c,\Leftrightarrow4x^2\left(4x-2\right)-x^3+8x^2=15\\ \Leftrightarrow16x^3-8x^2-x^3+8x^2=15\\ \Leftrightarrow15x^3=15\\ \Leftrightarrow x^3=1\Leftrightarrow x=1\)
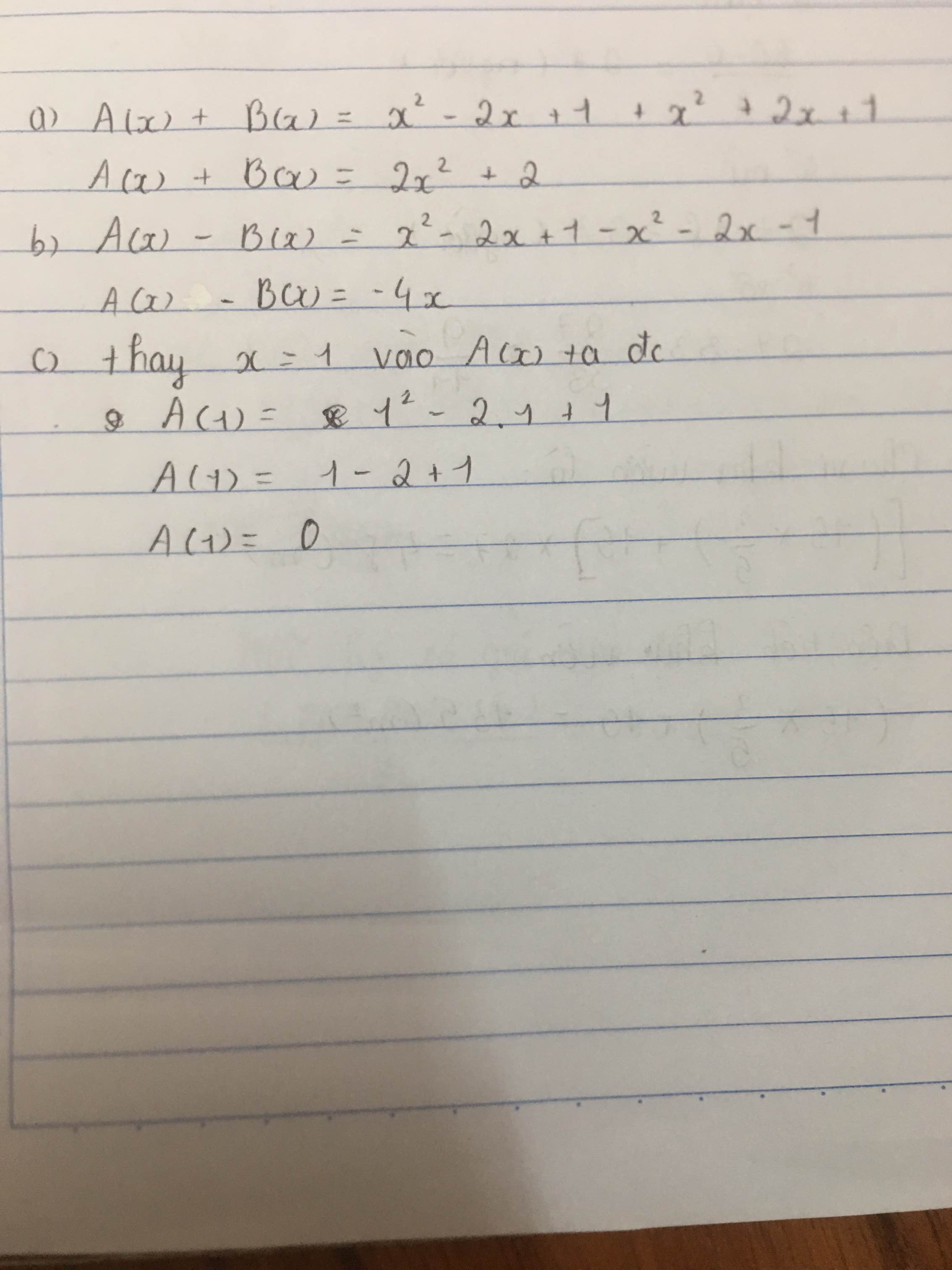
a, Thay x=2 vào A, ta được:
\(A\left(2\right)=3.2^3+5-6.2+5.2^2=37\)
Vậy A= 37 khi x=2.
b,
\(A\left(x\right)+B\left(x\right)=\left(3x^3+5-6x+5x^2\right)+\left(4x^2+6x-2x^7-9\right)\\ =-2x^7+3x^3+9x^2-4\)