Giải giúp mình câu 1, 2 với ạ. 
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



Câu 2
a) nH2 = \(\dfrac{4,48}{22,4}=0,2mol\)
nancol = 2nH2 = 0,4 mol
=> Mancol = \(\dfrac{17}{0,4}=42,5\)
=> Hai ancol là CH3OH và C2H5OH
b) số ete thu được tối đa = \(\dfrac{n\left(n+1\right)}{2}\)
= \(\dfrac{2.3}{2}=3\)

6 How often does your grandfather often listen to the radio
7 How often do you go to the gym
8 What did they send
9 What time did he leave the party
10 Where did he go
11 Who did he meet for coffee
12 Why did she called the firemen
13 How did he go to school
14 What does lan like doing
15 Where was your father last months
16 How much is the dictionary
17 How long did it take to finish the composition
18 Who bought a poster
What did Mrs Robinson by
19 Where is a restaurant
20 What did her neighbor give her
21 How was the homework yesterday
22 Do you have magaazine and newspapers
23 How often do you go to the movies

5:
a: góc ABO+góc ACO=180 độ
=>ABOC nội tiếp
b: Xét ΔABE và ΔADB có
góc ABE=góc ADB
góc BAE chung
=>ΔABE đồng dạng với ΔADB
=>AB^2=AE*AD
Xet (O) có
AB,AC là tiếp tuyến
=>AB=AC
mà OB=OC
nên OA là trung trực của BC
=>AH*AO=AB^2=AE*AD
=>AE/AO=AH/AD

\(n_{H_2}=\dfrac{2,24}{22,4}=0,1\left(mol\right)\)
PTHH:
Zn + 2HCl ---> ZnCl2 + H2
0,1<-0,2<----------------0,1
\(n_{ZnO}=\dfrac{10,55-0,1.65}{81}=0,05\left(mol\right)\)
ZnO + 2HCl ---> ZnCl2 + H2
0,05-->0,1
\(C_{M\left(HCl\right)}=\dfrac{0,1+0,2}{0,2}=1.5M\)

\(\Leftrightarrow\sqrt{4x^2-4x+1}=3x-1\)
\(\Leftrightarrow\left\{{}\begin{matrix}3x-1\ge0\\4x^2-4x+1=\left(3x-1\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge\dfrac{1}{3}\\5x^2-2x=0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x\ge\dfrac{1}{3}\\\left[{}\begin{matrix}x=0\left(loại\right)\\x=\dfrac{2}{5}\end{matrix}\right.\end{matrix}\right.\)
Vậy \(x=\dfrac{2}{5}\)


Bài 4:
b: Xét ΔABK vuông tại A có AD là đường cao ứng với cạnh huyền BK
nên \(BD\cdot BK=BA^2\left(1\right)\)
Xét ΔABC vuông tại A có AH là đường cao ứng với cạnh huyền BC
nên \(BH\cdot BC=AB^2\left(2\right)\)
Từ (1) và (2) suy ra \(BD\cdot BK=BH\cdot BC\)




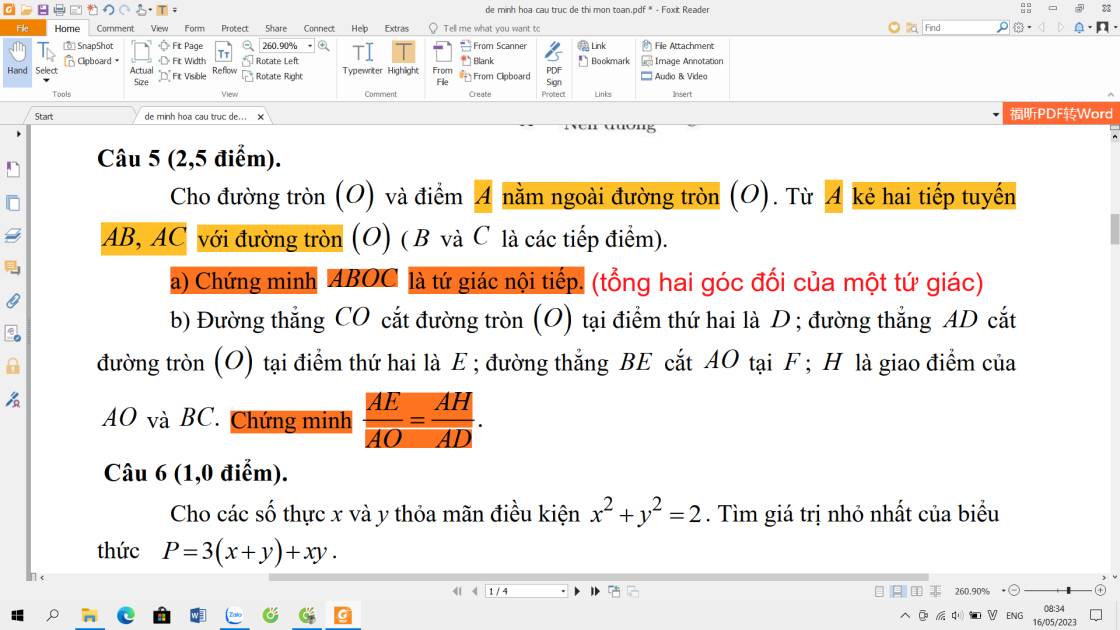 giải giúp mình 2 câu này với ạ
giải giúp mình 2 câu này với ạ

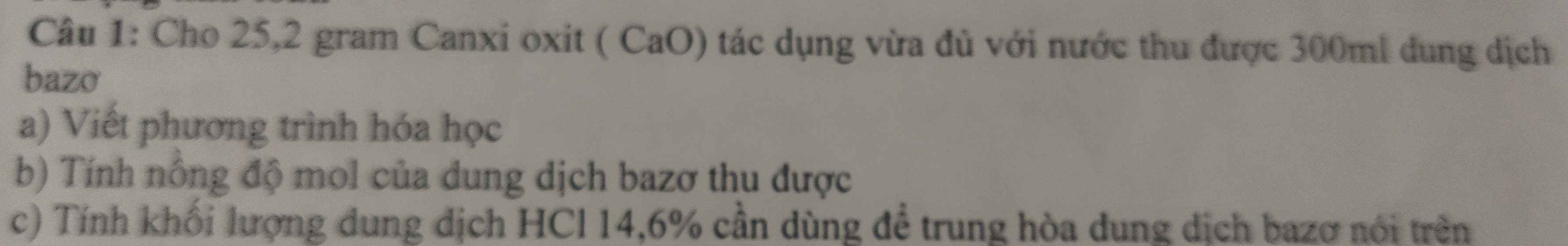
 Mn giúp có thể giúp mình câu C bài 4 và bài 5 được ko ạ, giải chi tiết 1 chút với ạ. Mình cảm ơn
Mn giúp có thể giúp mình câu C bài 4 và bài 5 được ko ạ, giải chi tiết 1 chút với ạ. Mình cảm ơn