Giúp mình câu b thôi ạ
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
LA
1
31 tháng 7 2021
b) Để P nguyên thì \(\sqrt{x}+5⋮3\sqrt{x}-1\)
\(\Leftrightarrow3\sqrt{x}+15⋮3\sqrt{x}-1\)
\(\Leftrightarrow16⋮3\sqrt{x}-1\)
\(\Leftrightarrow3\sqrt{x}-1\in\left\{-1;1;2;4;8;16\right\}\)
\(\Leftrightarrow3\sqrt{x}\in\left\{0;2;3;5;9;17\right\}\)
\(\Leftrightarrow3\sqrt{x}\in\left\{0;3;9\right\}\)
\(\Leftrightarrow\sqrt{x}\in\left\{0;1;3\right\}\)
hay \(x\in\left\{0;1;9\right\}\)
20 tháng 6 2023
b: BC=2*5=10cm
FC=3/5AF
=>AF/FC=5/3
=>AF/AC=5/8
EF//BC
=>EF/BC=AF/AC
=>EF/10=5/8
=>EF=50/8=25/4cm
2 tháng 7 2023
b: góc C=90-55=35 độ
góc AGI=góc BGH=90-55=35 độ
góc IGH=180-35=145 độ
5 tháng 9 2021
Bài 3:
a: Ta có: \(P=\left(\dfrac{2}{\sqrt{x}+2}-\dfrac{1}{\sqrt{x}+1}\right):\dfrac{x}{x\sqrt{x}-\sqrt{x}}\)
\(=\dfrac{2\sqrt{x}+2-\sqrt{x}-2}{\left(\sqrt{x}+2\right)\left(\sqrt{x}+1\right)}\cdot\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{x}\)
\(=\dfrac{\sqrt{x}-1}{\sqrt{x}+2}\)
PC
1
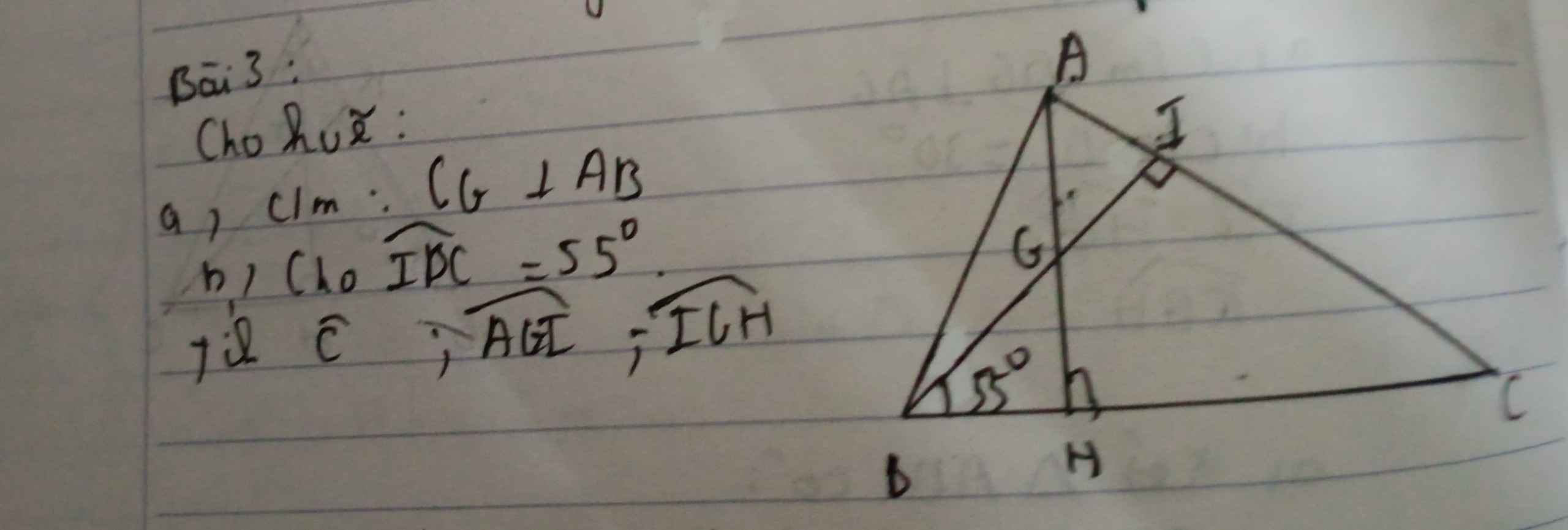
\(\lim\dfrac{\sqrt{3n^2+1}+n}{\sqrt{2n^2-3}+n+1}=\lim\dfrac{n\sqrt{3+\dfrac{1}{n^2}}+n}{n\sqrt{2-\dfrac{3}{n^2}}+n+1}\)
\(=\lim\dfrac{n\left(\sqrt{3+\dfrac{1}{n^2}}+1\right)}{n\left(\sqrt{2-\dfrac{3}{n^2}}+1+\dfrac{1}{n}\right)}=\lim\dfrac{\sqrt{3+\dfrac{1}{n^2}}+1}{\sqrt{2-\dfrac{3}{n^2}}+1+\dfrac{1}{n}}=\dfrac{\sqrt{3}+1}{\sqrt{2}+1}\)