Mọi người giải chi tiết cho mik với! Mik cảm ơn trước...
Đọc tiếp
Mọi người giải chi tiết cho mik với! Mik cảm ơn trước ạ!
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
QN
1
Những câu hỏi liên quan
6 tháng 8 2023
a: \(Q=\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}+2\right)-2\sqrt{x}\left(\sqrt{x}-2\right)-5\sqrt{x}-2}{x-4}:\dfrac{\sqrt{x}\left(3-\sqrt{x}\right)}{\left(\sqrt{x}+2\right)^2}\)
\(=\dfrac{x+3\sqrt{x}+2-2x+4\sqrt{x}-5\sqrt{x}-2}{x-4}\cdot\dfrac{\left(\sqrt{x}+2\right)^2}{\sqrt{x}\left(3-\sqrt{x}\right)}\)
\(=\dfrac{-x+2\sqrt{x}}{\sqrt{x}-2}\cdot\dfrac{\sqrt{x}+2}{\sqrt{x}\left(3-\sqrt{x}\right)}\)
\(=\dfrac{-\sqrt{x}\left(\sqrt{x}-2\right)}{\sqrt{x}\left(\sqrt{x}-2\right)\cdot\left(-1\right)}\cdot\dfrac{\sqrt{x}+2}{\sqrt{x}-3}=\dfrac{\sqrt{x}+2}{\sqrt{x}-3}\)
b: Khi x=4-2căn 3 thì \(Q=\dfrac{\sqrt{3}-1+2}{\sqrt{3}-1-3}=\dfrac{\sqrt{3}+1}{\sqrt{3}-4}=\dfrac{-7-5\sqrt{3}}{13}\)
c: Q>1/6
=>Q-1/6>0
=>\(\dfrac{\sqrt{x}+2}{\sqrt{x}-3}-\dfrac{1}{6}>0\)
=>\(\dfrac{6\sqrt{x}+12-\sqrt{x}+3}{6\left(\sqrt{x}-3\right)}>0\)
=>\(\dfrac{5\sqrt{x}+9}{6\left(\sqrt{x}-3\right)}>0\)
=>căn x-3>0
=>x>9
9 tháng 1 2023
a: Xét ΔABC có BM/BC=BD/BA
nên MD//AC
=>MM' vuông góc AB
=>M đối xứngM' qua AB
b: Xét tứ giác AMBM' có
D là trung điểm chung của AB và MM'
MA=MB
Do đó: AMBM' là hình thoi
6 tháng 1 2023
a: ĐKXĐ: x<>2; x<>-3
b: \(P+\dfrac{x^2-4-5-x-3}{\left(x-2\right)\left(x+3\right)}=\dfrac{\left(x-4\right)\left(x+3\right)}{\left(x-2\right)\left(x+3\right)}=\dfrac{x-4}{x-2}\)
c: Để P=-3/4 thì x-4/x-2=-3/4
=>4x-8=-3x+6
=>7x=14
=>x=2(loại)
e: x^2-9=0
=>x=3 (nhận) hoặc x=-3(loại)
Khi x=3 thì \(P=\dfrac{3-4}{3-2}=-1\)
10 tháng 1 2023
a: Xét tứ giác ADHE có
góc ADH=góc AEH=góc DAE=90 độ
nên ADHE là hình chữ nhật
b: \(HD=\sqrt{10^2-8^2}=6\left(cm\right)\)
\(S_{ADHE}=6\cdot8=48\left(cm^2\right)\)
c: Để ADHE là hình vuông thì AH là phân giác của góc BAC
=>góc B=45 độ
DX
24 tháng 12 2022
\(\dfrac{1}{x^2-4}+\dfrac{2x}{x+2}=\dfrac{1}{\left(x-2\right)\left(x+2\right)}+\dfrac{2x}{x+2}=\dfrac{1+2x\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}=\dfrac{1+2x^2-4x}{\left(x+2\right)\left(x-2\right)}\)
trên bài mink đã ẩn đi bước quy đồng!!
\(\dfrac{18}{\left(x-3\right)\left(x^2-9\right)}-\dfrac{3}{x^2-6x+9}-\dfrac{x}{x^2-9}=\dfrac{18}{\left(x-3\right)\left(x+3\right)\left(x-3\right)}-\dfrac{3}{\left(x-3\right)^2}-\dfrac{x}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{18}{\left(x-3\right)^2\left(x+3\right)}-\dfrac{3}{\left(x-3\right)^2}-\dfrac{x}{\left(x-3\right)\left(x+3\right)}=\dfrac{18-3\left(x+3\right)-x\left(x-3\right)}{\left(x-3\right)^2\left(x+3\right)}\)
\(=\dfrac{18-3x-9-x^2+3x}{\left(x-3\right)^2\left(x+3\right)}=\dfrac{9-x^2}{\left(x-3\right)^2\left(x+3\right)}=\dfrac{-\left(x-3\right)\left(x+3\right)}{\left(x-3\right)^2\left(x+3\right)}=\dfrac{-1}{x-3}\)
10 tháng 1 2023
a: \(=\dfrac{x^3+2x+2x-2-x^2-x-1}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{x^3-x^2+3x-3}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x^2+3}{x^2+x+1}\)
b: \(=\dfrac{x^2-2x-3+x^2+2x-3+2x-2x^2}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{2x-6}{\left(x-3\right)\left(x+3\right)}=\dfrac{2}{x+3}\)
c: \(=\dfrac{6-7+x}{3\left(x-1\right)}=\dfrac{x-1}{3\left(x-1\right)}=\dfrac{1}{3}\)
d: \(=\dfrac{x^3+2x+2x-2-x^2-x-1}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x^3-x^2+3x-3}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x^2+3}{x^2+x+1}\)
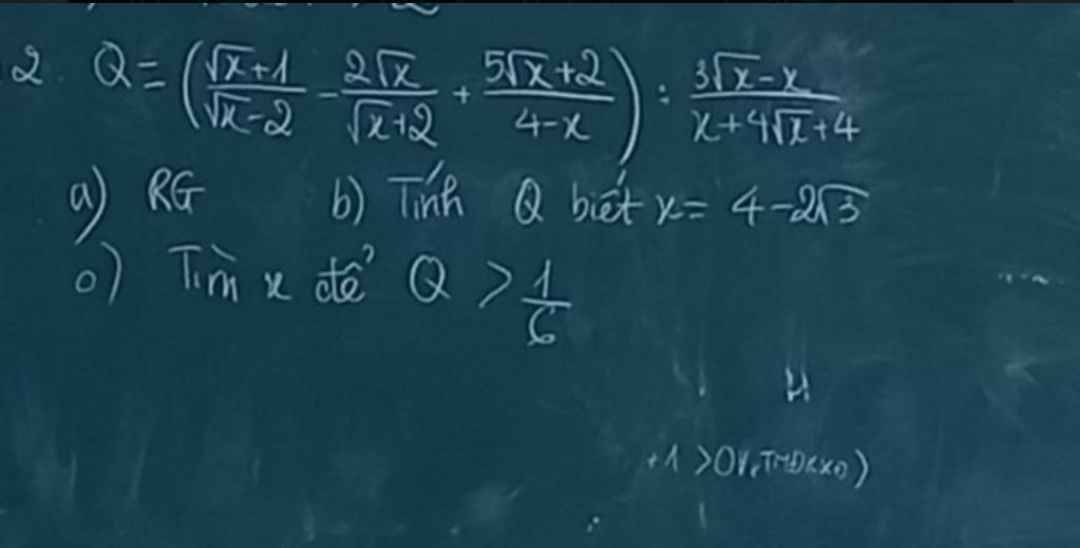
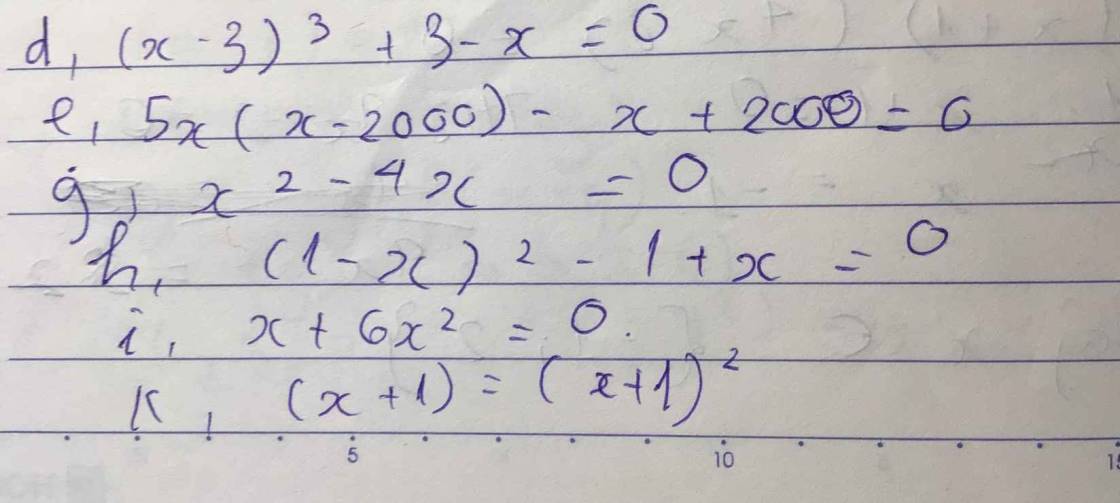
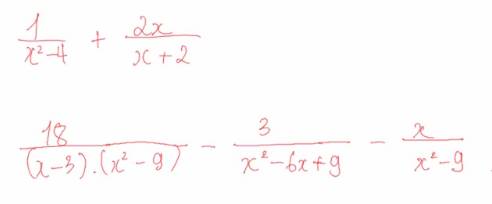
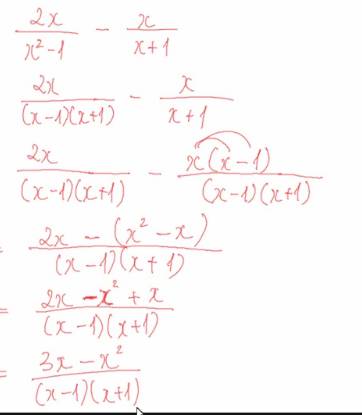
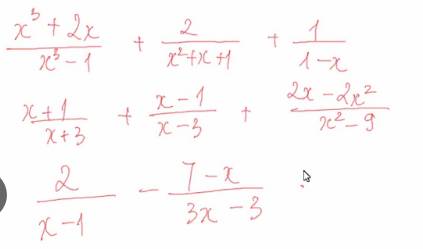
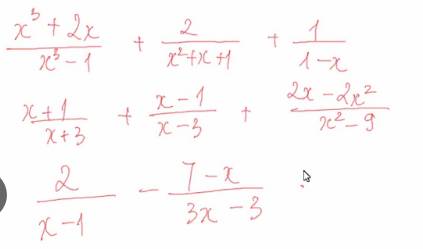
\(d_1\) nhận \(\overrightarrow{u_1}=\left(3;1\right)\) là 1vtcp
\(d_2\) nhận (2;-1) là 1 vtpt nên nhận \(\overrightarrow{u_2}=\left(1;2\right)\) là 1 vtcp
\(\Rightarrow cos\widehat{\left(d_1;d_2\right)}=\left|cos\widehat{\left(\overrightarrow{u_1};\overrightarrow{u_2}\right)}\right|=\dfrac{\left|\overrightarrow{u_1}.\overrightarrow{u_2}\right|}{\left|\overrightarrow{u_1}\right|.\left|\overrightarrow{u_2}\right|}=\dfrac{\left|3.1+1.2\right|}{\sqrt{3^2+1^2}.\sqrt{1^2+2^2}}=\dfrac{\sqrt{2}}{2}\)
\(\Rightarrow\widehat{\left(d_1;d_2\right)}=45^0\)