Cho hàm số \(y=x^2+bx+1\) với \(b\in\left(3;\dfrac{7}{2}\right)\)
Giải bất phương trình \(f\left(f\left(x\right)\right)>x\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
17 tháng 1 2021
f(0) = 1
\(\Rightarrow\) a.02 + b.0 + c = 1
\(\Rightarrow\) c = 1
Vậy hệ số a = 0; b = 0; c = 1
f(1) = 2
\(\Rightarrow\) a.12 + b.1 + c = 2
\(\Rightarrow\) a + b + c = 2
Vậy hệ số a = 1; b = 1; c = 1
f(2) = 4
\(\Rightarrow\) a.22 + b.2 + c = 4
\(\Rightarrow\) 4a + 2b + c = 4
Vậy hệ số a = 4; b = 2; c = 1
Chúc bn học tốt! (chắc vậy :D)
NV
Nguyễn Việt Lâm
Giáo viên
16 tháng 4 2022
\(\lim\limits_{x\rightarrow0}\dfrac{\sqrt[3]{ax+1}-\sqrt[]{1-bx}}{x}=\lim\limits_{x\rightarrow0}\dfrac{\dfrac{ax}{\sqrt[3]{\left(ax+1\right)^2}+\sqrt[3]{ax+1}+1}+\dfrac{bx}{1+\sqrt[]{1-bx}}}{x}\)
\(=\lim\limits_{x\rightarrow0}\left(\dfrac{a}{\sqrt[3]{\left(ax+1\right)^2}+\sqrt[3]{ax+1}+1}+\dfrac{b}{1+\sqrt[]{1-bx}}\right)=\dfrac{a}{3}+\dfrac{b}{2}\)
Hàm liên tục tại \(x=0\) khi:
\(\dfrac{a}{3}+\dfrac{b}{2}=3a-5b-1\Leftrightarrow8a-11b=3\)
20 tháng 1
a: f(1)=1
=>\(a\cdot1^2+b\cdot1+1=1\)
=>a+b=0
f(-1)=3
=>\(a\cdot\left(-1\right)^2+b\cdot\left(-1\right)+1=3\)
=>a-b=2
mà a+b=0
nên \(a=\dfrac{2+0}{2}=1;b=2-1=1\)
b: a=1 và b=1 nên \(f\left(x\right)=x^2+x+1\)
\(\Leftrightarrow\dfrac{n}{f\left(n\right)}=\dfrac{n}{n^2+n+1}\)
Gọi d=ƯCLN(n^2+n+1;n)
=>\(\left\{{}\begin{matrix}n^2+n+1⋮d\\n⋮d\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}n^2+n+1⋮d\\n\left(n+1\right)⋮d\end{matrix}\right.\)
=>\(\left(n^2+n+1\right)-n\left(n+1\right)⋮d\)
=>\(1⋮d\)
=>d=1
=>ƯCLN(n^2+n+1;n)=1
=>\(\dfrac{n}{f\left(n\right)}=\dfrac{n}{n^2+n+1}\) là phân số tối giản
HQ
Hà Quang Minh
Giáo viên
24 tháng 8 2023
Ta có: \(P'\left(x\right)=2ax+b\Rightarrow P''\left(x\right)=2a\\ \Rightarrow\left\{{}\begin{matrix}P'\left(1\right)=2a+b=0\\P''\left(1\right)=2a=-2\end{matrix}\right.\\ \Rightarrow\left\{{}\begin{matrix}a=-1\\b=2\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
29 tháng 6 2021
\(f'\left(x\right)=0\) có 3 nghiệm \(x=-1;0;2\)
Dấu của \(f'\left(x\right)\) trên trục số:
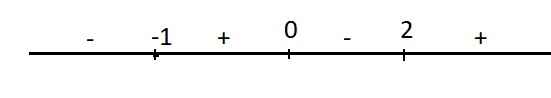
Ta thấy có 2 lần \(f'\left(x\right)\) đổi dấu từ âm sang dương nên hàm có 2 cực tiểu
17 tháng 3 2023
1: Theo đề, ta có:
-b/2*(-1)=5/2
=>-b/-2=5/2
=>b=5
2: y=-x^2+5x-4
