giải phương trình: \(4x^2-5x+6\sqrt{x}-8=0\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


2:
a: =>2x^2-4x-2=x^2-x-2
=>x^2-3x=0
=>x=0(loại) hoặc x=3
b: =>(x+1)(x+4)<0
=>-4<x<-1
d: =>x^2-2x-7=-x^2+6x-4
=>2x^2-8x-3=0
=>\(x=\dfrac{4\pm\sqrt{22}}{2}\)

\(a,PT\Leftrightarrow\left|x+3\right|=3x-6\\ \Leftrightarrow\left[{}\begin{matrix}x+3=3x-6\left(x\ge-3\right)\\x+3=6-3x\left(x< -3\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{9}{2}\left(tm\right)\\x=\dfrac{3}{4}\left(ktm\right)\end{matrix}\right.\\ \Leftrightarrow x=\dfrac{9}{2}\\ b,PT\Leftrightarrow\left|x-1\right|=\left|2x-1\right|\\ \Leftrightarrow\left[{}\begin{matrix}x-1=2x-1\\1-x=2x-1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{2}{3}\end{matrix}\right.\)
\(c,ĐK:x\le\dfrac{2}{5}\\ PT\Leftrightarrow4-5x=25x^2-20x+4\\ \Leftrightarrow25x^2-15x=0\\ \Leftrightarrow5x\left(5x-3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\left(tm\right)\\x=\dfrac{3}{5}\left(ktm\right)\end{matrix}\right.\Leftrightarrow x=0\\ d,ĐK:x\le\dfrac{2}{5}\\ PT\Leftrightarrow4-5x=2-5x\\ \Leftrightarrow x\in\varnothing\)

a: Ta có: \(\sqrt{4x^2+4x+3}=8\)
\(\Leftrightarrow4x^2+4x+1+2-64=0\)
\(\Leftrightarrow4x^2+4x-61=0\)
\(\Delta=4^2-4\cdot4\cdot\left(-61\right)=992\)
Vì Δ>0 nên phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x_1=\dfrac{-4-4\sqrt{62}}{8}=\dfrac{-1-\sqrt{62}}{2}\\x_2=\dfrac{-4+4\sqrt{62}}{8}=\dfrac{-1+\sqrt{62}}{2}\end{matrix}\right.\)

a: \(\Leftrightarrow\dfrac{1}{3}\sqrt{x-2}-\dfrac{2}{3}\cdot3\sqrt{x-2}+6\cdot\dfrac{\sqrt{x-2}}{9}=-4\)
\(\Leftrightarrow\sqrt{x-2}=4\)
=>x-2=16
hay x=18
b: \(\Leftrightarrow\left|3x+2\right|=4x\)
\(\Leftrightarrow\left[{}\begin{matrix}3x+2=4x\left(x>=-\dfrac{2}{3}\right)\\3x+2=-4x\left(x< -\dfrac{2}{3}\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\left(nhận\right)\\x=-\dfrac{2}{7}\left(nhận\right)\end{matrix}\right.\)
c: \(\Leftrightarrow3\sqrt{x-2}-2\sqrt{x-2}+3\sqrt{x-2}=40\)
\(\Leftrightarrow4\sqrt{x-2}=40\)
=>x-2=100
hay x=102
d: =>5x-6=9
hay x=3
\(\dfrac{1}{3}\sqrt{x-2}-\dfrac{2}{3}\sqrt{9x-18}+6\sqrt{\dfrac{x-2}{81}}=-4\) (đk: x≥2)
\(\dfrac{1}{3}\sqrt{x-2}-\dfrac{2}{3}\sqrt{9\left(x-2\right)}+6\sqrt{\dfrac{1}{81}\left(x-2\right)}=-4\)
\(\dfrac{1}{3}\sqrt{x-2}-2\sqrt{x-2}+\dfrac{2}{3}\sqrt{x-2}=-4\)
\(\dfrac{1}{3}\sqrt{x-2}-\dfrac{4}{3}\sqrt{x-2}=-4\)
\(-\sqrt{x-2}=-4\)
\(\sqrt{x-2}=4\)
\(\left|x-2\right|=16\)
\(\Leftrightarrow\left[{}\begin{matrix}x-2=16\\x-2=-16\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=18\left(TM\right)\\x=-14\left(L\right)\end{matrix}\right.\)

`a, <=> 5/3 . 3sqrt(x^2+2) + 3/2.2sqrt(x^2+2)-7sqrt6=sqrt(x^2+2)`
`= (5+3-1)sqrt(x^2+2)=7sqrt6`
`<=> 7sqrt(x^2+2)=7sqrt6`.
`<=> x^2+2=36`.
`<=> x^2=34`.
`<=> x=+-sqrt(34)`.
Vậy...
`b, sqrt(4x^2-12x+9)-6=0`
`<=> |2x-3|=6`.
`@ x >=3/2 <=> 2x-3=6.`
`<=> x=9/2 (tm)`.
`@x <3/2 <=> 3-2x=6`
`<=> 2x=-3`
`<=> x=-3/2.`
Vậy...

a: \(\Leftrightarrow\left(x-\sqrt{5}\right)^2=0\)
\(\Leftrightarrow x-\sqrt{5}=0\)
hay \(x=\sqrt{5}\)
b: \(\Leftrightarrow4x^4-9x^2+4x^2-9=0\)
\(\Leftrightarrow4x^2-9=0\)
=>x=3/2hoặc x=-3/2
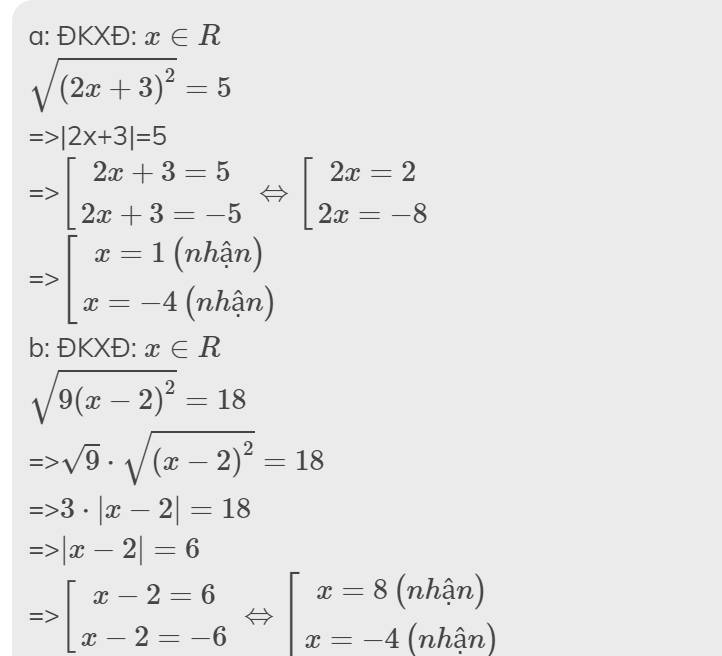
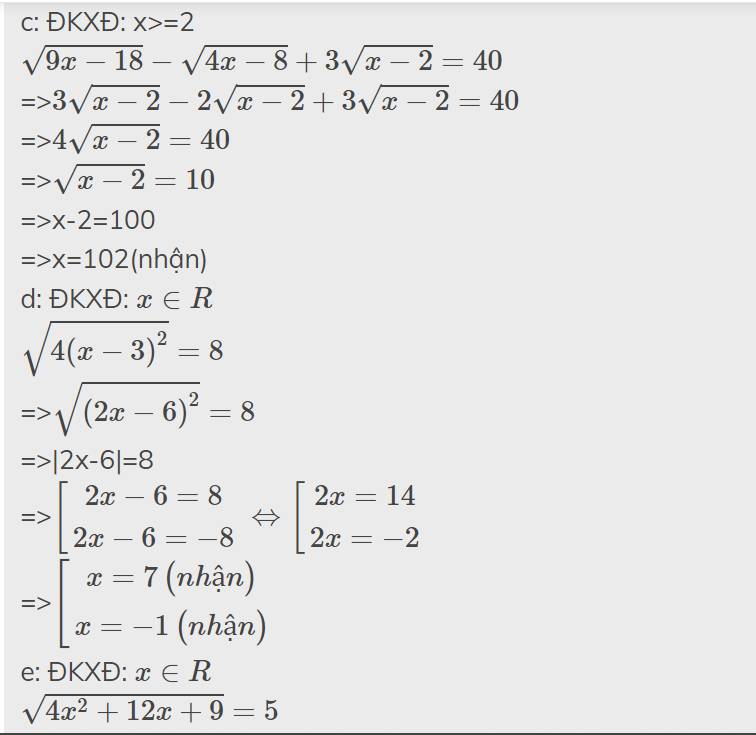
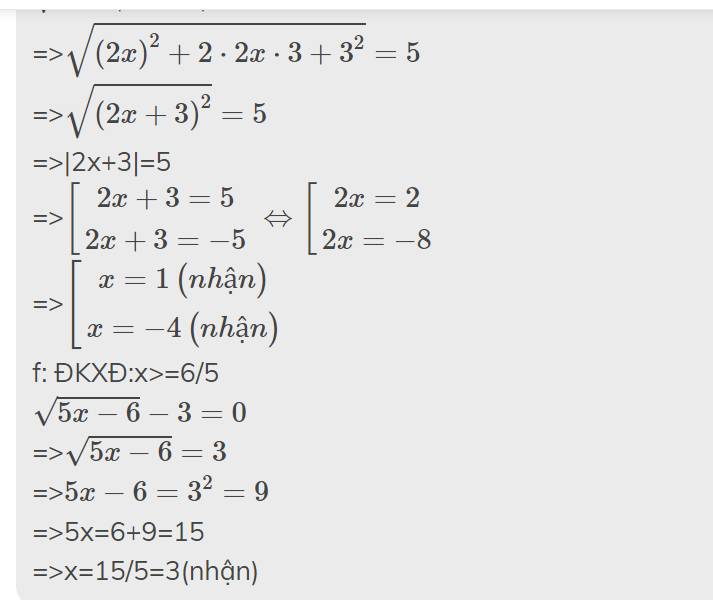
Lớp 9 đã học giải phương trình bậc 3 chưa nhỉ ?
\(4x^2-5x+6\sqrt{x}-8=0\)
\(< =>\left(4x^2-5x+6\sqrt{x}-8\right)x=0.x\)
\(< =>4x^3-5x^2-2x=0\)(đến đây giải pt bậc 3 hoặc làm theo mình)
\(< =>x\left(4x^2-5x-2\right)=0\)
\(< =>\orbr{\begin{cases}x=0\\4x^2-5x-2=0\left(1\right)\end{cases}}\)
Từ 1 ta có \(\Delta=\left(-5\right)^2-4.4.\left(-2\right)=25+32=57\)
Nên phương trình (1) có 2 nghiệm phân biệt
\(x_1=\frac{5+\sqrt{57}}{8}\)
\(x_2=\frac{5-\sqrt{57}}{8}\)
Vậy tập nghiệm của phương trình trên là \(\left\{0;\frac{5+\sqrt{57}}{8};\frac{5-\sqrt{57}}{8}\right\}\)
ĐK:..
Đặt: \(\sqrt{x}=t\ge0\) ta có phương trình ẩn t :
\(4t^4-5t^2+6t-8=0\)
<=> \(4t^4-\left(t^2-4t+4\right)-4t^2+2t-4=0\)
<=> \(\left(2t^2\right)^2-\left(t-2\right)^2-2\left(2t^2-t+2\right)=0\)
<=> \(\left(2t^2-t+2\right)\left(2t^2+t-2\right)-2\left(2t^2-t+2\right)=0\)
<=> \(\left(2t^2+t-4\right)\left(2t^2-t+2\right)\)= 0
<=> \(\orbr{\begin{cases}2t^2+t-4=0\\2t^2-t+2=0\left(vn\right)\end{cases}}\)
<=> \(\orbr{\begin{cases}t=\frac{-1+\sqrt{33}}{4}\\t=\frac{-1-\sqrt{33}}{4}< 0\left(loai\right)\end{cases}}\)
Khi đó: \(\sqrt{x}=\frac{-1+\sqrt{33}}{4}\Leftrightarrow x=\frac{17-\sqrt{33}}{8}\)tm
Vậy:...