cho a,b,c≥0 chứng minh a+b+c≥\(\sqrt{ab}\)+\(\sqrt{ac}\)+\(\sqrt{bc}\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
13 tháng 5 2020
\(\sqrt{a+bc}=\sqrt{a\left(a+b+c\right)+bc}=\sqrt{\left(a+b\right)\left(a+c\right)}\ge\sqrt{\left(a+\sqrt{bc}\right)^2}=a+\sqrt{bc}\)
Tương tự: \(\sqrt{b+ac}\ge b+\sqrt{ac}\) ; \(\sqrt{c+ab}\ge c+\sqrt{ab}\)
\(\Rightarrow VT\ge a+b+c+\sqrt{ab}+\sqrt{bc}+\sqrt{ca}-\sqrt{ab}-\sqrt{bc}-\sqrt{ca}\)
\(\Rightarrow VT\ge a+b+c=1\)
Dấu "=" xảy ra khi \(a=b=c=\frac{1}{3}\)
25 tháng 1 2020
1) \(\Sigma\frac{a}{b^3+ab}=\Sigma\left(\frac{1}{b}-\frac{b}{a+b^2}\right)\ge\Sigma\frac{1}{a}-\Sigma\frac{1}{2\sqrt{a}}=\Sigma\left(\frac{1}{a}-\frac{2}{\sqrt{a}}+1\right)+\Sigma\frac{3}{2\sqrt{a}}-3\)
\(\ge\Sigma\left(\frac{1}{\sqrt{a}}-1\right)^2+\frac{27}{2\left(\sqrt{a}+\sqrt{b}+\sqrt{c}\right)}-3\ge\frac{27}{2\sqrt{3\left(a+b+c\right)}}-3=\frac{3}{2}\)
6 tháng 3 2021
\(\dfrac{a}{\sqrt{a^2+1}}=\dfrac{a}{\sqrt{a^2+ab+ac+bc}}=\dfrac{a}{\sqrt{\left(a+b\right)\left(a+c\right)}}\le\dfrac{a}{2}\left(\dfrac{1}{a+b}+\dfrac{1}{a+c}\right)=\dfrac{1}{2}\left(\dfrac{a}{a+b}+\dfrac{a}{a+c}\right)\) Chứng minh tương tự ta được:
\(\dfrac{b}{\sqrt{b^2+1}}\le\dfrac{1}{2}\left(\dfrac{b}{b+a}+\dfrac{b}{b+c}\right);\dfrac{c}{\sqrt{c^2+1}}\le\dfrac{1}{2}\left(\dfrac{c}{c+a}+\dfrac{c}{c+b}\right)\)
\(\Rightarrow\dfrac{a}{\sqrt{a^2+1}}+\dfrac{b}{\sqrt{b^2+1}}+\dfrac{c}{\sqrt{c^2+1}}\le\dfrac{1}{2}\left(\dfrac{a}{a+b}+\dfrac{a}{a+c}+\dfrac{b}{b+a}+\dfrac{b}{b+c}+\dfrac{c}{c+a}+\dfrac{c}{c+b}\right)=\dfrac{1}{2}\left(\dfrac{a+b}{a+b}+\dfrac{b+c}{b+c}+\dfrac{c+a}{c+a}\right)=\dfrac{1}{2}\left(1+1+1\right)=\dfrac{3}{2}\) Dấu = xảy ra \(\Leftrightarrow a=b=c=\dfrac{1}{\sqrt{3}}\)
NV
Nguyễn Việt Lâm
Giáo viên
6 tháng 3 2021
\(\dfrac{a}{\sqrt{a^2+1}}=\dfrac{a}{\sqrt{a^2+ab+bc+ca}}=\dfrac{a}{\sqrt{\left(a+b\right)\left(a+c\right)}}\le\dfrac{1}{2}\left(\dfrac{a}{a+b}+\dfrac{a}{a+c}\right)\)
Tương tự: \(\dfrac{b}{\sqrt{b^2+1}}\le\dfrac{1}{2}\left(\dfrac{b}{a+b}+\dfrac{b}{b+c}\right)\) ; \(\dfrac{c}{\sqrt{c^2+1}}\le\dfrac{1}{2}\left(\dfrac{c}{c+a}+\dfrac{c}{b+c}\right)\)
Cộng vế:
\(VT\le\dfrac{1}{2}\left(\dfrac{a}{a+b}+\dfrac{b}{a+b}+\dfrac{a}{a+c}+\dfrac{c}{a+c}+\dfrac{b}{b+c}+\dfrac{c}{b+c}\right)=\dfrac{3}{2}\)
Dấu "=" xảy ra khi \(a=b=c=\dfrac{1}{\sqrt{3}}\)
KN
4 tháng 6 2020
Theo giả thiết thì \(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}=1\Rightarrow ab+bc+ca=abc\)
Ta cần chứng minh: \(\Sigma\sqrt{a+bc}\ge\sqrt{abc}+\Sigma\sqrt{a}\)(*)
Thật vậy: (*) \(\Leftrightarrow\Sigma\sqrt{\frac{a^2+abc}{a}}\ge\sqrt{abc}+\Sigma\sqrt{a}\)
\(\Leftrightarrow\Sigma\sqrt{\frac{a^2+ab+bc+ca}{a}}\ge\sqrt{abc}+\Sigma\sqrt{a}\)\(\Leftrightarrow\Sigma\sqrt{\frac{\left(a+b\right)\left(a+c\right)}{a}}\ge\sqrt{abc}+\Sigma\sqrt{a}\)
\(\Leftrightarrow\text{}\Sigma\sqrt{bc\left(a+b\right)\left(a+c\right)}\ge abc+\sqrt{abc}\left(\Sigma\sqrt{a}\right)\)(Nhân cả hai vế của bất đẳng thức với \(\sqrt{abc}>0\))
\(\Leftrightarrow\Sigma\sqrt{\left(b^2+ab\right)\left(c^2+ac\right)}\ge abc+\Sigma a\sqrt{bc}\)
Bất đẳng thức cuối luôn đúng vì theo BĐT Cauchy-Schwarz, ta có: \(\Sigma\sqrt{\left(b^2+ab\right)\left(c^2+ac\right)}\ge\Sigma\left(bc+a\sqrt{bc}\right)=abc+\Sigma a\sqrt{bc}\text{}\)
Đẳng thức xảy ra khi a = b = c = 3
16 tháng 7 2018
Đặt VT= \(\sqrt{a+bc}+\sqrt{b+ac}+\sqrt{c+ab}\)
Áp dụng bất đẳng thức Bu-nhi-a-cốp-xki ta có:
\(VT^2=\left(\sqrt{a+bc}+\sqrt{b+ac}+\sqrt{c+ab}\right)^2\le\)
\(\le\left(1^2+1^2+1^2\right)\left(a+b+c+ab+bc+ca\right)\)
Lại có \(ab+bc+ca\le\dfrac{1}{3}\left(a+b+c\right)^2\)( tự cm nhé)
Từ đó \(VT^2\le3.\left(1+\dfrac{1}{3}\right)=4\) (do a+b+c=1)
Dấu "=" xảy ra \(\Leftrightarrow a=b=c=\dfrac{1}{3}\)
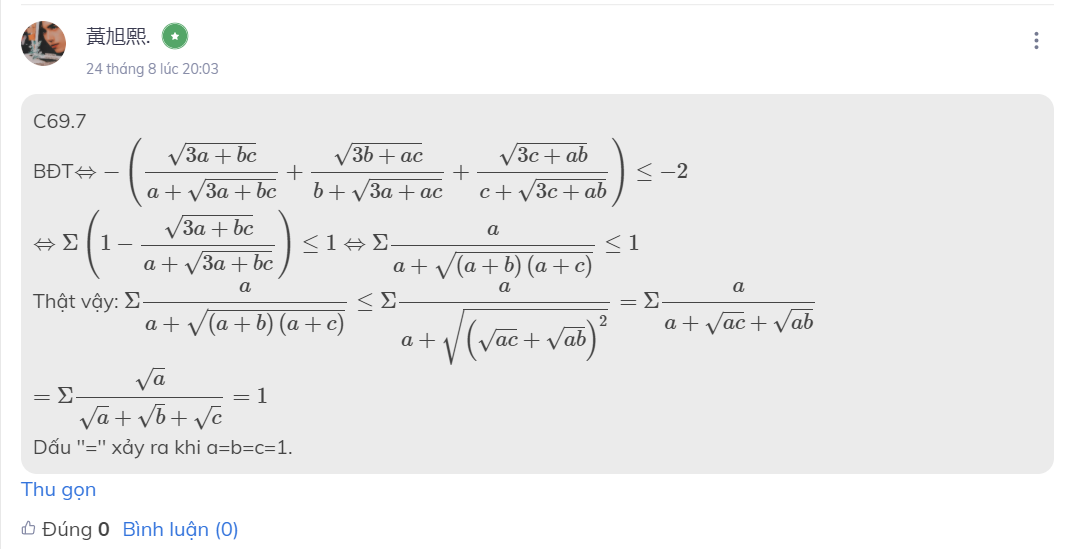
Biến đổi tương đương:
\(2a+2b+2c\ge2\sqrt{ab}+2\sqrt{bc}+2\sqrt{ca}\)
\(\Leftrightarrow a-2\sqrt{ab}+b+b-2\sqrt{bc}+c+c-2\sqrt{ca}+a\ge0\)
\(\Leftrightarrow\left(\sqrt{a}-\sqrt{b}\right)^2+\left(\sqrt{b}-\sqrt{c}\right)^2+\left(\sqrt{c}-\sqrt{a}\right)^2\ge0\) (luôn đúng)
Dấu "=" xảy ra khi \(a=b=c\)