 giúp mình vs mình đg cần gấp
giúp mình vs mình đg cần gấp
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Cơ chế hình thành cây có kiểu gen Aaa là do rồi loạn giảm phân, diễn ra ở kì sau của giảm phân 1.
Sơ đồ lai:
P: Aa x Aa
GP: Aa ; 0 ; A ; a
F1: Aaa ; a


Bài 8:
a: Ta có: \(\left(5x+1\right)^2=\dfrac{36}{49}\)
\(\Leftrightarrow\left[{}\begin{matrix}5x+1=\dfrac{6}{7}\\5x+1=-\dfrac{6}{7}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}5x=\dfrac{-1}{7}\\5x=\dfrac{-13}{7}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{-1}{35}\\x=\dfrac{-13}{35}\end{matrix}\right.\)
b: Ta có: \(\left(x-\dfrac{2}{9}\right)^3=\left(\dfrac{2}{3}\right)^6\)
\(\Leftrightarrow x-\dfrac{2}{9}=\dfrac{4}{9}\)
hay \(x=\dfrac{2}{3}\)
\(7,\\ a,=\dfrac{3^{10}\cdot3^5\cdot5^5}{5^6\cdot\left[-\left(3^7\right)\right]}=\dfrac{3^8}{-5}=-\dfrac{6561}{5}\\ b,=8+3-\dfrac{1}{4}\cdot4+\left(4:\dfrac{1}{2}\right)\cdot8\\ =8+3-1+64=74\\ 8,\\ a,\left(5x+1\right)^2=\dfrac{36}{49}\Rightarrow\left[{}\begin{matrix}5x+1=\dfrac{6}{7}\\5x+1=-\dfrac{6}{7}\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=-\dfrac{1}{35}\\x=-\dfrac{13}{35}\end{matrix}\right.\)
\(b,\Rightarrow8x-1=5\Rightarrow x=\dfrac{3}{4}\\ d,\Rightarrow\left\{{}\begin{matrix}x-3,5=0\\y-\dfrac{1}{10}=0\end{matrix}\right.\left[\left(x-3,5\right)^2\ge0;\left(y-\dfrac{1}{10}\right)^4\ge0\right]\\ \Rightarrow\left\{{}\begin{matrix}x=3,5\\y=\dfrac{1}{10}\end{matrix}\right.\)

a: góc DAC=90-40=50 độ
b: góc ADB=90 độ
c: góc DAB=90-80=10 độ
=>góc BAE=10+50=60 độ
góc AED=180-60=120 độ

14.He asked me to help my withe the exercises.
15.He said he left early on Friday.
16.She asked me where he would meet that night.
17.Milk could be used for making butter and cheese.
18.Bottles of milk are brought to houses by the milkman.
19.A lot of beautiful toys are made from recyced plastic.
20.The concerts usually are held at the university.


Câu 1:C
Câu 2: A
Câu 3: A
Câu 4: C
Câu 5: C
Câu 6: D
Câu 7: D
Câu 8: A
Câu 10: C




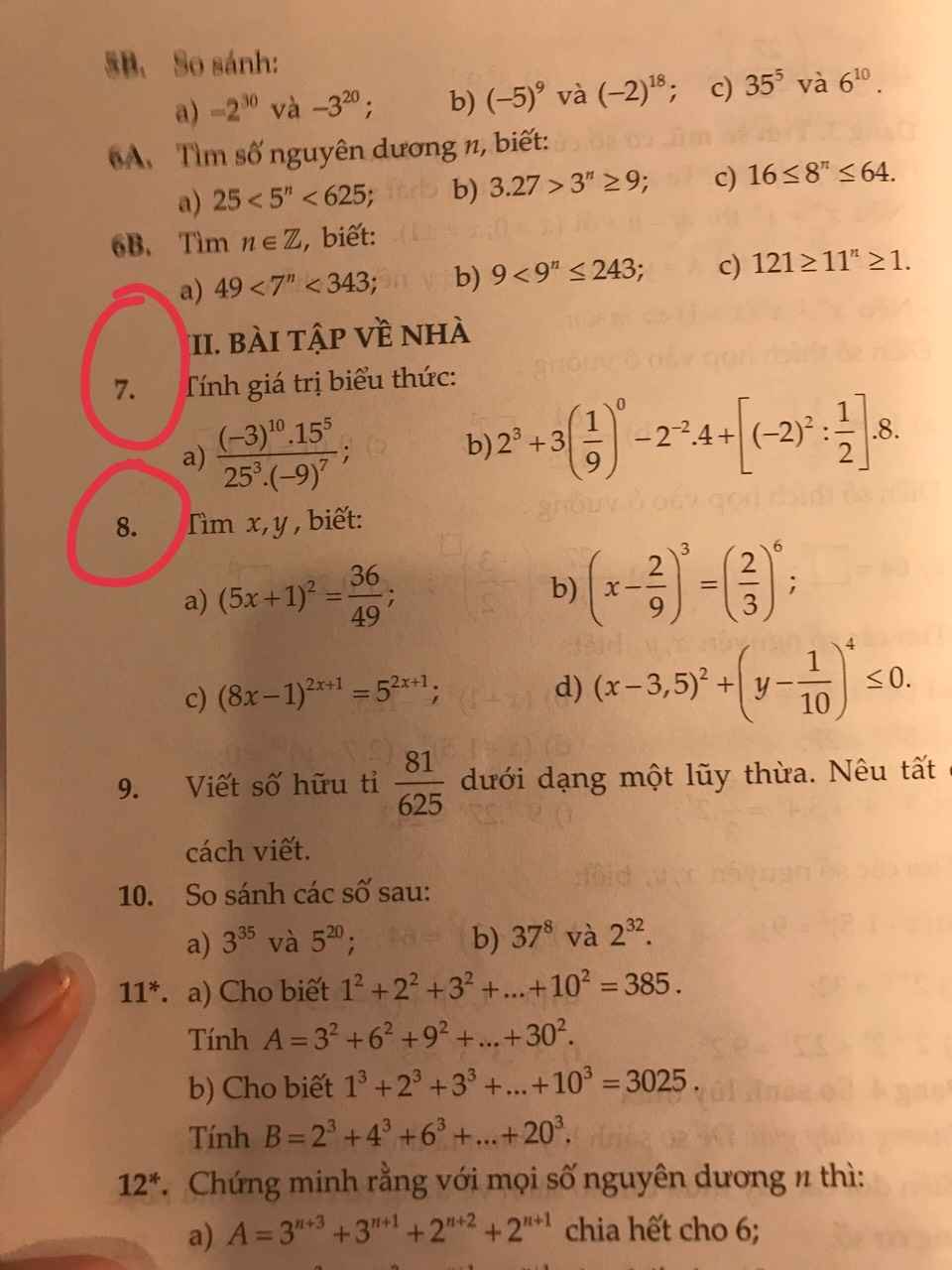



 giúp mình vs mik đg cần gấp
giúp mình vs mik đg cần gấp
a) Ta có: \(Q=\dfrac{3x+\sqrt{9x}-3}{x+\sqrt{x}-2}-\dfrac{\sqrt{x}+1}{\sqrt{x}+2}+\dfrac{\sqrt{x}-2}{1-\sqrt{x}}\)
\(=\dfrac{3x+3\sqrt{x}-3-\left(x-1\right)-\left(x-4\right)}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{3x+3\sqrt{x}-3-x+1-x+4}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{x+3\sqrt{x}+2}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{\sqrt{x}+1}{\sqrt{x}-1}\)
b) Thay \(x=4+2\sqrt{3}\) vào Q, ta được:
\(Q=\dfrac{\sqrt{3}+1+1}{\sqrt{3}+1-1}=\dfrac{2+\sqrt{3}}{\sqrt{3}}=\dfrac{2\sqrt{3}+3}{3}\)
c) Để Q=3 thì \(\sqrt{x}+1=3\sqrt{x}-3\)
\(\Leftrightarrow\sqrt{x}-3\sqrt{x}=-3-1\)
\(\Leftrightarrow2\sqrt{x}=4\)
hay x=4
d) Để \(Q>\dfrac{1}{2}\) thì \(\dfrac{\sqrt{x}+1}{\sqrt{x}-1}-\dfrac{1}{2}>0\)
\(\Leftrightarrow\dfrac{2\sqrt{x}+2-\sqrt{x}+1}{2\left(\sqrt{x}-1\right)}>0\)
\(\Leftrightarrow\sqrt{x}-1>0\)
\(\Leftrightarrow x>1\)
Kết hợp ĐKXĐ, ta được: x>1
e) Để Q nguyên thì \(\sqrt{x}+1⋮\sqrt{x}-1\)
\(\Leftrightarrow2⋮\sqrt{x}-1\)
\(\Leftrightarrow\sqrt{x}-1\in\left\{-1;1;2\right\}\)
\(\Leftrightarrow\sqrt{x}\in\left\{0;2;3\right\}\)
hay \(x\in\left\{0;4;9\right\}\)