ND
3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NN
4
11 tháng 9 2021
\(y=\sin^4x+\cos^4x\\ =1-2\sin^2x\cdot\cos^2x\\ =1-\dfrac{1}{2}\sin^22x\\ 0\le\sin^22x\le1\\ \Leftrightarrow\dfrac{1}{2}\le y\le1\\ y_{min}=\dfrac{1}{2}\Leftrightarrow\sin^22x=1\Leftrightarrow x=\dfrac{k\pi}{2}\pm\dfrac{\pi}{4}\\ y_{max}=1\Leftrightarrow\sin^22x=0\Leftrightarrow x=k\pi\)
11 tháng 9 2021
\(y=3\sin x+4\cos x\\ =5\left(\dfrac{3\sin x}{5}+\dfrac{4\cos x}{5}\right)\\ =5\cos\left(x-a\right),\forall\cos a=\dfrac{4}{5},\sin a=\dfrac{3}{5}\\ -1\le\cos\left(x-a\right)\le1\\ \Leftrightarrow-5\le y\le5\\ y_{min}=-5\Leftrightarrow\cos\left(x-a\right)=-1\\ y_{max}=5\Leftrightarrow\cos\left(x-a\right)=1\)
L
14 tháng 7 2021
Em không thấy đáp án giống như trên lời giải, có thể giúp em làm cách khác không ạ?
NV
Nguyễn Việt Lâm
Giáo viên
14 tháng 7 2021
Bạn Phúc hơi nhầm 1 xíu
\(y=4sinx\left(\dfrac{1}{2}cosx-\dfrac{\sqrt{3}}{2}sinx\right)=2sinx.cosx-2\sqrt{3}sin^2x\)
\(=sin2x-\sqrt{3}\left(1-cos2x\right)=sin2x+\sqrt{3}cos2x-\sqrt{3}\)
\(=2\left(\dfrac{\sqrt{3}}{2}cos2x+\dfrac{1}{2}sin2x\right)-\sqrt{3}\)
\(=2cos\left(2x-\dfrac{\pi}{6}\right)-\sqrt{3}\)
\(\Rightarrow y_{min}=-2-\sqrt{3}\) ; \(y_{max}=2-\sqrt{3}\)
Đáp án mà đề đưa ra như bên dưới đều sai cả.
TL
0
PA
1
NV
Nguyễn Việt Lâm
Giáo viên
20 tháng 7 2021
a.
Đặt \(cos2x=t\Rightarrow t\in\left[-1;1\right]\)
Xét hàm \(y=f\left(t\right)=2t^2+2t-4\) trên \(\left[-1;1\right]\)
\(-\dfrac{b}{2a}=-\dfrac{1}{2}\in\left[-1;1\right]\)
\(f\left(-1\right)=-4\) ; \(f\left(-\dfrac{1}{2}\right)=-\dfrac{9}{2}\) ; \(f\left(1\right)=0\)
\(\Rightarrow y_{min}=-\dfrac{9}{2}\) khi \(t=-\dfrac{1}{2}\) hay \(cos2x=-\dfrac{1}{2}\)
\(y_{max}=0\) khi \(cos2x=1\)
b. Đặt \(tanx=t\Rightarrow t\in\left[-1;\sqrt{3}\right]\)
Xét hàm \(f\left(t\right)=t^2-2\sqrt{3}t-1\) trên \(\left[-1;\sqrt{3}\right]\)
\(-\dfrac{b}{2a}=\sqrt{3}\in\left[-1;\sqrt{3}\right]\)
\(f\left(-1\right)=2\sqrt{3}\) ; \(f\left(\sqrt{3}\right)=-4\)
\(y_{min}=-4\) khi \(x=\dfrac{\pi}{3}\) ; \(y_{max}=2\sqrt{3}\) khi \(x=-\dfrac{\pi}{4}\)
NV
Nguyễn Việt Lâm
Giáo viên
22 tháng 12 2020
\(\Leftrightarrow1-2sin^2x+\left(2m-3\right)sinx+m-2=0\)
\(\Leftrightarrow2sin^2x-\left(2m-3\right)sinx-m+1=0\)
\(\Leftrightarrow2sin^2x+sinx-2\left(m-1\right)sinx-\left(m-1\right)=0\)
\(\Leftrightarrow sinx\left(2sinx+1\right)-\left(m-1\right)\left(2sinx+1\right)=0\)
\(\Leftrightarrow\left(2sinx+1\right)\left(sinx-m+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=-\dfrac{1}{2}\\sinx=m-1\end{matrix}\right.\)
Pt có đúng 2 nghiệm thuộc khoảng đã cho khi và chỉ khi:
\(\left\{{}\begin{matrix}m-1\ne-\dfrac{1}{2}\\-1\le m-1\le1\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}m\ne\dfrac{1}{2}\\0\le m\le2\end{matrix}\right.\)
BC
1
3 tháng 12 2023
Để \(cos\left(x-\dfrac{\Omega}{4}\right);sinx;cos\left(x+\dfrac{\Omega}{4}\right)\) là ba số hạng liên tiếp của cấp số nhân thì \(sin^2x=cos\left(x-\dfrac{\Omega}{4}\right)\cdot cos\left(x+\dfrac{\Omega}{4}\right)\)
=>\(sin^2x=\sqrt{2}\left(cosx-sinx\right)\cdot\sqrt{2}\left(cosx+sinx\right)\)
=>\(sin^2x=2cos^2x-2sin^2x\)
=>\(3\cdot sin^2x=2\cdot cos^2x\)
=>\(\dfrac{sin^2x}{cos^2x}=\dfrac{2}{3}\)
=>\(tan^2x=\dfrac{2}{3}\)
=>\(\left[{}\begin{matrix}tanx=\dfrac{\sqrt{6}}{3}\\tanx=-\dfrac{\sqrt{6}}{3}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=arctan\left(\dfrac{\sqrt{6}}{3}\right)+k\Omega\\x=arctan\left(-\dfrac{\sqrt{6}}{3}\right)+k\Omega\end{matrix}\right.\)
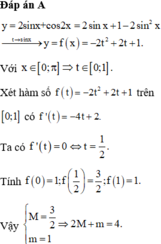
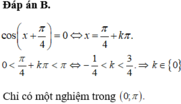
\(0\le x\le\pi\Rightarrow\dfrac{\pi}{3}\le x+\dfrac{\pi}{3}\le\dfrac{4\pi}{3}\)
\(\Rightarrow y_{max}=\dfrac{1}{2}\) khi \(x+\dfrac{\pi}{3}=\dfrac{\pi}{3}\Leftrightarrow x=0\)
\(y_{min}=-1\) khi \(x+\dfrac{\pi}{3}=\pi\Leftrightarrow x=\dfrac{2\pi}{3}\)
ry5yrerwwkewwwdwjwkjdjwkwe