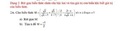ĐK : x >= 0 ; x khác 1

\(=\frac{\sqrt{x}\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}+\frac{3\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}-\frac{6\sqrt{x}-4}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)

\(=\frac{x+\sqrt{x}+3\sqrt{x}-3-6\sqrt{x}+4}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\frac{x-2\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\frac{\left(\sqrt{x}-1\right)^2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\frac{\sqrt{x}-1}{\sqrt{x}+1}\)

Để P = -1 thì \(\frac{\sqrt{x}-1}{\sqrt{x}+1}=-1\Rightarrow\sqrt{x}-1=-\sqrt{x}-1\Leftrightarrow2\sqrt{x}=0\Leftrightarrow x=0\left(tm\right)\)

em cảm ơn ạ

\(\frac{x+12}{x-4}+\frac{1}{\sqrt{x}+2}=\frac{x+12+\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\frac{\sqrt{x}+x+10}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\)

=\(\frac{\sqrt{x}+x+10}{x-4}\) nhé :/

\(đk:x\ge\frac{5}{3}\)

\(\sqrt{x^2-x}=\sqrt{3x-5}\)

\(\Leftrightarrow x^2+x=3x-5\)

\(\Leftrightarrow x^2-2x+5=0\)

\(\Leftrightarrow\left(x-1\right)^2+4=0\)

\(\Leftrightarrow\left(x-1\right)^2=-4\left(voli\right)\)

vậy pt vô nghiệm

a,  \(14\sqrt{\frac{1}{7}}-\frac{3}{2}\sqrt{28}\)\(+20\sqrt{0,63}\)

\(=2\sqrt{7}-3\sqrt{7}+6\sqrt{7}\)

\(=5\sqrt{7}\)

b,  \(\sqrt{\frac{a}{2}}+\frac{4}{5}\sqrt{8\text{a}}-\sqrt{\frac{2\text{a}}{9}}v\text{ới}a\ge0\)

\(=\sqrt{\frac{2\text{a}}{4\text{ }}}+\frac{8}{5}\sqrt{2\text{a}}-\frac{1}{3}\sqrt{2\text{a}}\)

\(=\frac{1}{2}\sqrt{2\text{a}}+\frac{8}{5}\sqrt{2\text{a}}-\frac{1}{3}\sqrt{2\text{a}}\)

\(=\frac{53}{30}\sqrt{2\text{a}}\)

Bạn kiểm tra lại điều kiện của đề bài nhé vì x ở dưới mẫu ko thể = 0 được

a) M = \(\left(\frac{\sqrt{x}}{\sqrt{x}-1}+\frac{\sqrt{x}}{x-1}\right)\): \(\left(\frac{2}{x}-\frac{2-x}{x\sqrt{x}+x}\right)\)  ( ĐKXĐ : x > 0 , x\(\ne\)1)

M = \(\frac{\sqrt{x}\left(\sqrt{x}+1\right)+\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\):\(\frac{2\left(\sqrt{x}+1\right)-2+x}{x\left(\sqrt{x}+1\right)}\)

M = \(\frac{x+2\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\):\(\frac{x+2\sqrt{x}}{x\left(\sqrt{x}+1\right)}\)

M = \(\frac{\sqrt{x}\left(\sqrt{x}+2\right)}{\left(\sqrt{x-1}\right)\left(\sqrt{x}+1\right)}.\frac{x\left(\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}+2\right)}\)

M = \(\frac{x}{\sqrt{x}-1}\)

b) M = \(\frac{x}{\sqrt{x}-1}\)( ĐKXĐ : x > 0, x\(\ne\)1)

    Ta có : \(\frac{x}{\sqrt{x}-1}\)= \(\frac{-1}{2}\)

\(\Rightarrow\)2x = -1 ( \(\sqrt{x}-1\))

\(\Leftrightarrow\) 2x + \(\sqrt{x}\)-1 = 0

\(\Leftrightarrow\) \(\left(2\sqrt{x}-1\right)\left(\sqrt{x}+1\right)\)= 0

\(\Leftrightarrow\)\(\orbr{\begin{cases}\sqrt{x}=\frac{1}{2}\\\sqrt{x}=-1\end{cases}\Rightarrow}x=\frac{1}{4}\left(TM\text{Đ}K\right)\)

Vậy x =\(\frac{1}{4}\) để M = \(\frac{-1}{2}\)