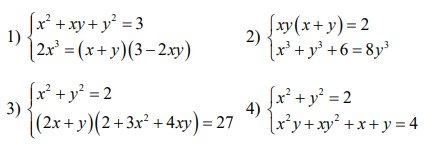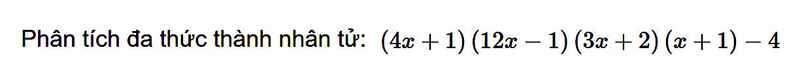cho hình thang abcd vuông tại b và d. cho o là tđ Ac và OA=OC=OD=OB , chứng minh DAC=DBC. mình cần gấp trong tối nay ạ
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 1:
Thay $3=x^2+xy+y^2$ vào PT(2) thì:
$2x^3=(x+y)(x^2+xy+y^2-2xy)$
$\Leftrightarrow 2x^3=(x+y)(x^2-xy+y^2)=x^3+y^3$
$\Leftrightarrow x^3=y^3\Leftrightarrow x=y$.
Thay vào PT(1) thì: $3x^2=3\Leftrightarrow x^2=1\Leftrightarrow x=\pm 1$
$\Rightarrow y=\pm 1$ (tương ứng)
Vậy HPT có nghiệm $(x,y)=(\pm 1, \pm 1)$
Bài 2:
Thay $2=xy(x+y)$ vào PT(2) thì:
$x^3+y^3+3xy(x+y)=8y^3$
$\Leftrightarrow (x+y)^3=(2y)^3$
$\Leftrightarrow x+y=2y\Leftrightarrow x=y$.
Thay vào PT(1): $x^2.2x=2$
$\Leftrightarrow 2x^3=2\Leftrightarrow x^3=1\Leftrightarrow x=1$.
$\Rightarrow y=x=1$
Vậy HPT có nghiệm $(x,y)=(1,1)$

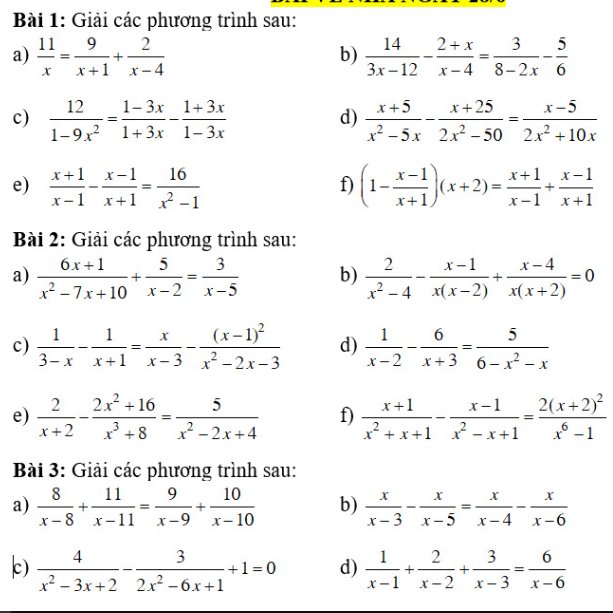
Bài 1:
e: ĐKXĐ: \(x\notin\left\{1;-1\right\}\)
\(\dfrac{x+1}{x-1}-\dfrac{x-1}{x+1}=\dfrac{16}{x^2-1}\)
=>\(\dfrac{\left(x+1\right)^2-\left(x-1\right)^2}{\left(x-1\right)\left(x+1\right)}=\dfrac{16}{\left(x-1\right)\left(x+1\right)}\)
=>\(\left(x+1\right)^2-\left(x-1\right)^2=16\)
=>\(\left(x+1+x-1\right)\left(x+1-x+1\right)=16\)
=>4x=16
=>x=4(nhận)
f: ĐKXĐ: \(x\notin\left\{1-1\right\}\)
\(\left(1-\dfrac{x-1}{x+1}\right)\left(x+2\right)=\dfrac{x+1}{x-1}+\dfrac{x-1}{x+1}\)
=>\(\dfrac{x+1-x+1}{\left(x+1\right)}\left(x+2\right)=\dfrac{\left(x+1\right)^2+\left(x-1\right)^2}{\left(x-1\right)\left(x+1\right)}\)
=>\(\dfrac{2\left(x+2\right)\left(x-1\right)}{\left(x+1\right)\left(x-1\right)}=\dfrac{2x^2+2}{\left(x-1\right)\left(x+1\right)}\)
=>\(2\left(x+2\right)\left(x-1\right)=2\left(x^2+1\right)\)
=>\(\left(x+2\right)\left(x-1\right)=x^2+1\)
=>\(x^2+x-2=x^2+1\)
=>x-2=1
=>x=3(nhận)
a: ĐKXĐ: \(x\notin\left\{0;-1;4\right\}\)
\(\dfrac{11}{x}=\dfrac{9}{x+1}+\dfrac{2}{x-4}\)
=>\(\dfrac{11}{x}=\dfrac{9\left(x-4\right)+2\left(x+1\right)}{\left(x+1\right)\left(x-4\right)}\)
=>\(\dfrac{11}{x}=\dfrac{11x-34}{x^2-3x-4}\)
=>\(11\left(x^2-3x-4\right)=x\left(11x-34\right)\)
=>\(11x^2-33x-44=11x^2-34x\)
=>-33x-44=-34x
=>-33x+34x=44
=>x=44(nhận)
b: ĐKXĐ: \(x\ne4\)
\(\dfrac{14}{3x-12}-\dfrac{2+x}{x-4}=\dfrac{3}{8-2x}-\dfrac{5}{6}\)
=>\(\dfrac{14}{3\left(x-4\right)}-\dfrac{x+2}{x-4}=\dfrac{-3}{2\left(x-4\right)}-\dfrac{5}{6}\)
=>\(\dfrac{28}{6\left(x-4\right)}-\dfrac{6\left(x+2\right)}{6\left(x-4\right)}=\dfrac{-9}{6\left(x-4\right)}-\dfrac{5\left(x-4\right)}{6\left(x-4\right)}\)
=>28-6(x+2)=-9-5(x-4)
=>28-6x-12=-9-5x+20
=>-6x+16=-5x+11
=>-6x+5x=11-16
=>-x=-5
=>x=5(nhận)
c: ĐKXĐ: \(x\notin\left\{\dfrac{1}{3};-\dfrac{1}{3}\right\}\)
\(\dfrac{12}{1-9x^2}=\dfrac{1-3x}{1+3x}-\dfrac{1+3x}{1-3x}\)
=>\(\dfrac{12}{\left(1-3x\right)\left(1+3x\right)}=\dfrac{\left(1-3x\right)^2-\left(1+3x\right)^2}{\left(1+3x\right)\left(1-3x\right)}\)
=>\(\left(1-3x\right)^2-\left(1+3x\right)^2=12\)
=>\(9x^2-6x+1-9x^2-6x-1=12\)
=>-12x=12
=>x=-1(nhận)
d: ĐKXĐ: \(x\notin\left\{0;5;-5\right\}\)
\(\dfrac{x+5}{x^2-5x}-\dfrac{x+25}{2x^2-50}=\dfrac{x-5}{2x^2+10x}\)
=>\(\dfrac{x+5}{x\left(x-5\right)}-\dfrac{x+25}{2\left(x-5\right)\left(x+5\right)}=\dfrac{x-5}{2x\left(x+5\right)}\)
=>\(\dfrac{2\left(x+5\right)^2}{2x\left(x-5\right)\left(x+5\right)}-\dfrac{x\left(x+25\right)}{2x\left(x-5\right)\left(x+5\right)}=\dfrac{\left(x-5\right)^2}{2x\left(x+5\right)\left(x-5\right)}\)
=>\(2\left(x+5\right)^2-x\left(x+25\right)=\left(x-5\right)^2\)
=>\(2x^2+20x+50-x^2-25x=x^2-10x+25\)
=>-5x+50=-10x+25
=>5x=-25
=>x=-5(loại)
Bài 2:
a: ĐKXĐ: \(x\notin\left\{2;5\right\}\)
\(\dfrac{6x+1}{x^2-7x+10}+\dfrac{5}{x-2}=\dfrac{3}{x-5}\)
=>\(\dfrac{6x+1}{\left(x-2\right)\left(x-5\right)}+\dfrac{5\left(x-5\right)}{\left(x-2\right)\left(x-5\right)}=\dfrac{3\left(x-2\right)}{\left(x-2\right)\left(x-5\right)}\)
=>6x+1+5x-25=3x-6
=>11x-24=3x-6
=>8x=18
=>x=9/4(nhận)
b: ĐKXĐ: \(x\notin\left\{0;2;-2\right\}\)
\(\dfrac{2}{x^2-4}-\dfrac{x-1}{x\left(x-2\right)}+\dfrac{x-4}{x\left(x+2\right)}=0\)
=>\(\dfrac{2x}{x\left(x-2\right)\left(x+2\right)}-\dfrac{\left(x-1\right)\left(x+2\right)}{x\left(x-2\right)\left(x+2\right)}+\dfrac{\left(x-4\right)\left(x-2\right)}{x\left(x-2\right)\left(x+2\right)}=0\)
=>2x-(x-1)(x+2)+(x-4)(x-2)=0
=>\(2x-\left(x^2+x-2\right)+x^2-6x+8=0\)
=>\(x^2-4x+8-x^2-x+2=0\)
=>-5x+10=0
=>x=2(loại)
c: ĐKXĐ: \(x\notin\left\{3;-1\right\}\)
\(\dfrac{1}{3-x}-\dfrac{1}{x+1}=\dfrac{x}{x-3}-\dfrac{\left(x-1\right)^2}{x^2-2x-3}\)
=>\(\dfrac{-1}{x-3}-\dfrac{1}{x+1}-\dfrac{x}{x-3}+\dfrac{\left(x-1\right)^2}{\left(x-3\right)\left(x+1\right)}=0\)
=>\(\dfrac{\left(-1-x\right)\left(x+1\right)-x+3}{\left(x-3\right)\left(x+1\right)}+\dfrac{\left(x-1\right)^2}{\left(x-3\right)\left(x+1\right)}=0\)
=>-(x+1)^2-x+3+(x-1)2=0
=>\(-x^2-2x-1-x+3+x^2-2x+1=0\)
=>-5x+3=0
=>\(x=\dfrac{3}{5}\left(nhận\right)\)
d: ĐKXĐ: \(x\notin\left\{2;-3\right\}\)
\(\dfrac{1}{x-2}-\dfrac{6}{x+3}=\dfrac{5}{6-x^2-x}\)
=>\(\dfrac{x+3-6\left(x-2\right)}{\left(x+3\right)\left(x-2\right)}=\dfrac{-5}{\left(x+3\right)\left(x-2\right)}\)
=>x+3-6(x-2)=-5
=>x+3-6x+12+5=0
=>-5x+20=0
=>x=4(nhận)
e: ĐKXĐ: x<>-2
\(\dfrac{2}{x+2}-\dfrac{2x^2+16}{x^3+8}=\dfrac{5}{x^2-2x+4}\)
=>\(\dfrac{2}{x+2}-\dfrac{2x^2+16}{\left(x+2\right)\left(x^2-2x+4\right)}-\dfrac{5}{x^2-2x+4}=0\)
=>\(\dfrac{2\left(x^2-2x+4\right)-2x^2-16-5x-10}{\left(x+2\right)\left(x^2-2x+4\right)}=0\)
=>\(2x^2-4x+8-2x^2-5x-26=0\)
=>-9x-18=0
=>x=-2(loại)
f: ĐKXĐ: \(x\notin\left\{1;-1\right\}\)
\(\dfrac{x+1}{x^2+x+1}-\dfrac{x-1}{x^2-x+1}=\dfrac{2\left(x+2\right)^2}{x^6-1}\)
=>\(\dfrac{\left(x+1\right)\left(x^2-x+1\right)-\left(x-1\right)\left(x^2+x+1\right)}{\left(x^2+x+1\right)\left(x^2-x+1\right)}=\dfrac{2\left(x+2\right)^2}{\left(x-1\right)\left(x+1\right)\left(x^2+x+1\right)\left(x^2-x+1\right)}\)
=>\(\dfrac{2}{\left(x^2+x+1\right)\left(x^2-x+1\right)}=\dfrac{2\left(x+2\right)^2}{\left(x^2-1\right)\left(x^2+x+1\right)\left(x^2-x+1\right)}\)
=>2(x^2-1)=2(x+2)^2
=>\(x^2-1=\left(x+2\right)^2\)
=>\(x^2+4x+4-x^2+1=0\)
=>4x+5=0
=>\(x=-\dfrac{5}{4}\left(nhận\right)\)
Bài 3:
c:
=>\(\dfrac{x}{x-1}+\dfrac{x}{x-2}+\dfrac{x}{x-3}=\dfrac{3x-12}{x-6}\)
=>
ĐKXĐ: \(x\notin\left\{1;2;\dfrac{3\pm\sqrt{7}}{2}\right\}\)
\(\dfrac{4}{x^2-3x+2}-\dfrac{3}{2x^2-6x+1}+1=0\)
=>\(\dfrac{4\left(2x^2-6x+1\right)-3\left(x^2-3x+2\right)}{\left(x^2-3x+2\right)\left(2x^2-6x+1\right)}=-1\)
=>\(8x^2-24x+4-3x^2+9x-6=-\left(x^2-3x+2\right)\left[2\cdot\left(x^2-3x\right)+1\right]\)
=>\(5x^2-15x-2=-\left[2\left(x^2-3x\right)^2+5\left(x^2-3x\right)+2\right]\)
=>\(5\left(x^2-3x\right)-2+2\left(x^2-3x\right)^2+5\left(x^2-3x\right)+2=0\)
=>\(2\left(x^2-3x\right)^2+10\left(x^2-3x\right)=0\)
=>\(\left(x^2-3x\right)^2+5\left(x^2-3x\right)=0\)
=>\(\left(x^2-3x\right)\left(x^2-3x+5\right)=0\)
mà \(x^2-3x+5=\left(x-\dfrac{3}{2}\right)^2+\dfrac{11}{4}>=\dfrac{11}{4}>0\forall x\)
nên x(x-3)=0
=>\(\left[{}\begin{matrix}x=0\left(nhận\right)\\x=3\left(nhận\right)\end{matrix}\right.\)
a:
ĐKXĐ: \(x\notin\left\{8;9;10;11\right\}\)
\(\dfrac{8}{x-8}+\dfrac{11}{x-11}=\dfrac{9}{x-9}+\dfrac{10}{x-10}\)
=>\(\left(\dfrac{8}{x-8}+1\right)+\left(\dfrac{11}{x-11}+1\right)=\left(\dfrac{9}{x-9}+1\right)+\left(\dfrac{10}{x-10}+1\right)\)
=>\(\dfrac{x}{x-8}+\dfrac{x}{x-11}-\dfrac{x}{x-9}-\dfrac{x}{x-10}=0\)
=>\(x\left(\dfrac{1}{x-8}+\dfrac{1}{x-11}-\dfrac{1}{x-9}-\dfrac{1}{x-10}\right)=0\)
=>x=0(nhận)
b:
ĐKXĐ: \(x\notin\left\{3;4;5;6\right\}\)
\(\dfrac{x}{x-3}-\dfrac{x}{x-5}=\dfrac{x}{x-4}-\dfrac{x}{x-6}\)
=>\(\dfrac{x\left(x-5\right)-x\left(x-3\right)}{\left(x-3\right)\left(x-5\right)}=\dfrac{x\left(x-6\right)-x\left(x-4\right)}{\left(x-4\right)\left(x-6\right)}\)
=>\(\dfrac{-2x}{\left(x-3\right)\left(x-5\right)}=\dfrac{-2x}{\left(x-4\right)\left(x-6\right)}\)
=>\(x\left(\dfrac{1}{\left(x-3\right)\left(x-5\right)}-\dfrac{1}{\left(x-4\right)\left(x-6\right)}\right)=0\)
=>\(x\cdot\dfrac{\left(x-4\right)\left(x-6\right)-\left(x-3\right)\left(x-5\right)}{\left(x-3\right)\left(x-5\right)\left(x-4\right)\left(x-6\right)}=0\)
=>\(x\left(x^2-10x+24-x^2+8x-15\right)=0\)
=>x(-2x+9)=0
=>\(\left[{}\begin{matrix}x=0\left(nhận\right)\\x=\dfrac{9}{2}\left(nhận\right)\end{matrix}\right.\)

Dựng hình bình hành ABCD.
Khi đó vì M là trung điểm AC nên M cũng là trung điểm BD \(\Rightarrow\) M, B, D thẳng hàng.
Lại có ABCD là hình bình hành \(\Rightarrow AD=BC\)
Mà \(AK=BC\) nên \(AK=AD\)
\(\Rightarrow\Delta ADK\) cân tại A
\(\Rightarrow\widehat{AKD}=\widehat{ADK}\) hay \(\widehat{LKB}=\widehat{KDA}\)
Mặt khác, LB//AD nên \(\widehat{KDA}=\widehat{KBL}\) (2 góc đồng vị)
Do đó \(\widehat{LKB}=\widehat{KBL}\left(=\widehat{KDA}\right)\)
\(\Rightarrow\Delta KBL\) cân tại L
\(\Rightarrow LK=BL\) (đpcm)

\(\dfrac{P\left(x\right)}{x-3}=\dfrac{x^3-2x^2-x+a}{x-3}\)
\(=\dfrac{x^3-3x^2+x^2-3x+2x-6+a+6}{x-3}\)
\(=x^2+x+2+\dfrac{a+6}{x-3}\)
Để P(x) chia x-3 dư 5 thì a+6=5
=>a=-1

\(\left(4x+1\right)\left(12x-1\right)\left(3x+2\right)\left(x+1\right)-4\\ =\left[\left(4x+1\right)\left(3x+2\right)\right]\left[\left(12x-1\right)\left(x+1\right)\right]-4\\ =\left(12x^2+8x+3x+2\right)\left(12x^2+12x-x-1\right)-4\\ =\left(12x^2+11x+2\right)\left(12x^2+11x-1\right)-4\)
Đặt: `12x^2+11x=t`
\(\left(t+2\right)\left(t-1\right)-4=t^2+2t-t-2-4=t^2-t-6\\ =t^2-3t+2t-6\\ =t\left(t-3\right)+2\left(t-3\right)\\ =\left(t-3\right)\left(t+2\right)\\ =\left(12x^2+11x-3\right)\left(12x^2+11x+2\right)\)

\(A=x^3-5x^2+3x+9\\ =x^3+x^2-6x^2-6x+9x+9\\ =x^2\left(x+1\right)-6x\left(x+1\right)+9\left(x+1\right)\\ =\left(x^2-6x+9\right)\left(x+1\right)\\ =\left(x-3\right)^2\left(x+1\right)\)
A = \(x^3\) - 5\(x^2\) + 3\(x\) + 9
A = \(x^3\) - 3\(x^2\) - \(x^2\) - \(x^2\) + 3\(x\) + 9
A = (\(x^3\) - 3\(x^2\)) - (\(x^2\) - 3\(x\)) - (\(x^2\) - 9)
A = \(x^2\)(\(x\) - 3) - \(x\)(\(x\) - 3) - (\(x\) - 3)(\(x\) + 3)
A = (\(x\) - 3)(\(x^2\) - \(x\) - \(x\) - 3)
A = (\(x\) - 3)[\(x^2\) - (\(x+x\)) - 3]
A = (\(x\) - 3)[\(x^2\) - 2\(x\) - 3]

\(2x^2-3x+1\\ =2x^2-x-2x+1\\ =\left(2x^2-x\right)-\left(2x-1\right)\\ =x\left(2x-1\right)-\left(2x-1\right)\\ =\left(2x-1\right)\left(x-1\right)\)
\(2x^2-3x+1\\ =2x^2-2x-x+1\\ =2x\left(x-1\right)-\left(x-1\right)\\ =\left(2x-1\right)\left(x-1\right)\)