x7 + x2 + 1
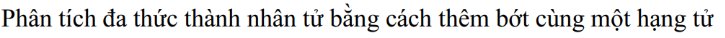
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) Thay x=2 vào ta có:
\(2^2-4m\cdot2+1=0\\ \Leftrightarrow4-8m+1=0\\ \Leftrightarrow5-8m=0\\ \Leftrightarrow8m=5\\ \Leftrightarrow m=\dfrac{5}{8}\)
b) Thay x=2 vào ta có:
\(3\cdot2^2-5m\cdot2+7\\ \Leftrightarrow12-10m+7=0\\ \Leftrightarrow19-10m=0\\ \Leftrightarrow10m=19\\\Leftrightarrow m=\dfrac{19}{10}\)
a:
Đặt \(x^2-4mx+1=0\left(1\right)\)
Thay x=2 vào (1), ta được:
\(2^2-4m\cdot2+1=0\)
=>\(4-8m+1=0\)
=>5-8m=0
=>8m=5
=>\(m=\dfrac{5}{8}\)
b: Đặt \(3x^2-5mx+7=0\left(2\right)\)
Thay x=2 vào (2), ta được:
\(3\cdot2^2-5m\cdot2+7=0\)
=>12-10m+7=0
=>19-10m=0
=>10m=19
=>\(m=\dfrac{19}{10}\)

a)5x+17-(2x+5)=0
=>5x+17-2x-5=0
=>3x+12=0
=>3x=-12
=>x=-12:3=-4
b)3(1-x)-(5-2x)=0
=>3-3x-5+2x=0
=>-2-x=0
=>x=-2
c)2(x-1)-3(x-2)=0
=>2x-2-3x+6=0
=>-x+4=0
=>x=4
d)(x-3)(2x-5)+(2x-4)(5-2x)=0
=>(x-3)(2x-5)-(2x-4)(2x-5)=0
=>(2x-5)(x-3-2x+4)=0
=>(2x-5)(1-x)=0
TH1: 2x - 5=0=>2x=5=>x=5/2
TH2: 1-x=0=>x=1
a: Đặt 5x+17-(2x+5)=0
=>\(5x+17-2x-5=0\)
=>\(3x+12=0\)
=>\(3x=-12\)
=>\(x=-\dfrac{12}{3}=-4\)
b: Đặt \(3\left(1-x\right)-\left(5-2x\right)=0\)
=>\(3-3x-5+2x=0\)
=>\(-x-2=0\)
=>x+2=0
=>x=-2
c: Đặt \(2\left(x-1\right)-3\left(x-2\right)=0\)
=>\(2x-2-3x+6=0\)
=>4-x=0
=>x=4
d: Sửa đề: (x-3)(2x-5)+(2x-4)*(5-x)
Đặt \(\left(x-3\right)\left(2x-5\right)+\left(2x-4\right)\left(5-x\right)=0\)
=>\(2x^2-5x-6x+15+10x-2x^2-20+4x=0\)
=>3x-5=0
=>3x=5
=>\(x=\dfrac{5}{3}\)

Đặt 5x+17-(2x+5)=0
=>5x+17-2x-5=0
=>3x+12=0
=>3x=-12
=>\(x=-\dfrac{12}{3}=-4\)

1: \(\left(2x-1\right)^2-\left(4x^2-1\right)=0\)
=>\(\left(2x-1\right)^2-\left(2x-1\right)\left(2x+1\right)=0\)
=>\(\left(2x-1\right)\left(2x-1-2x-1\right)=0\)
=>-2(2x-1)=0
=>2x-1=0
=>\(x=\dfrac{1}{2}\)
2: \(\left(x+2\right)^2-x\left(x-3\right)=2\)
=>\(x^2+4x+4-x^2+3x=2\)
=>7x+4=2
=>7x=-2
=>\(x=-\dfrac{2}{7}\)
3: \(\left(x-5\right)^2-x\left(x+2\right)=5\)
=>\(x^2-10x+25-x^2-2x=5\)
=>-12x+25=5
=>-12x=5-25=-20
=>\(x=\dfrac{20}{12}=\dfrac{5}{3}\)
4: \(\left(x-1\right)^2+x\left(4-x\right)=11\)
=>\(x^2-2x+1+4x-x^2=11\)
=>2x+1=11
=>2x=10
=>x=5
5: \(\left(x-3\right)\left(x+3\right)=\left(x-5\right)^2\)
=>\(x^2-9=x^2-10x+25\)
=>-10x+25=-9
=>-10x=-25-9=-34
=>\(x=\dfrac{34}{10}=\dfrac{17}{5}\)
6: \(\left(2x+1\right)^2-4x\left(x-1\right)=17\)
=>\(4x^2+4x+1-4x^2+4x=17\)
=>8x+1=17
=>8x=16
=>x=2
7: \(\left(3x+1\right)^2-9x\left(x-2\right)=25\)
=>\(9x^2+6x+1-9x^2+18x=25\)
=>24x+1=25
=>24x=24
=>x=1
8: \(\left(3x-2\right)\left(3x+2\right)-9x\left(x-1\right)=0\)
=>\(9x^2-4-9x^2+9x=0\)
=>9x-4=0
=>9x=4
=>\(x=\dfrac{4}{9}\)
9: \(\left(x+2\right)^2-\left(x-2\right)\left(x+2\right)=0\)
=>(x+2)(x+2-x+2)=0
=>4(x+2)=0
=>x+2=0
=>x=-2
10: \(\left(x+2\right)^2-\left(x-3\right)\left(x+3\right)=-3\)
=>\(x^2+4x+4-\left(x^2-9\right)+3=0\)
=>\(x^2+4x+7-x^2+9=0\)
=>4x+16=0
=>4x=-16
=>x=-4
11: \(\left(3x+2\right)^2-\left(3x-5\right)\left(3x+2\right)=0\)
=>(3x+2)(3x+2-3x+5)=0
=>7(3x+2)=0
=>3x+2=0
=>3x=-2
=>\(x=-\dfrac{2}{3}\)
12: \(\left(x+3\right)^2-\left(x+2\right)\left(x-2\right)=4x+17\)
=>\(x^2+6x+9-x^2+4=4x+17\)
=>6x+13=4x+17
=>2x=4
=>x=2
13: \(3\left(x-1\right)^2+\left(x+5\right)\left(-3x+2\right)=-25\)
=>\(3\left(x^2-2x+1\right)+2x-3x^2+10-15x=-25\)
=>\(3x^2-6x+3-3x^2-13x+10=-25\)
=>-19x+13=-25
=>-19x=-38
=>x=2
14: \(\left(x+3\right)^2+\left(x-2\right)^2=2x^2\)
=>\(x^2+6x+9+x^2-4x+4=2x^2\)
=>2x=-13
=>\(x=-\dfrac{13}{2}\)

a)
\(P=4x^4+y^4\\ =4x^4+4x^2y^2+y^4-4x^2y^2\\ =\left(4x^4+4x^2y^2+y^2\right)-4x^2y^2\\ =\left(2x^2+y^2\right)^2-\left(2xy\right)^2\\ =\left(2x^2-2xy+y^2\right)\left(2x^2+2xy+y^2\right)\)
b)
\(Q=x^4+64\\ =x^4+16x^2+64-16x^2\\ =\left(x^4+16x^2+64\right)-16x^2\\ =\left(x^2+8\right)^2-\left(4x\right)^2\\ =\left(x^2-4x+8\right)\left(x^2+4x+8\right)\)

Sửa đề: \(a^2+2ab+b^2-2a-2b+1\)
\(=\left(a^2+2ab+b^2\right)-2\left(a+b\right)+1\)
\(=\left(a+b\right)^2-2\left(a+b\right)\cdot1+1^2\)
\(=\left(a+b-1\right)^2\)

Xét tứ giác ABCD có \(\widehat{ABC}+\widehat{ADC}=180^0\)
nên ABCD là tứ giác nội tiếp
=>\(\widehat{DAC}=\widehat{DBC};\widehat{BAC}=\widehat{BDC}\)
mà \(\widehat{CDB}=\widehat{CBD}\)(CB=CD)
nên \(\widehat{DAC}=\widehat{BAC}\)
=>AC là phân giác của góc BAD

\(x^2+5y^2+2y-4xy-3=0\\ \Rightarrow\left(x^2-4xy+4y^2\right)+\left(y^2+2y+1\right)-4=0\\ \Rightarrow\left(x-2y\right)^2+\left(y+1\right)^2=4\)
Mình nghĩ bạn thiếu đề nhé
Bổ sung đề: Tìm cặp x, y nguyên thỏa mãn
Với x, y nguyên hiển nhiên x-2y và y+1 nguyên
Mà: \(4=0^2+2^2=0^2+\left(-2\right)^2\)
Các trường hợp xảy ra:
TH1: y+1=0 và x-2y=2
=> y=-1 và x=0
TH2: y+1=0 và x-2y=-2
=> y=-1 và x=-4
TH3: y+1=2 và x-2y=0
=> y=1 và x=2
TH4: y+1=-2 và x-2y=0
=> y=-3 và x=-6
Vậy (x;y)=(0;-1);(-4;-1);(2;1);(-6;-3)
\(x^7+x^2+1\)
\(=x^7+x^6+x^5-x^6-x^5-x^4+x^4+x^3+x^2-x^3-x^2-x+x^2+x+1\)
\(=x^5\left(x^2+x+1\right)-x^4\left(x^2+x+1\right)+x^2\left(x^2+x+1\right)-x\left(x^2+x+1\right)+\left(x^2+x+1\right)\)
\(=\left(x^2+x+1\right)\left(x^5-x^4+x^2-x+1\right)\)