Tìm số tự nhiên �a, biết rằng ��=0an=0 với mọi �∈�∗n∈N*
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Bài 2:
2a, 7,2: 2,4 \(\times\) \(x\) = 4,5
3 \(\times\) \(x\) = 4,5
\(x\) = 4,5 : 3
\(x\) = 1,5
2b, 9,15 \(\times\) \(x\) + 2,85 \(\times\) \(x\) = 48
\(x\) \(\times\) ( 9,15 + 2,85) = 48
\(x\) \(\times\) 12 = 48
\(x\) = 48 : 12
\(x\) = 4
c, (\(x\) \(\times\) 3 + 4): 5 = 8
\(x\) \(\times\) 3 + 4 = 8 \(\times\) 5
\(x\) \(\times\) 3 + 4 = 40
\(x\) \(\times\) 3 = 40 - 4
\(x\) \(\times\) 3 = 36
\(x\) = 36 : 3
\(x\) = 12

Lời giải:
a. $5\times (4+6x)=290$
$4+6x=290:5=58$
$6x=58-4=54$
$x=54:6=9$
b. $x\times 3,7+x\times 6,3=120$
$x\times (3,7+6,3)=120$
$x\times 10=120$
$x=120:10=12$
c.
$(15\times 24-x):0,25=100:\frac{1}{4}=100:0,25$
$15\times 24-x=100$
$360-x=100$
$x=360-100=260$
d.
$128\times x-12\times x-16\times x=5208000$
$x\times (128-12-16)=5208000$
$x\times 100=5208000$
$x=5208000:100=52080$
e.
$5\times x+3,75\times x+1,25\times x=20$
$x\times (5+3,75+1,25)=20$
$x\times 10=20$
$x=20:10=2$
g.
$(84,6-2\times x):3,02=5,1$
$84,6-2\times x=5,1\times 3,02=15,402$
$2\times x=84,6-15,402=69,198$
$x=69,198:2=34,599$

\(a^2+10⋮a-1\left(a\inℕ\right)\)
\(\Rightarrow a^2+10-a\left(a-1\right)⋮a-1\)
\(\Rightarrow a^2+10-a^2+a⋮a-1\)
\(\Rightarrow a+10⋮a-1\)
\(\Rightarrow a+10-\left(a-1\right)⋮a-1\)
\(\Rightarrow a+10-a+1⋮a-1\)
\(\Rightarrow11⋮a-1\)
\(\Rightarrow a-1\in U\left(11\right)=\left\{1;11\right\}\)
\(\Rightarrow a\in\left\{2;12\right\}\) thỏa đề bài

5.(2⁵ - 10) : 2³
= 5.22 : 8
= 110 : 8
= 13,75
Cả 4 đáp án đều sai
\(5.\left(2^5-10\right):2^3\\ =5.\left(32-10\right):8\\ =5.22:8\\ =110:8\\ =13,75\)
Vậy không có đáp án nào đúng

a) n + 9 ⋮ n - 1
⇒ n - 1 + 10 ⋮ n - 1
⇒ 10 ⋮ n - 1
⇒ n - 1 ϵ Ư(10) = {1; -1; 2; -2; 5; -5; 10; -10}
⇒ n ϵ {2; 0; 3; -1; 6; -4; 11; -9}
b) n + 5 ⋮ 2n + 3
⇒ 2(n + 5) ⋮ 2n + 3
⇒ 2n + 10 ⋮ 2n + 3
⇒ 2n + 3 + 7 ⋮ 2n + 3
⇒ 7 ⋮ 2n + 3
⇒ 2n + 3 ϵ Ư(7) = {1; -1; 7; -7}
⇒ n ϵ {-1; -2; 2; -5}
c) 2n + 4 ⋮ n + 6
⇒ 2n + 12 - 8 ⋮ n + 6
⇒ 2(n + 6) - 8 ⋮ n + 6
⇒ 8 ⋮ n + 6
⇒ n + 6 ϵ Ư(8) = {1; -1; 2; -2; 4; -4; 8; -8}
⇒ n ϵ {-5; -7; -4; -8; -2; -10; 2; -14}

Với x = 2, ta có:
C = (4.2 - 6)/(2.2 + 1)
= 2/5 không phải số nguyên
Em xem lại đề nhé
\(C=\dfrac{4x-6}{2x+1}\\ =\dfrac{4x+2-8}{2x+1}\\ =\dfrac{2\left(2x+1\right)-8}{2x+1}\)
Ta có:
\(2\left(2x+1\right)⋮2x+1\)
Để \(\dfrac{4x-6}{2x+1}\inℤ\)
\(\rightarrow-8⋮2x+1\)
hay \(2x+1\inƯ\left(8\right)=\left\{\pm1;\pm2;\pm4;\pm8\right\}\)
Ta có bảng sau:
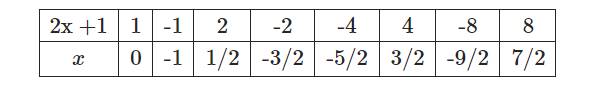
Vậy \(x\in\left\{0;-1;\dfrac{1}{2};-\dfrac{3}{2};-\dfrac{5}{2};\dfrac{3}{2};-\dfrac{9}{2};\dfrac{7}{2}\right\}\) thì \(\dfrac{4x-6}{2x+1}\inℤ\)

D = 3\(\dfrac{1}{2}\) \(\times\) \(\dfrac{12}{43}\) + \(\dfrac{49}{14}\) \(\times\) \(\dfrac{31}{43}\) + \(\dfrac{11}{2}\)
D = \(\dfrac{7}{2}\) \(\times\) \(\dfrac{12}{43}\) + \(\dfrac{7}{2}\) \(\times\) \(\dfrac{31}{43}\) + \(\dfrac{11}{2}\)
D = \(\dfrac{7}{2\times43}\) \(\times\) (12 + 31) + \(\dfrac{11}{2}\)
D = \(\dfrac{7}{2\times43}\) \(\times\) 43 + \(\dfrac{11}{2}\)
D = \(\dfrac{7}{2}\) + \(\dfrac{11}{2}\)
D = 9
D = 3 và 1/2 x 12/43 + 49/14 x 31/43 + 11/2
= 7/2 x 12/43 + 49/14 x 31/43 + 11/2
= 42/43 + 217/36 + 11/2
=9
# https://lazi.vn/user/tnsociu

A = 101.102.103.104...108
A = 101+2+3+..+8
A = 1036

A = 1.2 + 2.3 + 3.4 + 4.5 + ... + 99.100 + 100.101
3.A = 1.2.3 + 2.3.3 +3.4.3 + ... + 100.101.3
3A= 1.2.3 + 2.3.(4 - 1) + 3.4.(5 - 2) + ... + 100.101.(102 - 99)
3A = 1.2.3 + 2.3.4 - 1.2.3 + 2.3.4 -3.4.5 + ... +99.100.101 -100.101.102
3A = 99.100.101
A = 99.100.101 : 3
A = 33.100.101
Vậy A = 33. 100 .101 = 333300
{[(100+101)+100]+[(103.104.105 ):106 ].[(107 :108).109].1010}.1011=???
{[(1+10)+100]+[(1K.10K.100K):1M].[(10M:100M).1B].10B}.100B
K= nghìn
M= triệu
B= tỷ

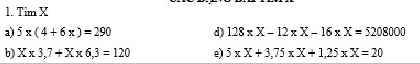

an = 0 ∀ n \(\in\) N*
⇒ a = 0