Bài 1 a, -5 \(\in\) Q; b, \(\dfrac{2}{-3}\) \(\notin\) I; c, \(\dfrac{3}{-5}\) \(\in\) R

d, N \(\subset\) Z \(\subset\) Q \(\subset\) R 

e, -\(\sqrt{25}\) \(\notin\) N; f, \(\sqrt{17}\) \(\in\) R

Bài 2

a, -0,33 \(\in\) Q;              b, 0,5241 \(\notin\) I;

c, 1,4142135... \(\in\) R;    d, Q \(\subset\) R

`#040911`

`b)`

\(x+\dfrac{1}{2}-x-\dfrac{2}{3}=\dfrac{1}{2}\\ \Rightarrow x+\dfrac{1}{2}-x-\dfrac{2}{3}-\dfrac{1}{2}=0\\ \Rightarrow\left(x-x\right)+\left(\dfrac{1}{2}-\dfrac{2}{3}-\dfrac{1}{2}\right)=0\\ \Rightarrow-\dfrac{2}{3}=0\left(\text{vô lý}\right)\\ \text{Vậy, }x\in\varnothing\)

`c)`

\(\left|x+1\right|=5\\ \Rightarrow\left[{}\begin{matrix}x+1=5\\x+1=-5\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=5-1\\x=-5-1\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=4\\x=-6\end{matrix}\right.\\ \text{Vậy, }x\in\left\{-6;4\right\}.\)

\(x+\dfrac{1}{2}-x-\dfrac{2}{3}=\dfrac{1}{2}\\ -\dfrac{2}{3}=\dfrac{1}{2}-\dfrac{1}{2}\\ -\dfrac{2}{3}=0\left(vô.lí\right)\\ Không.x.thoả\\ ----\\ \left|x+1\right|=5\\ \Leftrightarrow\left[{}\begin{matrix}x+1=5\\x+1=-5\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=4\\x=-6\end{matrix}\right.\)

`#040911`

`a)`

`2x^2 - 3x = 0`

`\Rightarrow x(2x - 3) = 0`

`\Rightarrow`\(\left[{}\begin{matrix}x=0\\2x-3=0\end{matrix}\right.\)

`\Rightarrow`\(\left[{}\begin{matrix}x=0\\2x=3\end{matrix}\right.\)

`\Rightarrow`\(\left[{}\begin{matrix}x=0\\x=\dfrac{3}{2}\end{matrix}\right.\)

Vậy, \(x\in\left\{0;\dfrac{3}{2}\right\}\)

`b)`

\(x+\dfrac{1}{2}-z-\dfrac{2}{3}=\dfrac{1}{2}?\)

Bạn xem lại đề

`c)`

\(x^3-x^2=0\\ \Rightarrow x^2\cdot\left(x-1\right)=0\\ \Rightarrow\left[{}\begin{matrix}x^2=0\\x-1=0\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=0\\x=1\end{matrix}\right.\)

Vậy, \(x\in\left\{0;1\right\}.\)

\(a,2x^2-3x=0\\ \Leftrightarrow x\left(2x-3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\2x-3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{3}{2}\end{matrix}\right.\\ b,Xem.lại,đề\\ c,x^3-x^2=0\\ \Leftrightarrow x^2.\left(x-1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\end{matrix}\right.\)

A B C M E H K

a/

Ta có

tg ABC vuông cân tại A

\(\Rightarrow\widehat{ABC}=\widehat{MCA}\)

Mà \(\widehat{ABC}+\widehat{MCA}=180^o-\widehat{A}=180^o-90^o=90^o\)

\(\Rightarrow\widehat{ABC}=\widehat{MCA}=\dfrac{90^o}{2}=45^o\)

Ta có

\(MB=MC\Rightarrow AM\perp BC\Rightarrow\widehat{AMB}=90^o\) (Trong tg cân đường trung tuyến xp từ đỉnh tg cân đồng thời là đường cao)

Xét tg vuông AMB

\(\widehat{BAM}=180^o-\left(\widehat{ABC}+\widehat{AMB}\right)=180^o-\left(45^o+90^o\right)=45^o\)

\(\Rightarrow\widehat{BAM}=\widehat{MCA}=45^o\)

b/

Xét tg vuông EAM có

\(\widehat{EAM}=180^o-\left(\widehat{AME}+\widehat{AEM}\right)=180^o-\left(90^o+\widehat{AEM}\right)\) (1)

Xét tg vuông KCE có

\(\widehat{KCE}=180^o-\left(\widehat{CKE}+\widehat{CEK}\right)=180^o-\left(90^o+\widehat{CEK}\right)\) (2)

Mà \(\widehat{AEM}=\widehat{CEK}\) (góc đối đỉnh) (3)

Từ (1) (2) (3) \(\Rightarrow\widehat{EAM}=\widehat{KCE}\)

c/

Ta có

\(\widehat{BAM}=\widehat{MCA}=45^o\) (cmt)

\(\widehat{EAM}=\widehat{KCE}\) (cmt)

\(\Rightarrow\widehat{BAM}+\widehat{EAM}=\widehat{MCA}+\widehat{KCE}\Rightarrow\widehat{BAH}=\widehat{ACK}\)

Xét tg vuông BAH và tg vuông ACK có

\(\widehat{BAH}=\widehat{ACK}\) (cmt)

AB=AC (cạnh bên tg cân)

=> tg BAH = tg ACK (Hai tg vuông có cạnh huyền và góc nhọn tương ứng bằng nhau)

=> BH=AK

d/

Xét tg vuông AME có

\(\widehat{EAM}+\widehat{AEB}=90^o\)

Xét tg vuông BHE có

\(\widehat{EBH}+\widehat{AEB}=90^o\)

\(\Rightarrow\widehat{EAM}=\widehat{EBH}\) (cùng phụ với \(\widehat{AEB}\) )

Xét tg AMK và tg BMH có

\(\widehat{EAM}=\widehat{EBH}\) (cmt)

AK=BH (cmt)

\(AM=BM=CM=\dfrac{BC}{2}\) (trong tg vuông trung tuyến thuộc cạnh huyền bằng nửa cạnh huyền)

=> tg AMK = tg BMH (c.g.c)=> MH=MK => tg HMK cân tại M

d/

Ta có  tg AMK = tg BMH (cmt)

\(\Rightarrow\widehat{AKM}=\widehat{BHM}\)

Mà \(\widehat{BHM}+\widehat{MHK}=\widehat{BHK}=90^o\)

\(\Rightarrow\widehat{AKM}+\widehat{MHK}=90^o\)

Xét tg MHK có

\(\widehat{HMK}=180^o-\left(\widehat{AKM}+\widehat{MHK}\right)=180^o-90^o=90^o\)

=> tg HMK vuông cân tại M

A = (- 1,25)³. (\(\dfrac{2}{5}\))³

A = (-1,25 . \(\dfrac{2}{5}\))³

A = (- \(\dfrac{2}{4}\))³

A = (-\(\dfrac{1}{2}\))³

A =  \(\dfrac{-1}{8}\)

Ta thấy: \(\left(x-y+3\right)^2\ge0\forall x;y\)

              \(\left|y-3\right|\ge0\forall y\)

\(\Rightarrow\left(x-y+3\right)^2+\left|y-3\right|\ge0\forall x;y\)

Mặt khác: \(\left(x-y+3\right)^2+\left|y-3\right|\le0\)

\(\Rightarrow\left(x-y+3\right)^2+\left|y-3\right|=0\)

\(\Leftrightarrow\left\{{}\begin{matrix}\left(x-y+3\right)^2=0\\y-3=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x-y+3=0\\y=3\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x-3+3=0\\y=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=0\\y=3\end{matrix}\right.\)

Khi đó, biểu thức \(\left(x-2y+6\right)^{10}+27\) trở thành:

\(\left(0-2\cdot3+6\right)^{10}+27\)

\(=\left(-6+6\right)^{10}+27\)

\(=27\)

#Urushi

bạn viết rõ ra nhé

A)23/42-10/21

B)16/25-3/15

C)7/8-1/3-1/2

D)15/7-4/9-10/9

Vì \(b^2=ac\) ta suy ra \(\dfrac{a}{b}=\dfrac{b}{c}\). Đặt \(a=kb\) và \(b=kc\).

Khi đó \(\dfrac{a}{c}=\dfrac{k\left(kc\right)}{c}=k^2\). (1)

Từ tính chất của dãy tỉ số bằng nhau ta có:

\(\dfrac{a}{b}=\dfrac{b}{c}=\dfrac{2012b}{2012c}=\dfrac{a+2012b}{b+2012c}=k\), suy ra \(k^2=\dfrac{\left(a+2012b\right)^2}{\left(b+2012c\right)^2}\). (2)

Từ (1) và (2) suy ra \(k^2=\dfrac{a}{c}=\dfrac{\left(a+2012b\right)^2}{\left(b+2012c\right)^2}\) (đpcm)

Ta dựng đường thẳng CD qua song song với AB, ta có:

          \(\widehat{ACD}\) = \(\widehat{A}\) = 40⁰ (so le trong)

         \(\widehat{DCE}\)  + \(\widehat{ACD}\)  = \(\widehat{ACE}\) 

 ⇒ \(\widehat{DCE}\) = \(\widehat{ACE}\) - \(\widehat{ACD}\) = 100⁰ - 40⁰  = 60⁰

CD // AB ⇒ CD // EG 

 ⇒ \(\widehat{GEx}\) = \(\widehat{DCE}\) (hai góc đồng vị)

⇒  \(\widehat{GEx}\) = 60⁰ (vì hai góc đồng vị)

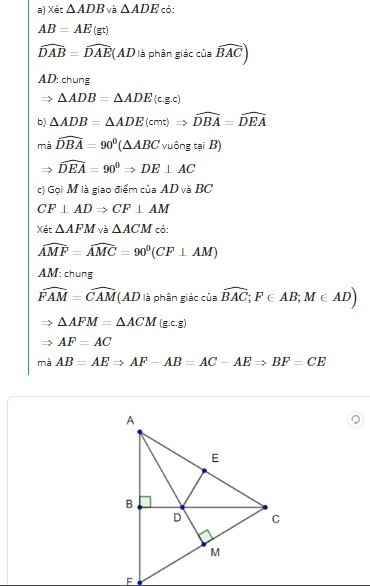
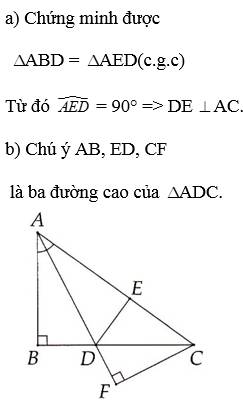
Xét \(\Delta ABC\) và \(\Delta ADE\) có:

\(AB=AD\left(gt\right)\)

\(\widehat{BAC}=\widehat{DAE}\) ( tính chất 2 góc đối đỉnh )

\(AC=AE\left(gt\right)\)

Vậy \(\Delta ABC=\) \(\Delta ADE\left(c.g.c\right)\)

\(\Rightarrow\widehat{C}=\widehat{E}\) ( 2 góc tương ứng )

Xét \(\Delta MAC\) và \(\Delta NAE\) có:

\(AC=AE\left(gt\right)\)

\(\widehat{C}=\widehat{E}\left(cmt\right)\)

\(CM=EN\left(gt\right)\)

Vậy \(\Delta MAC=\Delta NAE\left(c.g.c\right)\)

\(\Rightarrow\widehat{MAC}=\widehat{MAE}\) ( 2 góc tương ứng )

Ta có: \(\widehat{MAC}+\widehat{CAD}+\widehat{DAN}=\widehat{NAE}+\widehat{DAN}+\widehat{CAD}\)

\(\Rightarrow\widehat{MAN}=\widehat{CAE}\)

\(\Rightarrow\) 3 điểm \(M,A,N\) thẳng hàng.

Xét △ABC và △ADE ta có:⎧⎪⎨⎪⎩AB = AD (gt)∠BAC = ∠EAD (đđ)AC = AE (gt)⇒△ABC = △AED (c.g.c) {AB = AD (gt)∠BAC = ∠EAD (đđ)AC = AE (gt)⇒△ABC = △AED (c.g.c) 

⇒ ∠ABC = ∠AED (2 góc tương ứng)

Xét △ACM và △AEN ta có:

⎧⎪⎨⎪⎩CM = EN (gt)∠ACM = ∠AEN (cmt)AC = AE (gt)⇒△ACM = △AEN (c.g.c) {CM = EN (gt)∠ACM = ∠AEN (cmt)AC = AE (gt)⇒△ACM = △AEN (c.g.c) 

⇒ ∠CAM = ∠EAN (2 góc tương ứng)

Mà ∠CAM + ∠CAN = 180^o

⇒ ∠EAN + ∠CAN = 180^o

⇒ ∠MAN = 180^o

⇒ Ba điểm M, A, N thẳng hàng (đcpm).