
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a)
\(\dfrac{4}{x+3}-\dfrac{3}{x-5}=0\left(x\ne-3;x\ne5\right)\\ \Leftrightarrow\dfrac{4\left(x-5\right)}{\left(x+3\right)\left(x-5\right)}-\dfrac{3\left(x+3\right)}{\left(x+3\right)\left(x-5\right)}=0\\ \Leftrightarrow4\left(x-5\right)-3\left(x+3\right)=0\\ \Leftrightarrow4x-20-3x-9=0\\ \Leftrightarrow x-29=0\\ \Leftrightarrow x=29\left(tm\right)\)
b)
\(\dfrac{1}{x+2}-\dfrac{1}{x-2}=\dfrac{3x-12}{x^2-4}\left(x\ne\pm2\right)\\ \Leftrightarrow\dfrac{1}{x+2}-\dfrac{1}{x-2}=\dfrac{3x-12}{\left(x+2\right)\left(x-2\right)}\\ \Leftrightarrow\dfrac{x-2}{\left(x+2\right)\left(x-2\right)}-\dfrac{x+2}{\left(x+2\right)\left(x-2\right)}=\dfrac{3x-12}{\left(x+2\right)\left(x-2\right)}\\ \Leftrightarrow x-2-x-2=3x-12\\ \Leftrightarrow-4=3x-12\\ \Leftrightarrow3x=-4+12\\ \Leftrightarrow3x=8\\ \Leftrightarrow x=\dfrac{8}{3}\left(tm\right)\)
c)
\(\dfrac{1}{x+1}-\dfrac{4}{x^2-x+1}=\dfrac{2x^2+1}{x^3+1}\left(x\ne-1\right)\\ \Leftrightarrow\dfrac{x^2-x+1}{\left(x+1\right)\left(x^2-x+1\right)}-\dfrac{4\left(x+1\right)}{\left(x+1\right)\left(x^2-x+1\right)}=\dfrac{2x^2+1}{\left(x+1\right)\left(x^2-x+1\right)}\\ \Leftrightarrow x^2-x+1-4x-4=2x^2+1\\ \Leftrightarrow x^2-5x-3=2x^2+1\\ \Leftrightarrow2x^2-x^2+5x+1+3=0\\ \Leftrightarrow x^2+5x+4=0\\ \Leftrightarrow\left(x+1\right)\left(x+4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=-1\left(ktm\right)\\x=-4\left(tm\right)\end{matrix}\right.\)

a) Đặt: `4x-5=t`
\(t^2+2t+1=0\\ \Leftrightarrow\left(t+1\right)^2=0\\ \Leftrightarrow t+1=0\\ \Leftrightarrow t=-1\)
\(\Rightarrow4x-5=-1\\ \Leftrightarrow4x=-1+5\\ \Leftrightarrow4x=4\\ \Leftrightarrow x=1\)
b) Đặt: \(x^2-x=t\)
\(t\left(t+1\right)=6\\ \Leftrightarrow t^2+t-6=0\\ \Leftrightarrow t^2-2t+3t-6=0\\ \Leftrightarrow t\left(t-2\right)+3\left(t-2\right)=0\\ \Leftrightarrow\left(t-2\right)\left(t+3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}t=2\\t=-3\end{matrix}\right.\)
Với:
\(t=2\Rightarrow x^2-x=2\Leftrightarrow x^2-x-2=0\Leftrightarrow\left(x+1\right)\left(x-2\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=-1\\x=2\end{matrix}\right.\)
Với:
\(t=-3\Rightarrow x^2-x=-3\Leftrightarrow x^2-x+3=0\)
Mà: `x^2-x+3>0` nên vô lý

a)
\(3x\left(x-4\right)+7\left(x-4\right)=0\\ \Leftrightarrow\left(x-4\right)\left(3x+7\right)\\ \Leftrightarrow\left[{}\begin{matrix}x-4=0\\3x+7=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=4\\3x=-7\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=4\\x=-\dfrac{7}{3}\end{matrix}\right.\)
Vậy: ...
b)
\(\left(4x^2-9\right)+\left(x+2\right)\left(3-2x\right)=0\\ \Leftrightarrow\left(2x-3\right)\left(2x+3\right)-\left(x+2\right)\left(2x-3\right)=0\\ \Leftrightarrow\left(2x-3\right)\left(2x+3-x-2\right)=0\\ \Leftrightarrow\left(2x-3\right)\left(x+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{3}{2}\\x=-1\end{matrix}\right.\)
Vậy: ...
c)
\(\left(x-1\right)^2=\left(2x+3\right)^2\\ \Leftrightarrow\left(x-1\right)^2-\left(2x+3\right)^2=0\\ \Leftrightarrow\left(x-1+2x+3\right)\left(x-1-2x-3\right)=0\\ \Leftrightarrow\left(3x+2\right)\left(-x-4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}3x=-2\\-x=4\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{2}{3}\\x=-4\end{matrix}\right.\)
Vậy: ...
d)
\(x^2-6x+5=0\\ \Leftrightarrow x^2-5x-x+5=0\\ \Leftrightarrow x\left(x-5\right)-\left(x-5\right)=0\\ \Leftrightarrow\left(x-5\right)\left(x-1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=5\\x=1\end{matrix}\right.\)
Vậy: ...

a)
\(\left(x-5\right)\left(3x+4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-5=0\\3x+4=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=5\\3x=-4\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=5\\x=-\dfrac{4}{3}\end{matrix}\right.\)
Vậy: ...
b)
\(\left(\dfrac{2}{3}x-1\right)\left(\dfrac{1}{3}x+2\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}\dfrac{2}{3}x-1=0\\\dfrac{1}{3}x+2=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}\dfrac{2}{3}x=1\\\dfrac{1}{3}x=-2\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=1:\dfrac{2}{3}\\x=-2:\dfrac{1}{3}\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{3}{2}\\x=-6\end{matrix}\right.\)
Vậy: ...
c)
\(\left(x+7\right)\left(\dfrac{x}{3}-\dfrac{2-x}{6}\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x+7=0\\\dfrac{x}{3}-\dfrac{2-x}{6}=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-7\\\dfrac{2x}{6}-\dfrac{2-x}{6}=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-7\\\dfrac{2x-2+x}{6}=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-7\\3x-2=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-7\\3x=2\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-7\\x=\dfrac{2}{3}\end{matrix}\right.\)
Vậy: ...

a) y = 2x - 1 (a = 2; b=-1) và y = -x + 1 (a'=-1;b'=1)
Có a ≠ a' nên y = 2x - 1 và y = -x +1 cắt nhau nên hệ pt có 1 nghiệm duy nhất
b) 3x + 2y = 5 ⇔ \(y=\dfrac{5-3x}{2}=\dfrac{-3}{2}x+\dfrac{5}{2}\) (`a=-3/2;b=5/2`)
\(x-2y=2\Leftrightarrow y=\dfrac{2-x}{-2}\Leftrightarrow y=\dfrac{1}{2}x-1\left(a'=\dfrac{1}{2};b'=-1\right)\)
Có a ≠ a' nên hpt có 1 nghiệm duy nhất
c) \(x+y=2\Leftrightarrow y=-x+2\left(a=-1;b=2\right)\)
\(3x+3y=6\Leftrightarrow3\left(x+y\right)=6\Leftrightarrow x+y=2\Leftrightarrow y=-x+2\left(a'=-1;b=2\right)\)
Có a=a' và b=b' nên hpt có vô sô nghiệm

\(\left\{{}\begin{matrix}mx+y=3\\4x+my=6\end{matrix}\right.\) (1)
Để hpt có nghiệm thì: \(\dfrac{m}{4}\ne\dfrac{1}{m}\Leftrightarrow m\ne\pm2\)
\(\Leftrightarrow\left\{{}\begin{matrix}m^2x+my=3m\\4x+my=6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left(m^2-4\right)x=3m-6\\mx+y=3\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{3m-6}{m^2-4}=\dfrac{3}{m+2}\\\dfrac{3m}{m+2}+y=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{3}{m+2}\\y=3-\dfrac{3m}{m+2}=\dfrac{6}{m+2}\end{matrix}\right.\)
Mà: \(\left\{{}\begin{matrix}x_o>2\\y_o>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{3}{m+2}>2\\\dfrac{6}{m+2}>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-2< m< -\dfrac{1}{2}\\m>-2\end{matrix}\right.\Leftrightarrow-2< m< -\dfrac{1}{2}\)

Gọi T là giao điểm của EF và BC. Gọi J là trung điểm DT. Khi đó vì \(\widehat{TKD}=90^o\) nên \(K\in\left(J,JD\right)\). Đặt \(JB=b,JC=c,JD=JT=d\).
Dễ thấy \(AE=AF,BF=BD,CD=CE\) nên \(\dfrac{FA}{FB}.\dfrac{DB}{DC}.\dfrac{EC}{EA}=1\)
Hơn nữa, áp dụng định lý Menelaus cho tam giác ABC với cát tuyến EFT, ta có: \(\dfrac{FA}{FB}.\dfrac{TB}{TC}.\dfrac{EC}{EA}=1\)
Từ đó suy ra \(\dfrac{DB}{DC}=\dfrac{TB}{TC}\)
\(\Leftrightarrow\dfrac{JD-JB}{JC-JD}=\dfrac{JB+JT}{JC+JT}\)
\(\Leftrightarrow\dfrac{d-b}{c-d}=\dfrac{b+d}{c+d}\)
\(\Leftrightarrow\left(d-b\right)\left(c+d\right)=\left(c-d\right)\left(b+d\right)\)
\(\Leftrightarrow cd+d^2-bc-bd=bc+cd-bd-d^2\)
\(\Leftrightarrow2d^2=2bc\)
\(\Leftrightarrow JD^2=JB.JC=JK^2\) \(\left(vìJD=JK\right)\)
\(\Leftrightarrow\dfrac{JK}{JC}=\dfrac{JB}{JK}\)
Xét tam giác JBK và JKC, có:
\(\dfrac{JK}{JC}=\dfrac{JB}{JK}\) và \(\widehat{J}\) chung nên
\(\Delta JBK\sim\Delta JKC\left(c.g.c\right)\)
\(\Rightarrow\dfrac{KB}{KC}=\dfrac{JB}{JK}=\dfrac{JB}{JD}=\dfrac{b}{d}\)
Lại có \(d^2=bc\)
\(\Leftrightarrow d^2-bd=bc-bd\)
\(\Leftrightarrow d\left(d-b\right)=b\left(c-d\right)\)
\(\Leftrightarrow\dfrac{b}{d}=\dfrac{d-b}{c-d}\)
Như vậy \(\dfrac{KB}{KC}=\dfrac{b}{d}=\dfrac{d-b}{c-d}=\dfrac{JD-JB}{JC-JD}=\dfrac{DB}{DC}\)
Do đó theo tính chất đường phân giác trong tam giác, KD là phân giác \(\widehat{BKC}\) (đpcm)


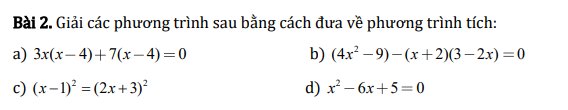
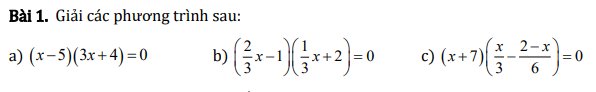


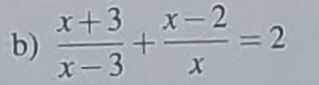
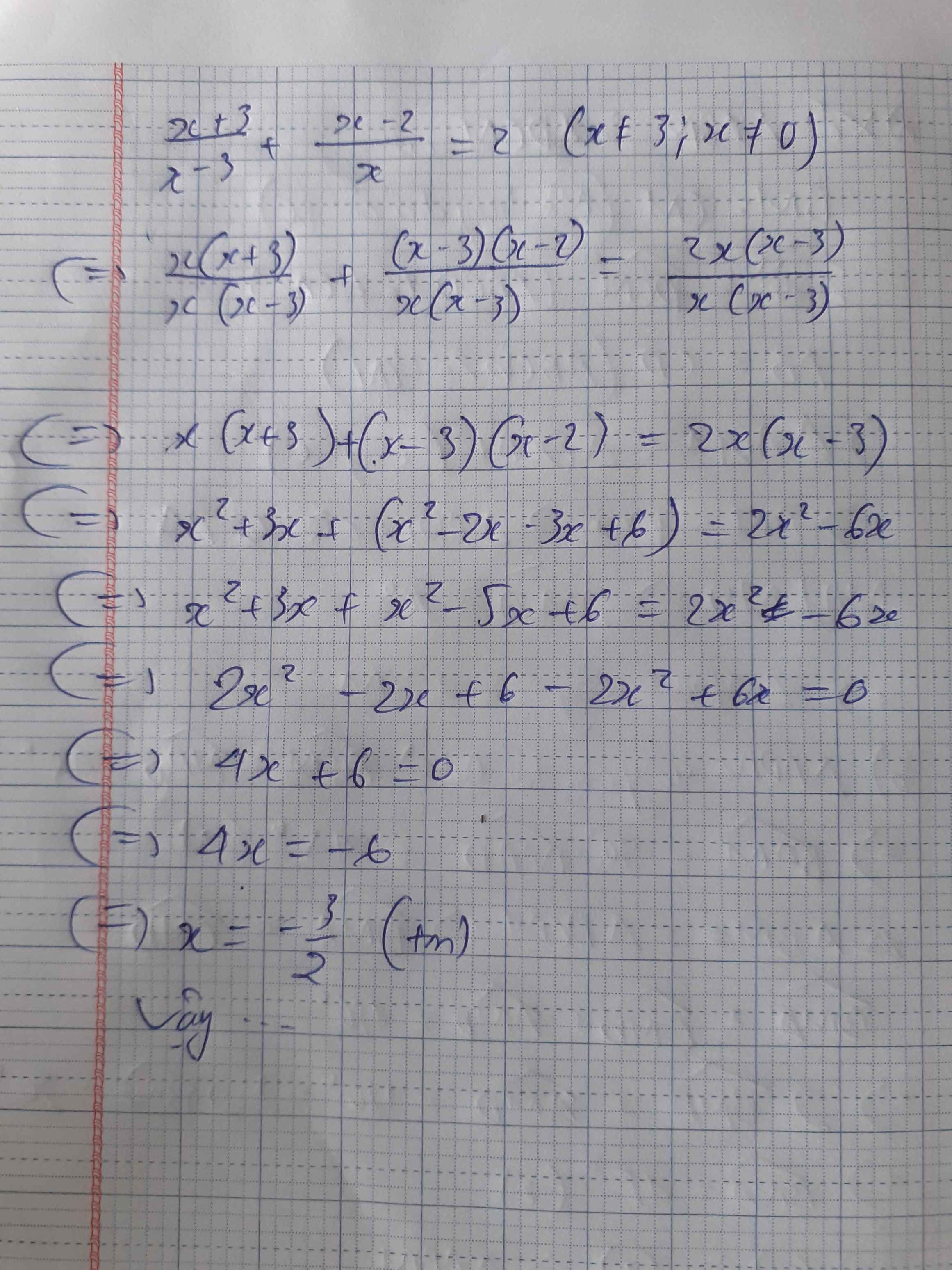

a) ĐKXĐ: `x\ne3;x\ne-1`
`\frac{x}{2(x-3)}+\frac{x}{2x+2}=\frac{2x}{(x+1)(x-3)}`
`\Leftrightarrow \frac{x(x+1)}{2(x+1)(x-3)}+\frac{x(x-3)}{2(x+1)(x-3)}=\frac{4x}{2x(x+1)(x-3)}`
`\Rightarrow x(x+1)+x(x-3)=4x`
`\Leftrightarrow 2x^2-2x=4x`
`\Leftrightarrow 2x^2-6x=0`
`\Leftrightarrow 2x(x-3)=0`
\(\Leftrightarrow \left[\begin{array}{} x=0(tm)\\x=3 (ktm)\end{array} \right.\)
b) ĐKXĐ: `x\ne-2`
`\frac{3x}{x^2-2x+4}=\frac{3}{x+2}+\frac{72}{x^3+8}`
`\Leftrightarrow \frac{3x(x+2)}{(x+2)(x^2-2x+4)}=\frac{3(x^2-2x+4)}{(x+2)(x^2-2x+4)}+\frac{72}{(x+2)(x^2-2x+4)}`
`\Rightarrow 3x^2+6x=3x^2-6x+12+72`
`\Leftrightarrow 12x=84`
`\Leftrightarrow x=7(tm)`
$\mathtt{Toru}$