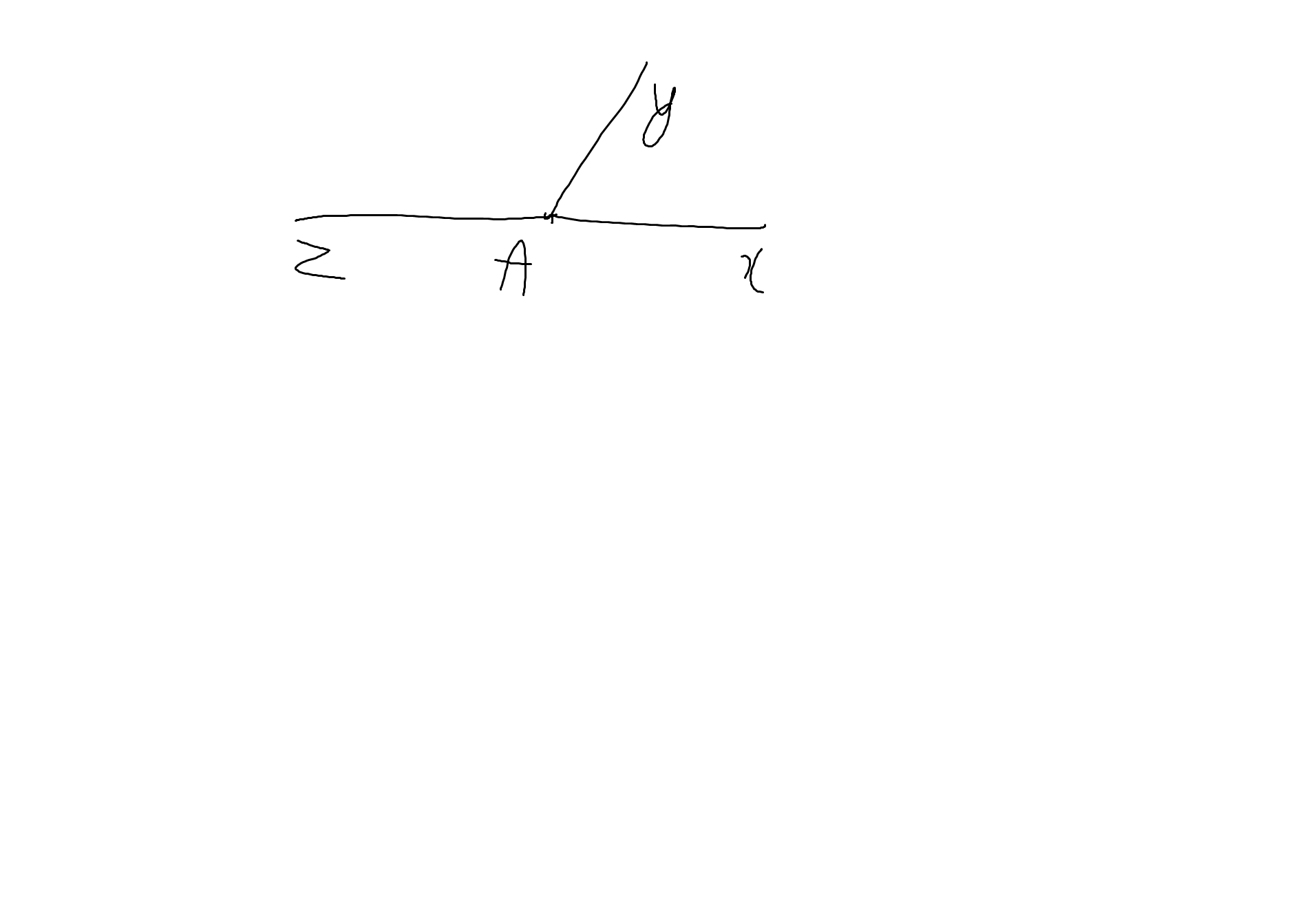
\(x\left(x+1\right)=2+4+6+...+2500\)

=>\(x\left(x+1\right)=2\cdot\left(1+2+3+...+1250\right)\)

=>\(x\left(x+1\right)=2\cdot\dfrac{1250\cdot1251}{2}=1250\cdot1251\)

=>x=1250

`x . (x + 1) = 2 + 4 +6 + ...+ 2500`

`=> x . (x + 1) = (2500 + 2) . [(2500 - 2) : 2 + 1] : 2`

`=> x . (x + 1) = 2502 . 1250 : 2`

`=> x . (x + 1) =1251. 1250`

`=> x . (x + 1) = (1250+1). 1250`

Do x là số nhiên, nên `x = 1250.`

Vậy `x = 1250 `

----------------------------

Phân tích thành nhân từ cũng được nhé:

`=> x . (x + 1) =1251. 1250`

`=> x^2 + x - 1251. 1250 = 0`

`=> x^2 + 1251x - 1250x - 1251. 1250 = 0`

`=> (x^2 + 1251x) - (1250x +  1251. 1250) = 0`

`=> x(x + 1251) - 1250 (x + 1251) = 0`

`=> (x-1250)(x+1251) = 0`

`=> x = 1250` hoặc `x = -1251`

Mà `x` là số tự nhiên nên `x = 1250`

+) 246a chia hết cho 2 

=> a ∈ {0; 2; 4; 6; 8} (1) 

+) 246a chia hết cho 3 

=> 2 + 4 + 6 + a = 12 + a chia hết cho 3 

12 ⋮ 3 => a ⋮ 3 => a ∈ {0; 3; 6; 9} (2) 

Từ (1) và (2) => a = 0 hoặc a = 6 

Để 246a là số lớn nhất thì a = 6 (vì 2460 < 2466) 

ABCD là hình chữ nhật 

=> CD = AB = 4 (cm) 

=> AD = BC = 3 (cm) 

=> BD = AC = 5 (cm)

1) 12 ⋮ x => x ∈ Ư(12) = {1; -1; 2; -2; 3; -3; 4; -4; 6; -6; 12; -12} 

Mà: x > 2 

=> x ∈ {3; 4; 6; 12} 

2) 24 ⋮ x => x ∈ Ư(24) = {1; -1; 2; -2; 3; -3; 4; -4; 6; -6; 8; -8; 12; -12; 24; -24} 

Mà: x > 4 

=> x ∈ {6; 8; 12; 24} 

3) 36 ⋮ x => x ∈ Ư(36) = {1; -1; 2; -2; 3; -3; 4; -4; 6; -6; 9; -9; 12; -12; 18; -18; 36; -36}

Mà: x ≥ 3 

=> x ∈ {3; 4; 6; 9; 12; 18; 36} 

4) 40 ⋮ x => x ∈ Ư(40) = {1; -1; 2; -2; 4; -4; 5; -5; 8; -8; 10; -10; 20; -20; 40; -40} 

Mà: x < 10 và x là số tự nhiên

=> x ∈ {1; 2; 4; 8}

Bài 1:

\(a.A=23,12+45,56+76,88+54,44\\ =\left(23,12+76,88\right)+\left(45,56+54,44\right)\\ =100+100\\ =200\\ b.201,5\cdot9+201,5\cdot2-201,5\\ =201,5\cdot\left(9+2-1\right)\\ =201,5\cdot10\\ =2015\\ c.C=\dfrac{1}{2}:0,5+\dfrac{1}{4}:0,25-\dfrac{1}{8}:0,125+2014\\ =\dfrac{1}{2}\cdot2+\dfrac{1}{4}\cdot4+\dfrac{1}{8}\cdot8+2014\\ =1+1+1+2014\\ =2017\\ d.D=2\dfrac{2}{3}+\dfrac{5}{9}+\dfrac{4}{9}:\left(30\%-\dfrac{1}{10}\right)-\dfrac{2}{9}\\ =\dfrac{8}{3}+\left(\dfrac{5}{9}-\dfrac{2}{9}\right)+\dfrac{4}{9}:\left(\dfrac{3}{10}-\dfrac{1}{10}\right)\\ =\dfrac{8}{3}+\dfrac{1}{3}+\dfrac{4}{9}:\dfrac{1}{5}\\ =\dfrac{9}{3}+\dfrac{4}{45}\\ =3+\dfrac{4}{9}\cdot5=3+\dfrac{20}{9}=\dfrac{47}{9}\)

Bài 2: 

a: y+2=2017

=>y=2017-2=2015

b: \(3y-2\dfrac{2}{7}=3\dfrac{5}{7}\)

=>\(3y=3+\dfrac{5}{7}+2+\dfrac{2}{7}=6\)

=>\(y=\dfrac{6}{3}=2\)

c: \(1\dfrac{3}{4}-\dfrac{3}{4}y=75\%\)

=>\(\dfrac{7}{4}-\dfrac{3}{4}y=\dfrac{3}{4}\)

=>7-3y=3

=>3y=7-3=4

=>\(y=\dfrac{4}{3}\)

d: \(\dfrac{2}{3}+\dfrac{1}{3}y+3\dfrac{2}{3}y=\dfrac{8}{3}\)

=>\(4y=\dfrac{8}{3}-\dfrac{2}{3}=\dfrac{6}{3}=2\)

=>\(y=\dfrac{2}{4}=\dfrac{1}{2}\)

e: \(y-14=25\)

=>y=25+14=39

f: \(5y-25=35\)

=>5y=25+35=60

=>y=60/5=12

g: 9,34-y=1,28

=>y=9,34-1,28=8,06

h: y:1,2=2,4

=>\(y=2,4\cdot1,2=2,88\)

i: 2,4:y=0,2

=>y=2,4:0,2=12

k: (y+1)+(y+3)=24

=>y+1+y+3=24

=>2y=20

=>y=10

Nam đếm được 129 cái gì.

\(S=\dfrac{1}{2}+\dfrac{1}{2^2}+\dfrac{1}{2^3}+...+\dfrac{1}{2^{200}}\)

\(\Rightarrow2S=2\left(\dfrac{1}{2}+\dfrac{1}{2^2}+\dfrac{1}{2^3}+...+\dfrac{1}{2^{200}}\right)\)

\(\Rightarrow2S=1+\dfrac{1}{2}+\dfrac{1}{2^2}+...+\dfrac{1}{2^{199}}\)

\(\Rightarrow2S-S=\left(1+\dfrac{1}{2}+\dfrac{1}{2^2}+...+\dfrac{1}{2^{199}}\right)-\left(\dfrac{1}{2}+\dfrac{1}{2^2}+\dfrac{1}{2^3}+...+\dfrac{1}{2^{200}}\right)\)

\(\Rightarrow S=1-\dfrac{1}{2^{100}}< 1\)

\(\Rightarrow S< 1\)

Vậy \(S< 1\)

Bài 18:

a: \(\left(-\dfrac{40}{51}\cdot0,32\cdot\dfrac{17}{20}\right):\dfrac{64}{75}\)

\(=-\dfrac{40}{20}\cdot\dfrac{17}{51}\cdot\dfrac{8}{25}\cdot\dfrac{75}{64}\)

\(=-\dfrac{2}{3}\cdot\dfrac{8}{64}\cdot\dfrac{75}{25}=-\dfrac{2}{3}\cdot3\cdot\dfrac{1}{8}=-\dfrac{2}{8}=-\dfrac{1}{4}\)

b: \(-\dfrac{10}{11}\cdot\dfrac{8}{9}+\dfrac{7}{18}\cdot\dfrac{10}{11}=\dfrac{10}{11}\left(-\dfrac{8}{9}+\dfrac{7}{18}\right)\)

\(=\dfrac{10}{11}\left(-\dfrac{16}{18}+\dfrac{7}{18}\right)=\dfrac{10}{11}\cdot\dfrac{-9}{18}=\dfrac{10}{11}\cdot\dfrac{-1}{2}=-\dfrac{5}{11}\)

c: \(\dfrac{3}{14}:\dfrac{1}{28}-\dfrac{13}{21}:\dfrac{1}{28}+\dfrac{29}{42}:\dfrac{1}{28}-8\)

\(=\left(\dfrac{3}{14}-\dfrac{13}{21}+\dfrac{29}{42}\right):\dfrac{1}{28}-8\)

\(=\left(\dfrac{9}{42}-\dfrac{6}{42}+\dfrac{29}{42}\right):\dfrac{1}{28}-8\)

\(=\dfrac{32}{42}\cdot28-8=32\cdot\dfrac{2}{3}-8=\dfrac{64}{3}-\dfrac{24}{3}=\dfrac{40}{3}\)

d: \(-1\dfrac{5}{7}\cdot15+\dfrac{2}{7}\cdot\left(-15\right)+\left(-105\right)\left(\dfrac{2}{3}-\dfrac{4}{5}+\dfrac{1}{7}\right)\)

\(=-\dfrac{12}{7}\cdot15+\dfrac{2}{7}\cdot\left(-15\right)+\left(-105\right)\left(\dfrac{70}{105}-\dfrac{84}{105}+\dfrac{15}{105}\right)\)

\(=\dfrac{-180-30}{7}+\left(-105\right)\cdot\dfrac{1}{105}\)

=-30-1=-31

Bài 19:

a: \(A=7x-2x-\dfrac{2}{3}y+\dfrac{7}{9}y=5x+y\left(\dfrac{7}{9}-\dfrac{2}{3}\right)=5x+\dfrac{y}{9}\)

Khi x=-1/10;y=4,8 thì \(A=5\cdot\dfrac{-1}{10}+\dfrac{4.8}{9}\)

\(=-\dfrac{1}{2}+\dfrac{8}{15}=\dfrac{-15+16}{30}=\dfrac{1}{30}\)

b: \(B=x+\dfrac{0,2-0,375+\dfrac{5}{11}}{-0,3+\dfrac{9}{16}-\dfrac{15}{22}}\)

\(=x+\dfrac{\dfrac{2}{10}-\dfrac{6}{16}+\dfrac{10}{22}}{-\dfrac{3}{10}+\dfrac{9}{16}-\dfrac{15}{22}}=x+\dfrac{2\left(\dfrac{1}{10}-\dfrac{3}{16}+\dfrac{5}{22}\right)}{-3\left(\dfrac{1}{10}-\dfrac{3}{16}+\dfrac{5}{22}\right)}\)

\(=x-\dfrac{2}{3}\)

Khi x=-1/3 thì \(B=-\dfrac{1}{3}-\dfrac{2}{3}=-\dfrac{3}{3}=-1\)

1: \(x\in B\left(12\right)\)

=>\(x\in\left\{0;12;24;36;48;60;72;...\right\}\)

mà x<=70

nên \(x\in\left\{0;12;24;36;48;60\right\}\)

2: \(x\in B\left(8\right)\)

=>\(x\in\left\{0;8;16;24;32;40;48;56;64;...\right\}\)

mà 12<=x<=50

nên \(x\in\left\{16;24;32;40;48\right\}\)

3: \(x\in B\left(7\right)\)

=>\(x\in\left\{0;7;14;21;28;35;42;49;56;...\right\}\)

mà 16<x<56

nên \(x\in\left\{21;28;35;42;49\right\}\)

4: \(x\in B\left(5\right)\)

=>\(x\in\left\{0;5;10;15;20;25;30;35;40;...\right\}\)

mà 17<=x<=37

nên \(x\in\left\{20;25;30;35\right\}\)