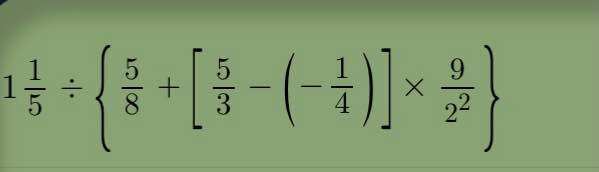Chứng minh rằng số có dạng n ^ 6 - n ^ 4 + 2n ^ 3 + 2n ^ 2, n \(\in\) N, n > 1 không phải số chính phương
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Nó còn tùy từng trường hợp cụ thể của đề bài chứ em?

ΔABC vuông tại A
=>\(AB^2+AC^2=BC^2\)
=>\(AC^2=12^2-5^2=144-25=119\)
=>\(AC=\sqrt{119}\left(cm\right)\)
a: Xét ΔABC vuông tại A có AH là đường cao
nên \(\left\{{}\begin{matrix}AB^2=BH\cdot BC\\AC^2=CH\cdot BC\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}BH=\dfrac{5^2}{12}=\dfrac{25}{12}\left(cm\right)\\CH=\dfrac{119}{12}\left(cm\right)\end{matrix}\right.\)
b: Xét ΔABC vuông tại A có AH là đường cao
nên \(AH^2=HB\cdot HC=\dfrac{25}{12}\cdot\dfrac{119}{12}=\dfrac{25}{144}\cdot119\)
=>\(AH=\sqrt{119}\cdot\sqrt{\dfrac{25}{144}}=\dfrac{5}{12}\cdot\sqrt{119}\left(cm\right)\)

Ta thấy
\(x+23⋮31\)
\(x+8⋮15\Rightarrow\left(x+8\right)+15=x+23⋮15\)
\(\Rightarrow\left(x+23\right)=BC\left(15;31\right)\) x nhỏ nhất khi \(x+23=BCNN\left(15;31\right)\)
\(\Rightarrow BCNN\left(15;31\right)=15x31=465\)
\(\Rightarrow x+23=465\Rightarrow x=442\)


A=104-100+96-92+88-84+...-12+8
=(104-100)+(96-92)+...+(16-12)+8
=4+4+...+4+8
\(=4\cdot12+8=48+8=56\)

`8 . 2^(x - 5) = 16^7`
`=> 2^3 . 2^(x - 5) = (2^4)^7`
`=> 2^(3 + x - 5) = 2^28`
`=> x - 2 = 28`
`=> x=28+2`
`=>x=30`
Vậy: `x=30`
\(8\cdot2^{x-5}=16^7\)
=>\(2^3\cdot2^{x-5}=2^{28}\)
=>\(2^{x-2}=2^{28}\)
=>x-2=28
=>x=2+28=30

a: ĐKXĐ: x>=1/2
\(\sqrt{2x-1}=5\)
=>\(2x-1=5^2=25\)
=>2x=26
=>x=13(nhận)
b: ĐKXĐ: \(x>=-\dfrac{2}{3}\)
\(\sqrt{3x+2}=\dfrac{1}{4}\)
=>\(3x+2=\left(\dfrac{1}{4}\right)^2=\dfrac{1}{16}\)
=>\(3x=\dfrac{1}{16}-2=\dfrac{1}{16}-\dfrac{32}{16}=-\dfrac{31}{16}\)
=>\(x=-\dfrac{31}{48}\left(nhận\right)\)
c: \(\sqrt{x^2+\dfrac{1}{4}}=\sqrt{\dfrac{49}{81}}\)
=>\(x^2+\dfrac{1}{4}=\dfrac{49}{81}\)
=>\(x^2=\dfrac{49}{81}-\dfrac{1}{4}=\dfrac{115}{324}\)
=>\(x=\pm\dfrac{\sqrt{115}}{18}\)

\(1\dfrac{1}{5}:\left\{\dfrac{5}{8}+\left[\dfrac{5}{3}-\left(-\dfrac{1}{4}\right)\right]\cdot\dfrac{9}{2^2}\right\}\)
\(=\dfrac{6}{5}:\left\{\dfrac{5}{8}+\left(\dfrac{5}{3}+\dfrac{1}{4}\right)\cdot\dfrac{9}{4}\right\}\)
\(=\dfrac{6}{5}:\left\{\dfrac{5}{8}+\dfrac{23}{12}\cdot\dfrac{9}{4}\right\}\)
\(=\dfrac{6}{5}:\left\{\dfrac{5}{8}+\dfrac{23\cdot3}{16}\right\}=\dfrac{6}{5}:\left(\dfrac{10}{16}+\dfrac{69}{16}\right)\)
\(=\dfrac{6}{5}\cdot\dfrac{16}{79}=\dfrac{96}{395}\)