Chứng minh rằng: (10a + 5)2 = 100a . a(a + 1) + 25
Từ đó hãy nêu cách tính nhẩm bình phương của một số tự nhiên có tận cùng bằng chữ số 5.
Áp dụng để tính: 252; 352; 652; 752
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

1. Ta có : 2x4 - 3x3 - 3x2 + 6x - 2
= 2x4 - 2x3 - x3 + x2 - 4x2 + 4x + 2x - 2
= 2x3( x - 1 ) - x2( x - 1 ) - 4x( x - 1 ) + 2( x - 1 )
= ( x - 1 )( 2x3 - x2 - 4x + 2 )
= ( x - 1 )[ x2( 2x - 1 ) - 2( 2x - 1 ) ]
= ( x - 1 )( 2x - 1 )( x2 - 2 )
=> ( 2x4 - 3x3 - 3x2 + 6x - 2 ) : ( x2 - 2 ) = ( x - 1 )( 2x - 1 ) = 2x2 - 3x + 1
2. \(\left(15x^4y^6-12x^3y^4-18x^2y^3\right)\div\left(-6x^2y^2\right)\)
\(=\frac{15x^4y^6}{-6x^2y^2}-\frac{12x^3y^4}{-6x^2y^2}-\frac{18x^2y^3}{-6x^2y^2}\)
\(=-\frac{5}{2}x^2y^4+2xy^2+3y\)

a) \(\frac{1}{3}x^2y+\frac{1}{6}xy^2-\frac{1}{9}xy\)
\(=xy\left(\frac{1}{3}x+\frac{1}{6}y-\frac{1}{9}\right)\)
b) \(a^3+3a^2+3a-7\)
\(=\left(a^3+3a^2+3a+1\right)-8\)
\(=\left(a+1\right)^3-2^3\)
\(=\left(a+1-2\right)\left[\left(a+1\right)^2+2\left(a+1\right)+2^2\right]\)
\(=\left(a-1\right)\left(a^2+2a+1+2a+2+4\right)\)
\(=\left(a-1\right)\left(a^2+4a+7\right)\)
c) \(2x\left(2x-1\right)-2x^2\)
\(=4x^2-2x-2x^2\)
\(=2x^2-2x=2x\left(x-1\right)\)

Áp dụng định lí 1 đường trung bình của tam giác
ΔADC có E là trung điểm AD và EI song song với cạnh DC
⇒ Điểm I là trung điểm AC
ΔABC có I là trung điểm AC và FI song song với cạnh AB
⇒ điểm F là trung điểm BC

a) \(\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)-8\)
\(=\left[\left(x+1\right)\left(x+4\right)\right]\left[\left(x+2\right)\left(x+3\right)\right]-8\)
\(=\left(x^2+5x+4\right)\left(x^2+5x+6\right)-8\)
\(=\left(x^2+5x+5\right)^2-1-8\)
\(=\left(x^2+5x+5\right)^2-3^2\)
\(=\left(x^2+5x+2\right)\left(x^2+5x+8\right)\)
b) \(xy\left(x-y\right)+yz\left(y-z\right)+zx\left(z-x\right)\)
\(=xy\left(x-y\right)+y^2z-yz^2+z^2x-zx^2\)
\(=xy\left(x-y\right)+z^2\left(x-y\right)-z\left(x-y\right)\left(x+y\right)\)
\(=\left(x-y\right)\left(xy+z^2-zx-yz\right)\)
\(=\left(x-y\right)\left[x\left(y-z\right)-z\left(y-z\right)\right]\)
\(=\left(x-y\right)\left(x-z\right)\left(y-z\right)\)
a) ( x + 1 )( x + 2 )( x + 3 )( x + 4 ) - 8
= [ ( x + 1 )( x + 4 ) ][ ( x + 2 )( x + 3 ) ] - 8
= ( x2 + 5x + 4 )( x2 + 5x + 6 ) - 8
Đặt t = x2 + 5x + 5
bthuc ⇔ ( t - 1 )( t + 1 ) - 8
= t2 - 1 - 8
= t2 - 9
= ( t - 3 )( t + 3 )
= ( x2 + 5x + 5 - 3 )( x2 + 5x + 5 + 3 )
= ( x2 + 5x + 2 )( x2 + 5x + 8 )
b) xy( x - y ) + yz( y - z ) + zx( z - x )
= x2y - xy2 + y2z - yz2 + zx( z - x )
= ( y2z - xy2 ) - ( yz2 - x2y ) + zx( z - x )
= y2( z - x ) - y( z2 - x2 ) + zx( z - x )
= ( z - x )( y2 + zx ) - y( z - x )( z + x )
= ( z - x )( y2 + zx - yz - yx )
= ( z - x )[ ( y2 - yx ) - ( yz - zx ) ]
= ( z - x )[ y( y - x ) - z( y - x ) ]
= ( z - x )( y - x )( y - z )

Hẳn là PTĐTTNT rồi!
1) \(x^2-9y^2+6y-1\)
\(=x^2-\left(9y^2-6y+1\right)\)
\(=x^2-\left(3y-1\right)^2\)
\(=\left(x-3y+1\right)\left(x+3y-1\right)\)
2) \(x^2-y^2-4x+4y\) (chắc vậy mới đúng)
\(=\left(x-y\right)\left(x+y\right)-4\left(x-y\right)\)
\(=\left(x-y\right)\left(x+y-4\right)\)
3) \(2xy-x^2-y^2+9\)
\(=9-\left(x^2-2xy+y^2\right)\)
\(=9-\left(x-y\right)^2\)
\(=\left(3-x+y\right)\left(3+x-y\right)\)
4) \(x^2-9=\left(x-3\right)\left(x+3\right)\)
5) \(x^3-27=\left(x-3\right)\left(x^2+3x+9\right)\)
6) \(8x^3+1=\left(2x+1\right)\left(4x^2-2x+1\right)\)
7) \(2xy-x^2-y^2+9\) (đã sửa đề)
\(=9-\left(x^2-2xy+y^2\right)\)
\(=9-\left(x-y\right)^2\)
\(=\left(3-x+y\right)\left(3+x-y\right)\) giống câu 3

a) \(3x\left(x-4\right)+15=3x^2\)
\(\Leftrightarrow3x^2-12x+15-3x^2=0\)
\(\Leftrightarrow-12x+15=0\)
\(\Leftrightarrow x=\frac{5}{4}\)
b) \(x^2+y^2-2x+8y+17=0\)
\(\Leftrightarrow\left(x^2-2x+1\right)+\left(y^2+8y+16\right)=0\)
\(\Leftrightarrow\left(x-1\right)^2+\left(y+1\right)^2=0\)
Mà \(\hept{\begin{cases}\left(x-1\right)^2\ge0\\\left(y+1\right)^2\ge0\end{cases}}\)
\(\Rightarrow\hept{\begin{cases}x-1=0\\y+1=0\end{cases}\Leftrightarrow\hept{\begin{cases}x=1\\y=-1\end{cases}}}\)

a) \(x^2-5x+5y-y^2\)
\(=\left(x-y\right)\left(x+y\right)-5\left(x-y\right)\)
\(=\left(x-y\right)\left(x+y-5\right)\)
b) \(2\left(x^2+1\right)^2-8x^2\)
\(=2\left[\left(x^2+1\right)^2-4x^2\right]\)
\(=2\left(x^2-2x+1\right)\left(x^2+2x+1\right)\)
\(=2\left(x-1\right)^2\left(x+1\right)^2\)
Ta có:
(10a + 5)2 = (10a)2 + 2.10a.5 + 52
= 100a2 + 100a + 25
= 100a(a + 1) + 25
Đặt A = a.(a + 1). Khi đó ta có:
Do vậy, để tính bình phương của một số tự nhiên có dạng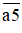 , ta chỉ cần tính tích a.(a + 1) rồi viết 25 vào đằng sau kết quả vừa tìm được.
, ta chỉ cần tính tích a.(a + 1) rồi viết 25 vào đằng sau kết quả vừa tìm được.