Đề là so sánh phân số mới đúng nhé bạn!

a)

Ta có: 

Mẫu số chung 2 phân số: 12

$\frac{-3}{4}=\frac{-3\cdot3}{4\cdot3}=\frac{-9}{12}$

$\frac{-2}{3}=\frac{-2\cdot4}{3\cdot4}=\frac{-8}{12}$

Vì $-9<-8$ nên$\frac{-9}{12}<\frac{-8}{12}$

Vậy $\frac{-3}{4}<\frac{-2}{3}$

b)

$\frac{24}{-60}=\frac{24:-12}{-60:-12}=\frac{-2}{5}$

$\frac{-33}{44}=\frac{-33:11}{44:11}=\frac{-3}{4}$

Ta có: 

Mẫu số chung 2 phân số: 20

$\frac{-2}{5}=\frac{-2\cdot4}{5\cdot4}=\frac{-8}{20}$

$\frac{-3}{4}=\frac{-3\cdot5}{4\cdot5}=\frac{-15}{20}$

Vì $-8>-15$ nên$\frac{-8}{20}>\frac{-15}{20}$

Vậy $\frac{24}{-60}>\frac{-33}{44}$

c)

$\frac{-75}{85}=\frac{-75:5}{85:5}=\frac{-15}{17}$

$\frac{34}{-68}=\frac{34:-34}{-68:-34}=\frac{-1}{2}$

Ta có: 

Mẫu số chung 2 phân số: 34

$\frac{-15}{17}=\frac{-15\cdot2}{17\cdot2}=\frac{-30}{34}$

$\frac{-1}{2}=\frac{-1\cdot17}{2\cdot17}=\frac{-17}{34}$

Vì $-30<-17$ nên$\frac{-30}{34}<\frac{-17}{34}$

Vậy $\frac{-75}{85}<\frac{34}{-68}$

Tính gì vậy bạn?

\(-3\left(x^2+5\right)-\left(x+1\right)\left(3x-9\right)=3x+10\)

=>\(-3x^2-15-3x^2+9x-3x+9=3x+10\)

=>\(-6x^2+6x-6-3x-10=0\)

=>\(-6x^2+3x-16=0\)

\(\text{Δ}=3^2-4\cdot\left(-6\right)\left(-16\right)=9-4\cdot96=-375< 0\)

=>Phương trình vô nghiệm

-3(x² + 5) - (x + 1)(3x - 9) = 2x + 10

-3x² - 15 - 3x² + 9x - 3x + 9 = 2x + 10

-6x² + 6x - 6 = 2x + 10

-6x² + 6x - 6 - 2x - 10 = 0

-6x² + 4x - 16 = 0

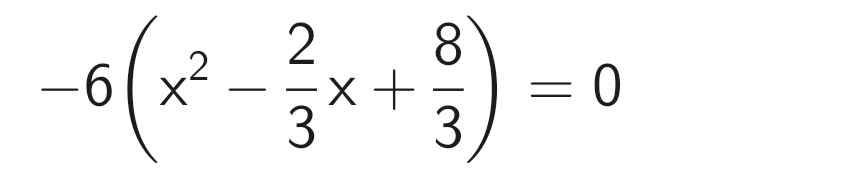  Ta có:

x² - 2/3 x + 8/3

= x² - 2.x.1/3 + (1/3)² + 23/9

= (x - 1/3)² + 23/9 > 0 với mọi x ∈ R

⇒ -6[(x - 1/3)² + 23/9] < 0 với mọi x ∈ R

Vậy không tìm được x thỏa mãn đề bài

a: Vì \(\widehat{xOy};\widehat{zOy}\) là hai góc kề nhau nên tia Oy nằm giữa hai tia Ox,Oz

=>\(\widehat{xOy}+\widehat{zOy}=\widehat{xOz}=120^0\)

mà \(\widehat{xOy}-\widehat{zOy}=40^0\)

nên \(\widehat{xOy}=\dfrac{120^0+40^0}{2}=80^0;\widehat{zOy}=80^0-40^0=40^0\)

b: Ta có: \(\widehat{xOm}+\widehat{xOy}=180^0\)(hai góc kề bù)

=>\(\widehat{xOm}+80^0=180^0\)

=>\(\widehat{xOm}=100^0\)

c: Ta có: \(\widehat{mOn}=\widehat{yOz}\)(hai góc đối đỉnh)

mà \(\widehat{yOz}=40^0\)

nên \(\widehat{mOn}=40^0\)

a) Ta có:

\(\widehat{xOy}+\widehat{zOy}=\widehat{xOz}=120^o\) (hai góc kề nhau)

Mà \(\widehat{xOy}-\widehat{zOy}=40^o\) nên:

\(\widehat{xOy}=\dfrac{120^o+40^o}{2}=80^o\)

Do đó: \(\widehat{zOy}=120^o-80^o=40^o\)

Vậy...

b) Ta có:

\(\widehat{xOy}+\widehat{xOm}=180^o\) (hai góc kề bù)

Mà \(\widehat{xOy}=80^o\) nên:

\(\widehat{xOm}=180^o-80^o=100^o\)

Vậy...

c) Ta có:

\(\widehat{mOn}=\widehat{zOy}\) (hai góc đối đỉnh)

Mà \(\widehat{zOy}=40^o\) nên:

\(\widehat{mOn}=40^o\)

Vậy...

a: \(\dfrac{1}{2}< \dfrac{12}{a}< \dfrac{4}{3}\)

=>\(\dfrac{12}{24}< \dfrac{12}{a}< \dfrac{12}{9}\)

=>9<a<24

mà a nguyên

nên \(a\in\left\{10;11;...;23\right\}\)

b: \(\dfrac{7}{4}< \dfrac{a}{8}< 3\)

=>\(\dfrac{14}{8}< \dfrac{a}{8}< \dfrac{24}{8}\)

=>14<a<24

mà a nguyên

nên \(a\in\left\{15;16;...;23\right\}\)

c: \(\dfrac{2}{3}< \dfrac{a-1}{6}< \dfrac{8}{9}\)

=>\(\dfrac{12}{18}< \dfrac{3\left(a-1\right)}{18}< \dfrac{16}{18}\)

=>12<3a-3<16

=>15<3a<19

=>5<a<19/3

mà a nguyên

nên a=6

d: \(\dfrac{12}{9}< \dfrac{4}{a}< \dfrac{8}{3}\)

=>\(\dfrac{8}{6}< \dfrac{8}{2a}< \dfrac{8}{3}\)

=>3<2a<6

mà a nguyên

nên 2a=4

=>a=2

\(\dfrac{1}{21}\) = \(\dfrac{1\times3}{21\times3}\) = \(\dfrac{3}{63}\) < \(\dfrac{3}{27}\)

Vậy \(\dfrac{1}{21}\) < \(\dfrac{3}{27}\)

a;  \(\dfrac{4}{27}\) = \(\dfrac{4\times7}{27\times7}\) = \(\dfrac{28}{189}\)

     \(\dfrac{15}{63}\) = \(\dfrac{15\times3}{63\times3}\) = \(\dfrac{45}{189}\)

     \(\dfrac{28}{189}\) < \(\dfrac{45}{189}\)

     - \(\dfrac{28}{189}\) > - \(\dfrac{45}{189}\)

Vậy - \(\dfrac{4}{27}\) > \(\dfrac{15}{-63}\) 

b;   \(\dfrac{13}{15}\) = \(\dfrac{13\times9}{15\times9}\) =  \(\dfrac{117}{135}\)

     \(\dfrac{9}{11}\) = \(\dfrac{9\times13}{11\times13}\) = \(\dfrac{117}{143}\)

     \(\dfrac{117}{135}\) > \(\dfrac{117}{143}\) 

Vậy \(\dfrac{13}{15}\) > \(\dfrac{9}{11}\) 

\(\frac{x+4}{2000}+\frac{x+3}{2001}=\frac{x+2}{2002}+\frac{x+1}{2003}\\\Leftrightarrow \frac{x+4}{2000}+\frac{x+3}{2001}-\frac{x+2}{2002}-\frac{x+1}{2003}=0\\\Leftrightarrow \left(\frac{x+4}{2000}+1\right)+\left(\frac{x+3}{2001}+1\right)-\left(\frac{x+2}{2002}+1\right)-\left(\frac{x+1}{2003}+1\right)=0\\\Leftrightarrow \frac{x+2004}{2000}+\frac{x+2004}{2001}-\frac{x+2004}{2002}-\frac{x+2004}{2003}=0\\\Leftrightarrow (x+2024)\left(\frac{1}{2000}+\frac{1}{2001}-\frac{1}{2002}-\frac{1}{2003}\right)=0\\\Leftrightarrow x+2024=0(\text{vì }\frac{1}{2000}+\frac{1}{2001}-\frac{1}{2002}-\frac{1}{2003} \ne0)\\\Leftrightarrow x=-2024\)

Vậy phương trình có 1 nghiệm duy nhất là $x=-2024$.

a: \(-\dfrac{19}{49}=\dfrac{-19\cdot47}{49\cdot47}=\dfrac{-893}{2303}\)

\(\dfrac{-23}{47}=\dfrac{-23\cdot49}{47\cdot49}=\dfrac{-1127}{2303}\)

mà -893>-1127

nên \(-\dfrac{19}{49}>-\dfrac{23}{47}\)

b: \(\dfrac{-5}{8}=\dfrac{-5\cdot5}{8\cdot5}=\dfrac{-25}{40}\)

\(\dfrac{7}{-10}=\dfrac{-7}{10}=\dfrac{-7\cdot4}{10\cdot4}=\dfrac{-28}{40}\)

mà -25>-28

nên \(-\dfrac{5}{8}>\dfrac{7}{-10}\)

c: \(\dfrac{24}{35}=\dfrac{24\cdot6}{35\cdot6}=\dfrac{144}{210};\dfrac{19}{30}=\dfrac{19\cdot7}{30\cdot7}=\dfrac{133}{210}\)

mà 144>133

nên \(\dfrac{24}{35}>\dfrac{19}{30}\)

\(-1,25=-\dfrac{125}{100}=-\dfrac{5}{4}\)