Giúp với ạ 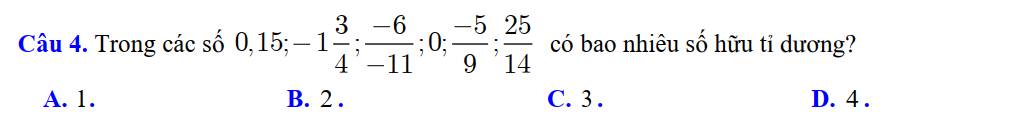
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



-3^7.2^8/2^.3^7
=-3.2
=-6
5^3.3^5/5^3(0,5+2,5)
=5^3.3^5/5^3.3\
3^4
=81
5.7^4+7^3.25/7^5.125-7^3.50
=5.7^3(7+5
5.7^4+7^3.25/7^5.125-7^3.50
=5.7^4+7^3.5^2/7^5.5^3-7^3.11.5
=5.7^3(1.7+1.5)/7^3.5(7^2.25-11)
12/1250


Kéo dài tia AO và đặt là Ax. Khi đó:
\(\widehat{BOC}=\widehat{BOx}+\widehat{COx}\)
Xét tam giác OAB có \(\widehat{BOx}\) là góc ngoài tại O nên
\(\widehat{BOx}=\widehat{A_1}+\widehat{ABO}\) (1)
Tương tự, ta có \(\widehat{COx}=\widehat{A_2}+\widehat{ACO}\) (2)
Cộng theo vế (1) và (2), ta được:
\(\widehat{BOC}=\widehat{A_1}+\widehat{A_2}+\widehat{ABO}+\widehat{ACO}\)
\(=\widehat{A}+\widehat{ABO}+\widehat{ACO}\)
Ta có đpcm.

Cho \(A=\left(-\dfrac{4}{7}\right)^3\times\left(-\dfrac{4}{7}\right)^4\times\left(\dfrac{4}{7}\right)^5\)
\(A=\left(-\dfrac{4}{7}\right)^{3+4}\times\left(\dfrac{4}{7}\right)^5\)
\(A=\left(-\dfrac{4}{7}\right)^7\times\left(\dfrac{4}{7}\right)^5\)
\(-A=-\left[\left(-\dfrac{4}{7}\right)^7\times\left(\dfrac{4}{7}\right)^5\right]\)
\(-A=\left(-\dfrac{4}{7}\right)^7\times\left(-\dfrac{4}{7}\right)^5\)
\(-A=\left(-\dfrac{4}{7}\right)^{7+5}\)
\(-A=\left(-\dfrac{4}{7}\right)^{12}\)
\(A=-\left(\dfrac{4}{7}\right)^{12}\)

A = \(\dfrac{1+5+5^2+5^3+5^4+...+5^{17}}{1+5^2+5^4+...+5^{16}}\)
Đặt tử số là B = 1 + 5 + 52 + 53 + 54 +...+ 517
5B = 5 + 52 + 53 + 54 +...+ 517 + 518
5B - B = 518 - 1
4B = 518 - 1
B = (518 - 1) : 4
Đặt mẫu số là C = 1 + 52 + 54 +...+ 516
52.C = 52 + 54 +...+ 516 + 518
25.C - C = 518 - 1
24C = 518 - 1
C = (518 - 1): 24
A = \(\dfrac{B}{C}\) = \(\dfrac{\dfrac{5^{18}-1}{4}}{\dfrac{5^{18}-1}{24}}\)
A = 6

\(\Leftrightarrow x^2-xy-5x+4y+9=0\)
\(\Leftrightarrow\left(x^2-xy\right)-\left(4x-4y\right)-x+9=0\)
\(\Leftrightarrow x\left(x-y\right)-4\left(x-y\right)-x+9=0\)
\(\Leftrightarrow\left(x-y\right)\left(x-4\right)-\left(x-4\right)+5=0\)
\(\Leftrightarrow\left(x-4\right)\left(x-y-1\right)=-5\)
Do \(x;y\in Z\Rightarrow\left(x-4\right);\left(x-y-1\right)\in Z\)
Ta có các trường hợp sau
+ TH1:
\(\left\{{}\begin{matrix}x-4=1\\x-y-1=-5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=5\\y=9\end{matrix}\right.\)
+ TH2:
\(\left\{{}\begin{matrix}x-4=-1\\x-y-1=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=-3\end{matrix}\right.\)
+ TH3:
\(\left\{{}\begin{matrix}x-4=5\\x-y-1=-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=9\\y=9\end{matrix}\right.\)
+ TH4:
\(\left\{{}\begin{matrix}x-4=-5\\x-y-1=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-1\\y=-3\end{matrix}\right.\)
Có 3 số hữu tỉ dương là \(0,15;\dfrac{-6}{-11}=\dfrac{6}{11};\dfrac{25}{14}\)
Chọn C