cứu mik với, mik cần gấp
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 4: \(8^{10}\cdot125^{10}< =2^n\cdot5^n< =20^{16}\cdot5^{16}\)
=>\(1000^{10}< =10^n< =100^{16}\)
=>\(10^{30}< =10^n< =10^{32}\)
=>30<=n<=32
mà n là số tự nhiên
nên \(n\in\left\{30;31;32\right\}\)
Bài 1:
1: \(3^{-2}\cdot3^4\cdot3^n=3^7\)
=>\(3^n\cdot3^2=3^7\)
=>n+2=7
=>n=7-2=5
2: \(2^{-1}\cdot2^n+4\cdot2^n=9\cdot2^5\)
=>\(2^n\left(\dfrac{1}{2}+4\right)=2^5\cdot9\)
=>\(2^n=9\cdot2^5:\dfrac{9}{2}=2^6\)
=>n=6
Bài 2:
1: \(243>=3^n>=9\)
=>\(3^2< =3^n< =3^5\)
=>2<=n<=5
mà n là số tự nhiên
nên \(n\in\left\{2;3;4;5\right\}\)
2: \(2^{n+3}\cdot2^n=144\)
=>\(2^{2n+3}=144\)
=>\(2n+3=log_2144\)
=>\(2n=log_2144-3\)
=>\(n=\dfrac{log_2144-3}{2}\left(loại\right)\)
Bài 3:
\(10^x:5^y=20^y\)
=>\(10^x=20^y\cdot5^y=100^y=10^{2y}\)
=>x=2y
vậy: \(\left(x;y\right)\in\){(2k;k)|\(k\in N\)}

a: Ta có: \(\widehat{ICA}+\widehat{ICB}=\widehat{ACB}=90^0\)
\(\widehat{ICB}+\widehat{NCB}=\widehat{NCI}=90^0\)
Do đó: \(\widehat{ICA}=\widehat{NCB}\)
Ta có: \(\widehat{CAI}+\widehat{CBI}=90^0\)(ΔCBA vuông tại C)
\(\widehat{CBI}+\widehat{CBN}=\widehat{NBI}=90^0\)
Do đó: \(\widehat{CAI}=\widehat{CBN}\)
Xét ΔCAI và ΔCBN có
\(\widehat{CAI}=\widehat{CBN}\)
\(\widehat{ICA}=\widehat{NCB}\)
Do đó: ΔCAI~ΔCBN
b: Ta có: \(\widehat{ACM}+\widehat{ACI}=\widehat{ICM}=90^0\)
\(\widehat{ICA}+\widehat{ICB}=\widehat{ACB}=90^0\)
Do đó: \(\widehat{ACM}=\widehat{ICB}\)
Ta có: \(\widehat{CAM}+\widehat{CAB}=\widehat{BAM}=90^0\)
\(\widehat{CAB}+\widehat{CBA}=90^0\)(ΔCAB vuông tại C)
Do đó: \(\widehat{CAM}=\widehat{CBA}\)
Xét ΔCAM và ΔCBI có
\(\widehat{CAM}=\widehat{CBI}\)
\(\widehat{ACM}=\widehat{BCI}\)
Do đó: ΔCAM~ΔCBI
=>\(\dfrac{AC}{CB}=\dfrac{AM}{BI}\)
=>\(AC\cdot BI=MA\cdot BC\)
c: Xét tứ giác CIBN có \(\widehat{ICN}+\widehat{IBN}=90^0+90^0=180^0\)
nên CIBN là tứ giác nội tiếp
=>\(\widehat{CIN}=\widehat{CBN}\)
=>\(\widehat{CIN}=\widehat{BAC}\)

a: \(\left(x+2\right)^2-\left(x-2\right)\left(x+1\right)=3\)
=>\(x^2+4x+4-\left(x^2-x-2\right)=3\)
=>\(x^2+4x+4-x^2+x+2-3=0\)
=>5x+3=0
=>5x=-3
=>\(x=-\dfrac{3}{5}\)
b: \(\left(2x+3\right)^2-4\left(x-1\right)^2=0\)
=>\(\left(2x+3\right)^2-\left(2x-2\right)^2=0\)
=>\(\left(2x+3+2x-2\right)\left(2x+3-2x+2\right)=0\)
=>\(5\left(4x+1\right)=0\)
=>4x+1=0
=>4x=-1
=>\(x=-\dfrac{1}{4}\)
c: \(\left(x+1\right)\left(x^2-x+1\right)-x\left(x^2+2\right)-2=0\)
=>\(x^3+1-x^3-2x-2=0\)
=>-2x-1=0
=>-2x=1
=>\(x=\dfrac{1}{-2}=-\dfrac{1}{2}\)

b: Chọn mp(SAC) có chứa SC
\(I\in SA\subset\left(SAC\right);I\in\left(BIK\right)\)
Do đó: \(I\in\left(SAC\right)\cap\left(BIK\right)\)
Trong mp(ABCD), gọi H là giao điểm của AC và BK
=>\(H\in\left(SAC\right)\cap\left(BIK\right)\)
=>\(\left(SAC\right)\cap\left(BIK\right)=HI\)
Gọi M là giao điểm của HI với SC
=>M là giao điểm của SC với mp(BIK)

Gọi phân số cần tìm có dạng là \(\dfrac{a}{b}\)
Nếu thêm 2 đơn vị vào tử số thì phân số mới có giá trị bằng 1 nên \(\dfrac{a+2}{b}=1\)
=>a+2=b
=>b=a+2
Nếu chuyển 5 đơn vị từ tử số xuống phân số thì phân số mới có giá trị là 1/2 nên \(\dfrac{a-5}{b+5}=\dfrac{1}{2}\)
=>2(a-5)=b+5
=>2a-10=a+7
=>2a-a=10+7
=>a=17
=>b=17+2=19
vậy: Phân số cần tìm là \(\dfrac{17}{19}\)

Gọi độ dài mảnh đất ban đầu là x(m)
(Điều kiện: x>0)
Chiều dài mảnh đất mới là x+8(m)
Chiều rộng mảnh đất mới là x+4(m)
Diện tích mảnh đất đó tăng thêm 360m2 nên ta có:
\(\left(x+8\right)\left(x+4\right)-x^2=360\)
=>\(x^2+12x+32-x^2=360\)
=>12x=360-32=328
=>\(x=\dfrac{328}{12}=\dfrac{82}{3}\left(nhận\right)\)
Diện tích mảnh đất ban đầu là \(\left(\dfrac{82}{3}\right)^2=\dfrac{6724}{9}\left(m^2\right)\)

a: \(\left\{{}\begin{matrix}2\left(x+y\right)+3\left(x-y\right)=4\\x+y+2\left(x-y\right)=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x+2y+3x-3y=4\\x+y+2x-2y=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}5x-y=4\\3x-y=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}5x-y-3x+y=4-5\\3x-y=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2x=-1\\y=3x-5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-\dfrac{1}{2}\\y=3\cdot\dfrac{-1}{2}-5=-\dfrac{3}{2}-5=-\dfrac{13}{2}\end{matrix}\right.\)
b: \(\left\{{}\begin{matrix}\left(x+1\right)\left(y-1\right)=xy-1\\\left(x-3\right)\left(y+3\right)=xy-3\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}xy-x+y-1=xy-1\\xy+3x-3y-9=xy-3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-x+y=0\\3x-3y=6\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x-y=0\\x-y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x-y-x+y=0-2\\x-y=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}0y=-2\\x-y=0\end{matrix}\right.\Leftrightarrow\left(x;y\right)\in\varnothing\)

ΔABC=ΔDEF
=>\(\widehat{A}=\widehat{D}\)
=>\(\widehat{D}=55^0\)
ΔABC=ΔDEF
=>\(\widehat{B}=\widehat{E}\)
=>\(\widehat{B}=75^0\)
Xét ΔABC có \(\widehat{A}+\widehat{B}+\widehat{C}=180^0\)
=>\(\widehat{C}=180^0-55^0-75^0=50^0\)
=>\(\widehat{F}=\widehat{C}=50^0\)

\(\dfrac{11}{3}+\left|x\right|=\dfrac{9}{4}\)
=>\(\left|x\right|=\dfrac{9}{4}-\dfrac{11}{3}=\dfrac{27}{12}-\dfrac{44}{12}=-\dfrac{17}{12}\)
mà \(\left|x\right|>=0\forall x\)
nên \(x\in\varnothing\)
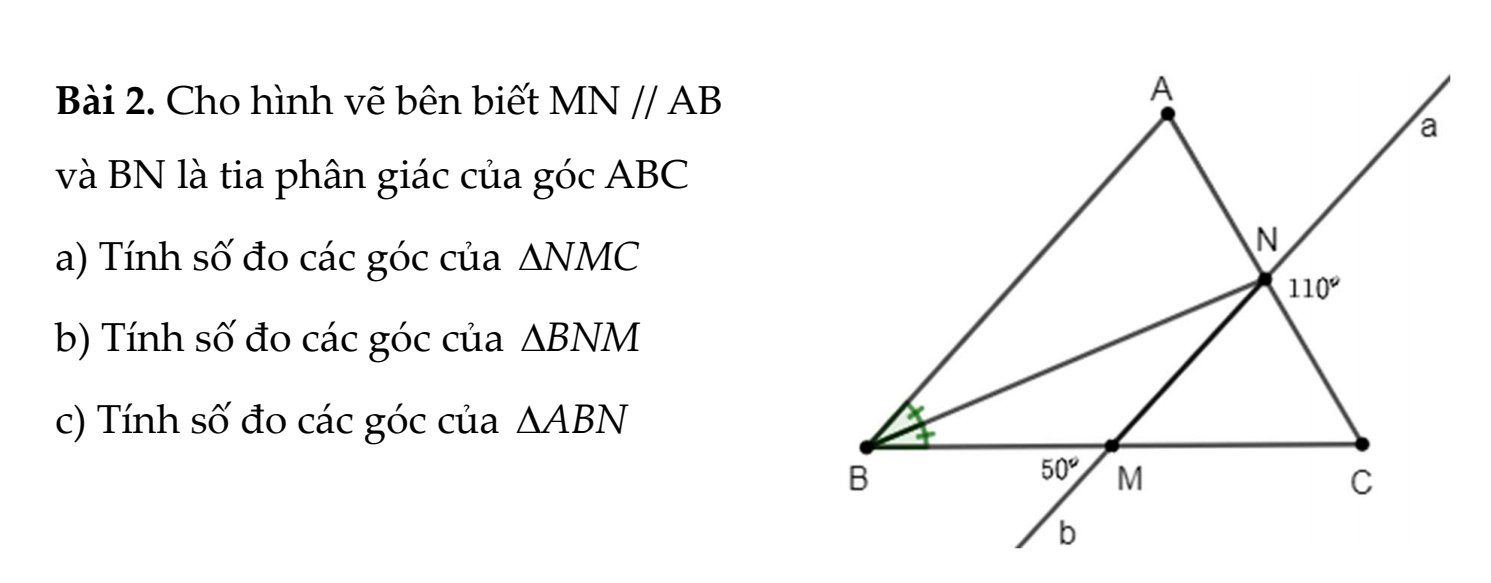
a: Ta có: \(\widehat{bMB}=\widehat{NMC}\)(hai góc đối đỉnh)
mà \(\widehat{bMB}=50^0\)
nên \(\widehat{NMC}=50^0\)
Ta có: \(\widehat{MNC}+\widehat{aNC}=180^0\)(hai góc kề bù)
=>\(\widehat{MNC}+110^0=180^0\)
=>\(\widehat{MNC}=70^0\)
Xét ΔMNC có \(\widehat{NMC}+\widehat{MNC}+\widehat{C}=180^0\)
=>\(\widehat{C}+50^0+70^0=180^0\)
=>\(\widehat{C}=60^0\)
b: Ta có: \(\widehat{NMB}+\widehat{NMC}=180^0\)(hai góc kề bù)
=>\(\widehat{NMB}+50^0=180^0\)
=>\(\widehat{NMB}=130^0\)
Ta có: MN//AB
=>\(\widehat{CMN}=\widehat{CBA}\)(hai góc đồng vị)
=>\(\widehat{CBA}=50^0\)
BN là phân giác của góc CBA
=>\(\widehat{NBM}=\dfrac{\widehat{ABC}}{2}=25^0\)
Xét ΔNMB có \(\widehat{NMB}+\widehat{BNM}+\widehat{NBM}=180^0\)
=>\(\widehat{MNB}=180^0-130^0-25^0=25^0\)
c: BN là phân giác của góc CBA
=>\(\widehat{ABN}=\dfrac{\widehat{ABC}}{2}=25^0\)
Xét ΔABC có \(\widehat{ABC}+\widehat{ACB}+\widehat{BAC}=180^0\)
=>\(\widehat{BAN}+60^0+50^0=180^0\)
=>\(\widehat{BAN}=70^0\)
Xét ΔBAN có \(\widehat{BAN}+\widehat{ABN}+\widehat{ANB}=180^0\)
=>\(\widehat{ANB}=180^0-75^0-25^0=85^0\)