\(\dfrac{x+2}{x-2}-\dfrac{1}{x}=\dfrac{2}{x\left(x-2\right)}\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


<=> 3x^2 -6X -x^2+4= 0
<=> 2 x^2 -6x+4 =0
<=> x^2-3x+2=0
ta có a+b+c = 1-3+2=0
=> x1=1
x2=2
vậy S=...... (tự kết luận )

a, \(\dfrac{3x-2}{3}-1>0\Leftrightarrow\dfrac{3x-5}{3}>0\Leftrightarrow\left\{{}\begin{matrix}x>\dfrac{5}{3}\\x\ne\dfrac{5}{3}\end{matrix}\right.\)
b, \(\dfrac{4x-1}{2}-\dfrac{2x-3}{5}< 0\Leftrightarrow\dfrac{20x-5-4x+6}{10}< 0\Leftrightarrow\left\{{}\begin{matrix}x< -\dfrac{1}{16}\\x\ne-\dfrac{1}{16}\end{matrix}\right.\)

1, đk x khác -2 ; 0
\(x^2-x-x-2=2\Leftrightarrow x^2-2x-4=0\Leftrightarrow\left(x-1\right)^2-5=0\Leftrightarrow x=\pm\sqrt{5}+1\)
2, \(\dfrac{5+x-6}{2}-\dfrac{1-2x}{3}>0\Leftrightarrow\dfrac{3x-3-2+4x}{6}>0\Leftrightarrow\left\{{}\begin{matrix}x>\dfrac{5}{7}\\x\ne\dfrac{5}{7}\end{matrix}\right.\)

Bài 2
a, đk x khác 0 ; -2
\(x^2-x-x-2=2\Leftrightarrow x^2-2x-4=0\)
\(\Leftrightarrow\left(x-1\right)^2-5=0\Leftrightarrow\left[{}\begin{matrix}x=\sqrt{5}+1\\x=-\sqrt{5}+1\end{matrix}\right.\)(tm)
b, \(\dfrac{x+5}{2}-3>\dfrac{1-2x}{3}\Leftrightarrow\dfrac{3x+15-18-2\left(1-2x\right)}{6}>0\)
\(\Leftrightarrow\dfrac{7x-5}{6}>0\Leftrightarrow\left\{{}\begin{matrix}x>\dfrac{5}{7}\\x\ne\dfrac{5}{7}\end{matrix}\right.\)
a, đk x khác -2 ; 0
\(x^2-x-x-2=2\Leftrightarrow x^2-2x-4=0\Leftrightarrow\left(x-1\right)^2-5=0\Leftrightarrow x=\pm\sqrt{5}+1\)
b, \(\dfrac{x+5-6}{2}-\dfrac{1-2x}{3}>0\Leftrightarrow\dfrac{3x-3-2+4x}{6}>0\Leftrightarrow\left\{{}\begin{matrix}x>\dfrac{5}{7}\\x\ne\dfrac{5}{7}\end{matrix}\right.\)

a, Theo định lí Pytago tam giác DEF vuông tại D
\(DF=\sqrt{EF^2-DE^2}=16cm\)
b, Xét tam giác EDF và tam giác DHF có
^EFD _ chung, ^EDF = ^DHF = 900
Vậy tam giác EDF ~ tam giác DHF (g.g)
\(\dfrac{EF}{DF}=\dfrac{DF}{HF}\Rightarrow DF^2=EF.HF\)

\(\dfrac{-3x+1}{2x+1}+2< 0\Leftrightarrow\dfrac{-3x+1+4x+2}{2x+1}< 0\Leftrightarrow\dfrac{x+4}{2x+1}< 0\)
TH1 : \(\left\{{}\begin{matrix}x+4< 0\\2x+1>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x< -4\\x>-\dfrac{1}{2}\end{matrix}\right.\)(vô lí)
TH2 : \(\left\{{}\begin{matrix}x+4>0\\2x+1< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>-4\\x< -\dfrac{1}{2}\end{matrix}\right.\Leftrightarrow-4< x< -\dfrac{1}{2}\)



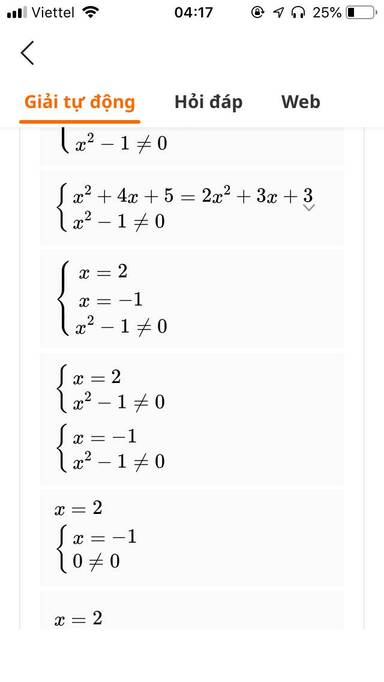
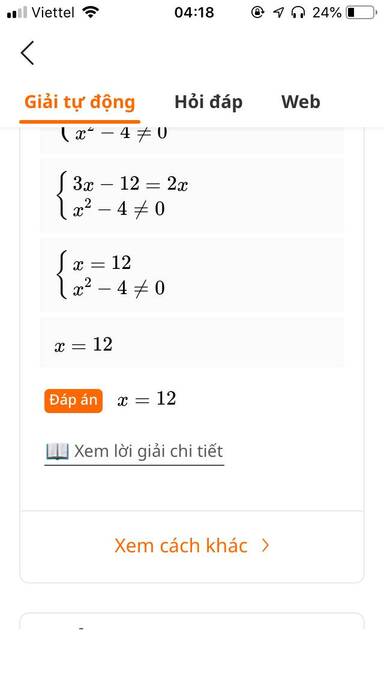
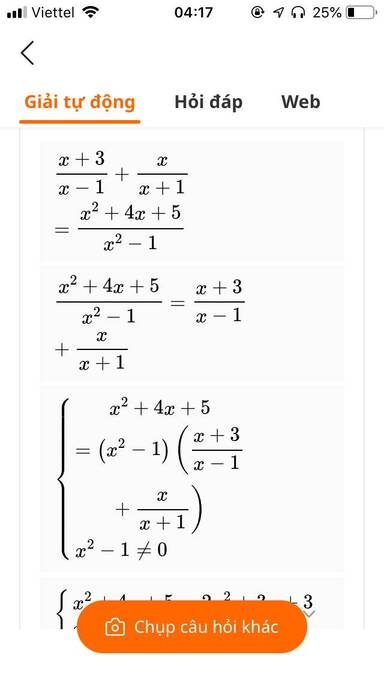
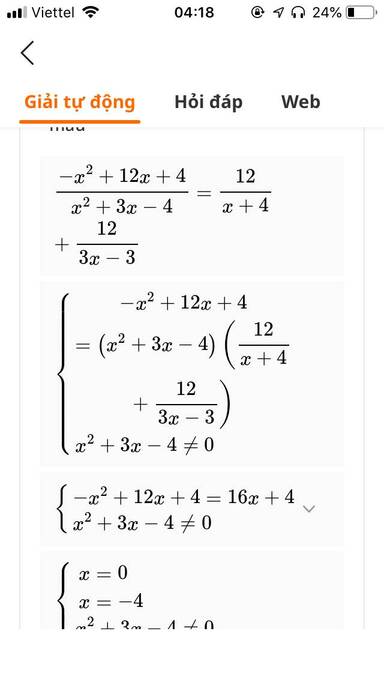
\(\dfrac{x\left(x+2\right)-\left(x-2\right)}{x\left(x-2\right)}=\dfrac{2}{x\left(x-2\right)}\left(đk:x\ne0,2\right)\)
\(\Leftrightarrow x^2+2x-x+2=2\)
\(\Leftrightarrow x^2+x=0\)
\(\Leftrightarrow x\left(x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\left(L\right)\\x=-1\left(TM\right)\end{matrix}\right.\)
\(\dfrac{x+2}{x-2}-\dfrac{1}{x}=\dfrac{2}{x\left(x-2\right)}\) (ĐK: \(x\ne0,x\ne2\))
\(\Leftrightarrow\dfrac{x\left(x+2\right)-\left(x-2\right)}{x\left(x-2\right)}=\dfrac{2}{x\left(x-2\right)}\)
\(\Rightarrow x\left(x+2\right)-\left(x-2\right)=2\)
\(\Leftrightarrow x^2+x=0\)
\(\Leftrightarrow x=-1\) (vì \(x\ne0\))