trong các điểm sau A(0,1); B(-1,1); C(1,3); D(-2,-4); E(2,6).Điểm nào nằm trên đồ thị y=2x^2+1
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(Đk:x\ge0;y\ge1;z\ge2\)
\(\sqrt{x}+\sqrt{y-1}+\sqrt{z-2}=\dfrac{1}{2}\left(x+y+z\right)\)
\(\Leftrightarrow x+y+z-2\sqrt{x}-2\sqrt{y-1}-2\sqrt{z-2}=0\)
\(\Leftrightarrow\left[x-2\sqrt{x}+1\right]-1+\left[\left(y-1\right)-2\sqrt{y-1}+1\right]+\left[\left(z-2\right)-2\sqrt{z-2}+1\right]+1=0\)
\(\Leftrightarrow\left(\sqrt{x}-1\right)^2+\left(\sqrt{y-1}-1\right)^2+\left(\sqrt{z-2}-1\right)^2=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(\sqrt{x}-1\right)^2=0\\\left(\sqrt{y-1}-1\right)^2=0\\\left(\sqrt{z-2}-1\right)^2=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=2\\z=3\end{matrix}\right.\)

\(a,=\sqrt{a}\left(\sqrt{b}-1\right)-\left(\sqrt{b}-1\right)\\ =\left(\sqrt{a}-1\right)\left(\sqrt{b}-1\right)\\ b,=\sqrt{a}\left(\sqrt{a}+1\right)+2\sqrt{b}\left(\sqrt{a}+1\right)\\ =\left(\sqrt{a}+2\sqrt{b}\right)\left(\sqrt{a}+1\right)\\ c,Sửa:x\sqrt{x}+y\sqrt{y}+x+y\\ =\sqrt{xy}\left(x+y\right)+\left(x+y\right)=\left(\sqrt{xy}+1\right)\left(x+y\right)\\ d,=x+\sqrt{x}-2\sqrt{x}-2\\ =\sqrt{x}\left(\sqrt{x}+1\right)-2\left(\sqrt{x}+1\right)\\ =\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)\)

\(a,x\sqrt{x}+\sqrt{x}-x-1\\ =\sqrt{x}\left(x+1\right)-\left(x+1\right)\\ =\left(\sqrt{x}-1\right)\left(x+1\right)\\ b,\sqrt{ab}+2\sqrt{a}+3\sqrt{b}+6\\ =\sqrt{a}\left(\sqrt{b}+2\right)+3\left(\sqrt{b}+2\right)\\ =\left(\sqrt{a}+3\right)\left(\sqrt{b}+2\right)\)
`a, x sqrt x + sqrt x - x - 1`
`= sqrt x(x+1) - (x+1)`
`= sqrt(x-1)(x+1)`
`b, sqrt a(sqrt b + 2) + 3(sqrt b + 2)`
`= (sqrt a + 3)(sqrt b+2)`

Gọi \(B\left(x_0;y_0\right)\in\left(d\right)\Rightarrow y_0=-x_0+4\)
\(AB=\sqrt{\left(x_0-1\right)^2+\left(y_0-4\right)^2}\\ \Leftrightarrow AB^2=\left(x_0-1\right)^2+\left(-x_0+4-4\right)^2\\ =2x^2_0-2x_0+1=\left(\sqrt{2}x-\dfrac{1}{\sqrt{2}}\right)^2+\dfrac{1}{2}\ge\dfrac{1}{2}\)
Dễ thấy AB nhỏ nhất khi \(\left(\sqrt{2}x_0-\dfrac{1}{\sqrt{2}}\right)^2=0\Rightarrow\sqrt{2}x_0-\dfrac{1}{\sqrt{2}}=0\\ \Rightarrow x_0=\dfrac{1}{\sqrt{2}}:\sqrt{2}=\dfrac{1}{2}\Rightarrow y_0=\dfrac{7}{2}\)
Vậy \(B\left(\dfrac{1}{2};\dfrac{7}{2}\right)\) thì AB bé nhất và bằng \(\dfrac{\sqrt{2}}{2}\)

x2 + \(\dfrac{18x}{5}\) - 64 = 0
△ = (18/5)2 -4.(-64) = \(\dfrac{6724}{25}\)
x = { -(18/5) + - (82/5)}: 2
x ϵ {32/5; -10}
rút gọn A=\(\dfrac{\sqrt{x}+1}{\sqrt{x}-1}+\dfrac{\sqrt{x}-1}{\sqrt{x}+1}-\dfrac{3\sqrt{x}+1}{x-1}\)

Với \(x \ge 0,x \ne 1\) có:
\(A=\dfrac{\sqrt{x}+1}{\sqrt{x}-1}+\dfrac{\sqrt{x}-1}{\sqrt{x}+1}-\dfrac{3\sqrt{x}+1}{x-1}\)
\(A=\dfrac{(\sqrt{x}+1)^2+(\sqrt{x}-1)^2-3\sqrt{x}-1}{(\sqrt{x}+1)(\sqrt{x}-1)}\)
\(A=\dfrac{x+2\sqrt{x}+1+x-2\sqrt{x}+1-3\sqrt{x}-1}{(\sqrt{x}+1)(\sqrt{x}-1)}\)
\(A=\dfrac{2x-3\sqrt{x}+1}{(\sqrt{x}+1)(\sqrt{x}-1)}\)
\(A=\dfrac{(\sqrt{x}-1)(2\sqrt{x}-1)}{(\sqrt{x}+1)(\sqrt{x}-1)}\)
\(A=\dfrac{2\sqrt{x}-1}{\sqrt{x}+1}\)
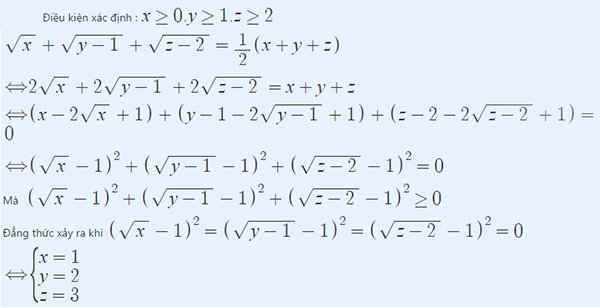
xét A(0;1) ta có: 2.02 + 1 = 1 vậy A ϵ y = 2x2 + 1
xét B(-1,1) ta có : 2.(-1)2 + 1 = 3 vậy B \(\notin\) y = 2x2 + 1
xét C(1,3) ta có: 2.12 + 1 = 3 vậy C ϵ y = 2x2 + 1
xét D(-2,-4) ta có : 2.(-2)2 + 1 = 9 vậy D \(\notin\) y = 2x2 + 1
xét E(2,6) ta có : 2.22 + 1 = 9 vậy E \(\notin\) y = 2x2 + 1
Bạn thay lần lượt từng điểm vào nhé. Ví dụ A(0,1) ta thay x=0 vào đồ thị ta được kết quả y=1 => A thuộc đồ thị
Tương tự như vậy ta có điểm C(1;3) cũng thuộc đồ thị