Diện tích xung quanh của căn nhà là: 

\(2\times\left(25+6\right)\times5=310\left(m^2\right)\)

Diện tích trần nhà là:

\(25\times6=150\left(m^2\right)\)

Diện tích cửa ra vào là:

\(3\times15=45\left(m^2\right)\)

Diện tích hai cửa sổ là:

\(2\times\left(1\times1\right)=2\left(m^2\right)\)

Diện tích cần sơn là:

\(\left(310+150\right)-\left(45+2\right)=413\left(m^2\right)\)

Chi phí để sơn căn phòng là:

\(413:1,2\times30000=10325000\)

Vậy...

giúp mình với

a: Ta có: \(\widehat{xOy}+\widehat{yOz}=180^0\)(hai góc kề bù)

=>\(\widehat{yOz}+120^0=180^0\)

=>\(\widehat{yOz}=60^0\)

b: Om là phân giác của góc xOy

=>\(\widehat{xOm}=\widehat{yOm}=\dfrac{\widehat{xOy}}{2}=60^0\)

Ta có: \(\widehat{xOm}+\widehat{zOm}=180^0\)(hai góc kề bù)

=>\(\widehat{zOm}+60^0=180^0\)

=>\(\widehat{zOm}=120^0\)

c: Ta có: \(\widehat{yOz}=\widehat{yOm}\left(=60^0\right)\)

mà tia Oy nằm giữa hai tia Oz và Om(Vì \(\widehat{yOz}+\widehat{yOm}=\widehat{zOm}\))

nên Oy là phân giác của góc zOm

Ta có: \(\widehat{AOD}=\widehat{BOC}\)(hai góc đối đỉnh)

mà \(\widehat{AOD}+\widehat{BOC}=110^0\)

nên \(\widehat{AOD}=\widehat{BOC}=\dfrac{110^0}{2}=55^0\)

Ta có: \(\widehat{AOD}+\widehat{AOC}=180^0\)(hai góc kề bù)

=>\(\widehat{AOC}+55^0=180^0\)

=>\(\widehat{AOC}=125^0\)

Ta có: \(\widehat{AOC}=\widehat{BOD}\)(hai góc đối đỉnh)

mà \(\widehat{AOC}=125^0\)

nên \(\widehat{BOD}=125^0\)

Ta có: \(\widehat{BOC}=\widehat{AOD}\)(hai góc đối đỉnh)

mà \(\widehat{BOC}=45^0\)

nên \(\widehat{AOD}=45^0\)

Ta có: \(\widehat{AOD}+\widehat{DOB}=180^0\)(hai góc  kề bù)

=>\(\widehat{DOB}+45^0=180^0\)

=>\(\widehat{DOB}=135^0\)

Ta có: \(\widehat{DOB}=\widehat{AOC}\)(hai góc đối đỉnh)

mà \(\widehat{DOB}=135^0\)

nên \(\widehat{AOC}=135^0\)

b: (2x+1):2=12:3

=>(2x+1):2=4

=>2x+1=2*4=8

=>2x=7

=>\(x=\dfrac{7}{2}\)

d: \(\dfrac{2x-14}{3}=\dfrac{12}{9}\)

=>\(\dfrac{2x-14}{3}=\dfrac{4}{3}\)

=>2x-14=4

=>2x=18

=>x=9

a: tia OB nằm giữa hai tia OA và OC

=>\(\widehat{AOB}+\widehat{BOC}=\widehat{AOC}\)

=>\(\widehat{BOC}=165^0-20^0=145^0\)

b: Ta có: \(\widehat{BOC}+\widehat{COD}=180^0\)(hai góc kề bù)

=>\(\widehat{COD}+145^0=180^0\)

=>\(\widehat{COD}=35^0\)

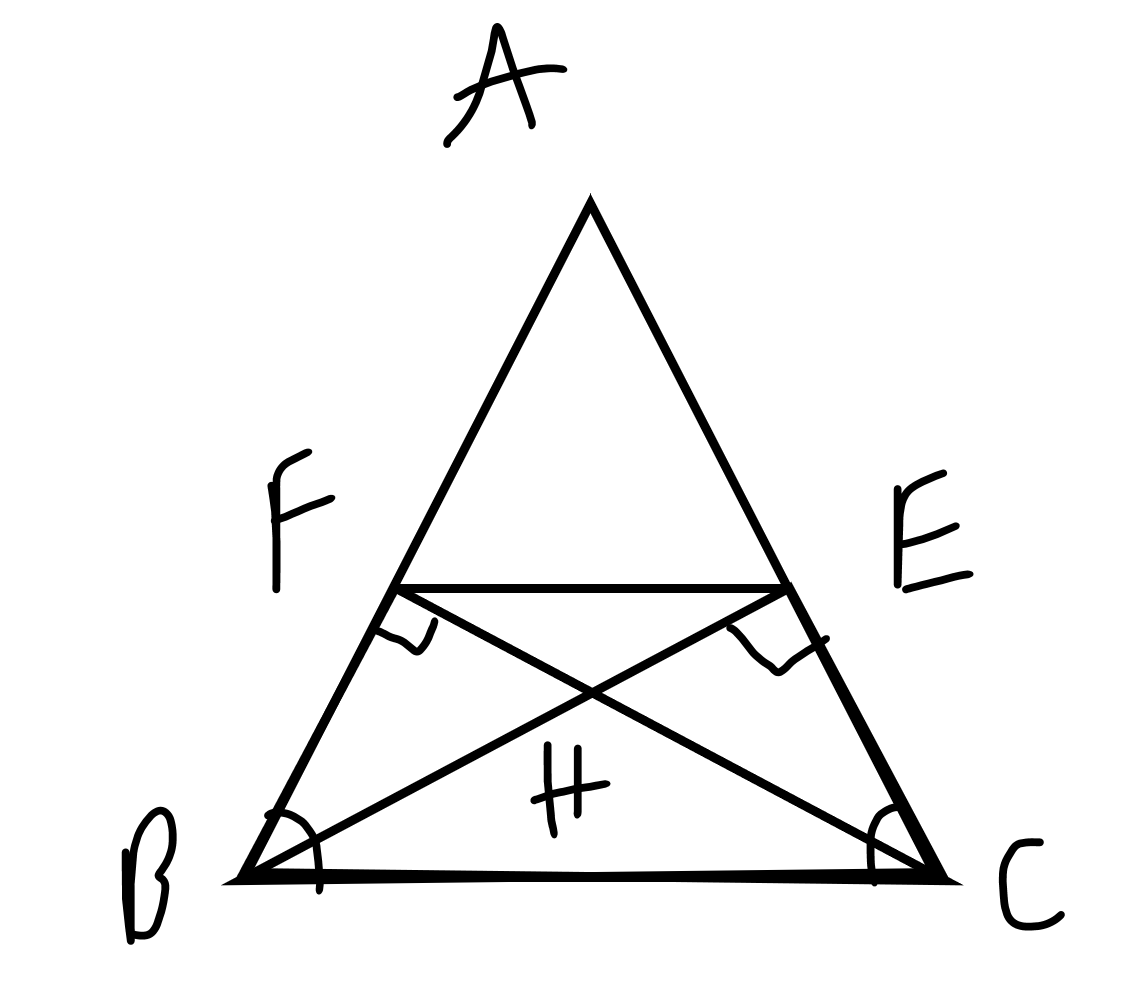
a: Xét ΔAEB vuông tại E và ΔAFC vuông tại F có

AB=AC

\(\widehat{BAE}\) chung

Do đó: ΔAEB=ΔAFC

=>BE=CF

b: ΔAEB=ΔAFC

=>AE=AF

Xét ΔAFH vuông tại F và ΔAEH vuông tại E có

AH chung

AF=AE

Do đó: ΔAFH=ΔAEH

=>HE=HF

=>ΔHEF cân tại H

c: Xét ΔABC có \(\dfrac{AE}{AC}=\dfrac{AF}{AB}\)

nên EF//BC

d: Ta có: AE=AF

=>A nằm trên đường trung trực của EF(1)

Ta có: HE=HF

=>H nằm trên đường trung trực của EF(2)

Từ (1),(2) suy ra AH là đường trung trực của EF

=>AH\(\perp\)EF

\(a)\left(x^6+x^7-x^9\right):x\\ =x^6:x+x^7:x-x^9:x\\ =x^5+x^6x^8\\ b)\left(6x^4+4x^3+8x^2\right):2x^2\\ =6x^4:2x^2+4x^3:2x^2+8x^2:2x^2\\ =3x^2+2x+4\\ c)\left(14x^4+21x^5+7x^7\right):x^3\\ =14x^4:x^3+21x^5:x^3+7x^7:x^3\\ =14x+21x^2+7x^4\\ d)\left(-x^2+4x\right):\left(x-4\right)\\ =-x\left(x-4\right):\left(x-4\right)\\ =-x\)

\(e)\left(x^2+x-12\right):\left(x-3\right)\\ =\left(x^2-3x+4x-12\right):\left(x-3\right)\\ =\left[x\left(x-3\right)+4\left(x-3\right)\right]:\left(x-3\right)\\ =\left(x-3\right)\left(x+4\right):\left(x-3\right)\\ =x+4\\ g)\left(x^2+5x-6\right):\left(x-1\right)\\ =\left(x^2-x+6x-6\right):\left(x-1\right)\\ =\left[x\left(x-1\right)+6\left(x-1\right)\right]:\left(x-1\right)\\ =\left(x+6\right)\left(x-1\right):\left(x-1\right)\\ =x+6\\ i)\left(x^3+5x^2+11x+10\right):\left(x+2\right)\\ =\left(x^3+2x^2+3x^2+6x+5x+10\right):\left(x+2\right)\\ =\left[x^2\left(x+2\right)+3x\left(x+2\right)+5\left(x+2\right)\right]:\left(x+2\right)\\ =\left(x^2+3x+5\right)\left(x+2\right):\left(x+2\right)\\ =x^2+3x+5\)

(xem lại đề câu f vs h) 

\(a)6x\left(2x-1\right)\\ =6x\cdot2x-6x\\ =12x^2-6x\\ b)\left(5x-3\right)\dfrac{2}{3}x\\ =5x\cdot\dfrac{2}{3}x-3\cdot\dfrac{2}{3}x\\ =\dfrac{10}{3}x^2-2x\\c)-5x^3\left(3x^2-7\right)\\ =-5x^3\cdot3x^2-5x^3\cdot\left(-7\right)\\ =-15x^5+35x^3\\ d)-3x\left(5x^2-2x-1\right)\\ =-3x\cdot5x^2-3x\cdot-2x-3x\cdot-1\\ =-15x^3+6x^2+3x\)

\(e)\left(3x-x^2+6\right)\dfrac{2}{3}x^2\\ =3x\cdot\dfrac{2}{3}x^2-x^2\cdot\dfrac{2}{3}x^2+6\cdot\dfrac{2}{3}x^2\\ =2x^3-\dfrac{2}{3}x^4+4x^2\\ f)-\dfrac{5}{6}x\left(\dfrac{2}{3}x^4+\dfrac{3}{14}x^3-\dfrac{2}{3}x^2\right)\\ =-\dfrac{5}{6}x\cdot\dfrac{2}{3}x^4-\dfrac{5}{6}x\cdot\dfrac{3}{14}x^3+\dfrac{2}{3}x^2\cdot\dfrac{5}{6}x\\ =-\dfrac{5}{9}x^5-\dfrac{5}{28}x^4+\dfrac{5}{9}x^3\\ g)\left(4x-3\right)\left(x+2\right)\\ =4x\left(x+2\right)-3\left(x+2\right)\\ =4x^2+8x-3x-6\\ =4x^2+5x-6\\ h)\left(5x+2\right)\left(-x^2+3x+1\right)\\ =5x\left(-x^2+3x+1\right)+2\left(-x^2+3x+1\right)\\ =-5x^3+15x^2+5x-2x^2+6x+2\\ =-5x^3+13x^2+11x+2\)

Ta có: 

\(P\left(x\right)=x^5-3x^5+7x^4-9x^3+x^3+x^2-\dfrac{1}{4}x\\ =-2x^5+7x^4-8x^3+x^2-\dfrac{1}{4}x\\ =x\cdot\left(-2x^4+7x^3-8x^2+x-\dfrac{1}{4}\right)\)

Thay `x=0` vào P(x) ta có:

\(P\left(x\right)=0\cdot\left(2\cdot0^4+7\cdot0^3-8\cdot0^2+0-\dfrac{1}{4}\right)=0\)

=> `x=0` là nghiệm của P(x) 

\(Q\left(x\right)=5x^4-x^5+x^2-2x^3+3x^2-\dfrac{1}{4}\\ =-x^5+5x^4-2x^3+4x^2-\dfrac{1}{4}\)

Thay `x=0` vào Q(x) ta có: 

\(Q=-0^5+5\cdot0^4-2\cdot0^3+4\cdot0^2-\dfrac{1}{4}=-\dfrac{1}{4}\)

=> `x=0` không phải là nghiệm của Q(x) 

Ta có:

P(0) = 0⁵ - 3⁵ + 7.0⁴ - 9.0³ + 0³ + 0² - 1/4 . 0

= -3⁵

= -243

Vậy x = 0 không là nghiệm của P(x)

Em xem lại đề nhé!