Thực hiện phép nhân sau :
a,\(6x\left(2x-1\right)\) e,\(\left(3x-x^2+6\right)\dfrac{2}{3}x^2\)
b,\(\left(5x-3\right)\dfrac{2}{3}x\) f,\(-\dfrac{5}{6}x\left(\dfrac{2}{3}x^4+\dfrac{3}{14}x^3-\dfrac{2}{3}x^2\right)\)
c,\(-5x^3\left(3x^2-7\right)\) g,\(\left(4x-3\right)\left(x+2\right)\)
d,\(-3x\left(5x^2-2x-1\right)\) h, \(\left(5x+2\right)\left(-x^2+3x+1\right)\)
i, \(\left(2x^3-7x+4\right)\left(-13x^2+6x+5\right)\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Ta có:
\(P\left(x\right)=x^5-3x^5+7x^4-9x^3+x^3+x^2-\dfrac{1}{4}x\\ =-2x^5+7x^4-8x^3+x^2-\dfrac{1}{4}x\\ =x\cdot\left(-2x^4+7x^3-8x^2+x-\dfrac{1}{4}\right)\)
Thay `x=0` vào P(x) ta có:
\(P\left(x\right)=0\cdot\left(2\cdot0^4+7\cdot0^3-8\cdot0^2+0-\dfrac{1}{4}\right)=0\)
=> `x=0` là nghiệm của P(x)
\(Q\left(x\right)=5x^4-x^5+x^2-2x^3+3x^2-\dfrac{1}{4}\\ =-x^5+5x^4-2x^3+4x^2-\dfrac{1}{4}\)
Thay `x=0` vào Q(x) ta có:
\(Q=-0^5+5\cdot0^4-2\cdot0^3+4\cdot0^2-\dfrac{1}{4}=-\dfrac{1}{4}\)
=> `x=0` không phải là nghiệm của Q(x)
Ta có:
P(0) = 0⁵ - 3⁵ + 7.0⁴ - 9.0³ + 0³ + 0² - 1/4 . 0
= -3⁵
= -243
Vậy x = 0 không là nghiệm của P(x)
Em xem lại đề nhé!

ĐKXĐ: \(x\ne\pm\dfrac{1}{2}\)
\(\dfrac{8x^2}{3\left(1-4x^2\right)}=\dfrac{2x}{6x-3}-\dfrac{1+8x}{4+8x}\)
\(\Leftrightarrow\dfrac{-8x^2}{3\left(2x-1\right)\left(2x+1\right)}=\dfrac{2x}{3\left(2x-1\right)}-\dfrac{1+8x}{4\left(2x+1\right)}\)
\(\Leftrightarrow\dfrac{-32x^2}{12\left(2x-1\right)\left(2x+1\right)}=\dfrac{8x\left(2x+1\right)}{12\left(2x-1\right)\left(2x+1\right)}-\dfrac{3\left(1+8x\right)\left(2x-1\right)}{12\left(2x-1\right)\left(2x+1\right)}\)
\(\Rightarrow-32x^2=16x^2+8x-3\left(16x^2-6x-1\right)\)
\(\Leftrightarrow-32x^2=16x^2+8x-48x^2+18x+3\)
\(\Leftrightarrow-32x^2=-32x^2+26x+3\)
\(\Leftrightarrow26x+3=0\)
\(\Leftrightarrow26x=-3\)
\(\Leftrightarrow x=-\dfrac{3}{26}\) (tmđk)
$Toru$

2,5+3,2+2,7
=5,7+2,7
=8,4
\(\left[-1,5\right]-\dfrac{1}{5}=-\dfrac{3}{2}-\dfrac{1}{5}=-\dfrac{15}{10}-\dfrac{2}{10}=-\dfrac{17}{10}\)
`#3107.101107`
`2,5 + 3,2 + 2,7`
`= 5,7 + 2,7`
`= 8,4`
____
$-1,5 - \dfrac{1}{5}$
`= -1,5 - 0,2`
`= -1,7`

Ta có: \(\dfrac{y+z+2}{x}=\dfrac{x+z+3}{y}=\dfrac{x+y-5}{z}=\dfrac{1}{x+y+z}\) (\(x,y,z\ne0\))
Áp dụng tính chất của dãy tỉ số bằng nhau, ta được:
\(\dfrac{y+z+2}{x}=\dfrac{x+z+3}{y}=\dfrac{x+y-5}{z}=\dfrac{1}{x+y+z}\)
\(=\dfrac{y+z+2+x+z+3+x+y-5}{x+y+z}=\dfrac{2\left(x+y+z\right)}{x+y+z}=2\)
\(\Rightarrow\left\{{}\begin{matrix}y+z+2=2x\\x+z+3=2y\\x+y-5=2z\\x+y+z=\dfrac{1}{2}\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}x+y+z+2=3x\\x+y+z+3=3y\\x+y+z-5=3z\\x+y+z=\dfrac{1}{2}\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}3x=\dfrac{1}{2}+2=\dfrac{5}{2}\\3y=\dfrac{1}{2}+3=\dfrac{7}{2}\\3z=\dfrac{1}{2}-5=-\dfrac{9}{2}\\x+y+z=\dfrac{1}{2}\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}x=\dfrac{5}{6}\left(tm\right)\\y=\dfrac{7}{6}\left(tm\right)\\z=-\dfrac{3}{2}\left(tm\right)\end{matrix}\right.\)
#$\mathtt{Toru}$

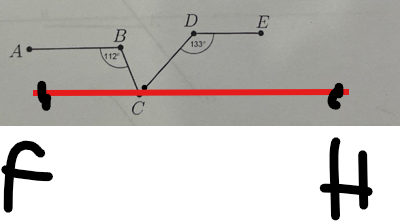
Bài 7: Kẻ CF//AB//DE(CF,AB cùng nằm trên nửa mặt phẳng bờ AC)
CF//AB
=>\(\widehat{BAC}+\widehat{ACF}=180^0\)
CF//DE
=>\(\widehat{FCD}+\widehat{CDE}=180^0\)
\(\widehat{BAC}+\widehat{ACF}+\widehat{FCD}+\widehat{CDE}\)
\(=180^0+180^0=360^0\)
=>\(\widehat{BAC}+\widehat{ACD}+\widehat{CDE}=360^0\)
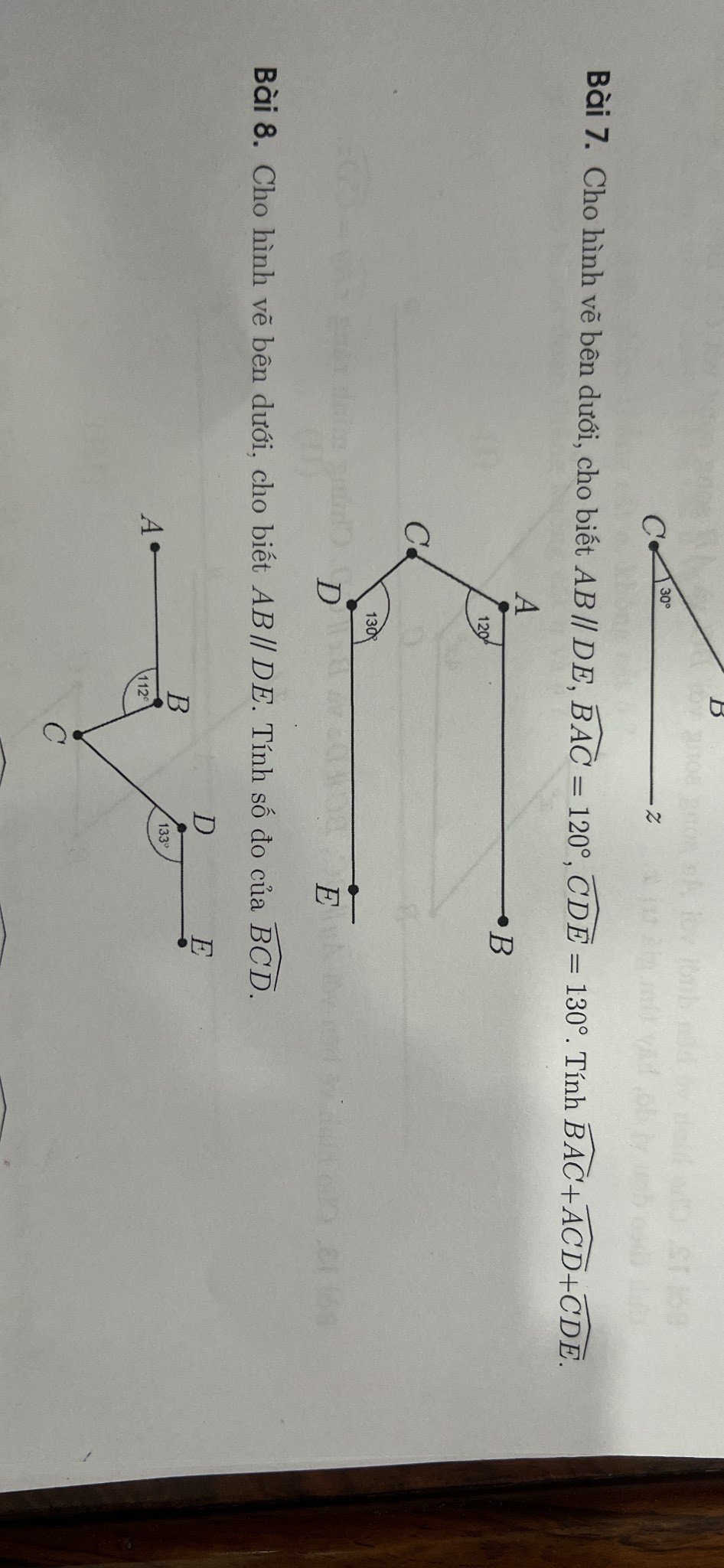
Bài 8:
Qua C, kẻ FH//AB//DE
FC//AB
=>\(\widehat{ABC}+\widehat{FCB}=180^0\)
=>\(\widehat{FCB}=68^0\)
Ta có: CH//DE
=>\(\widehat{CDE}+\widehat{DCH}=180^0\)
=>\(\widehat{DCH}=180^0-133^0=47^0\)
Ta có: \(\widehat{BCF}+\widehat{BCD}+\widehat{HCD}=180^0\)
=>\(\widehat{BCD}+47^0+68^0=180^0\)
=>\(\widehat{BCD}=65^0\)

a) Để `x/7` là số hữu tỉ thì \(x\in Z\)
b) Để `5/x` là số hữu tỉ thì \(x\in Z,x\ne0\)
c) Để `-5/(2x)` là số hữu tỉ thì \(2x\in Z\Rightarrow x\in Z,x\ne0\)

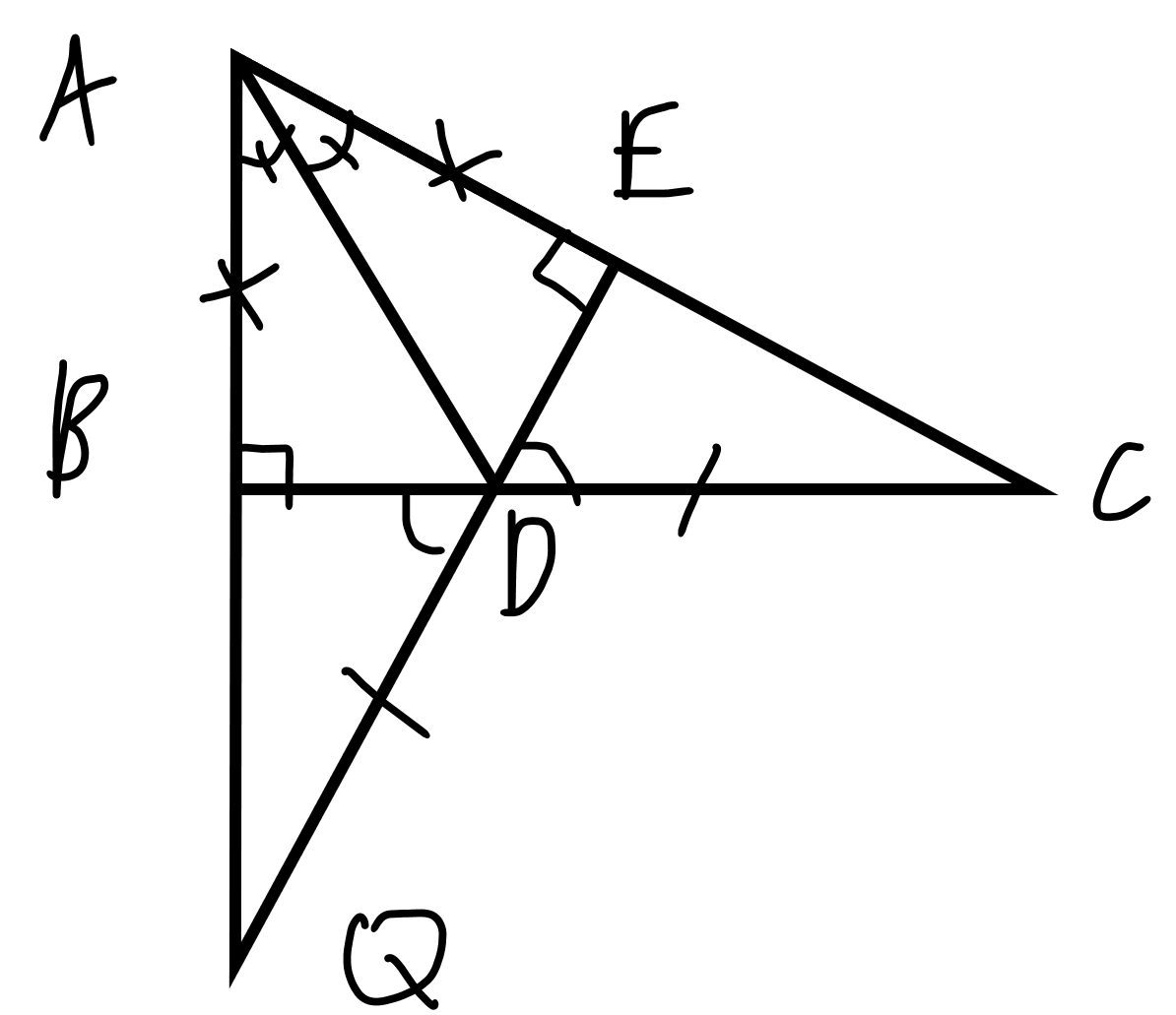
*chứng minh AB = AE
xét tam giác vuông ABD và tam giác vuông AED, có:
góc BAD = góc EAD (vì A là đường phân giác của tam giác ABC)
AD là cạnh chung
=> tam giác ABD = tam giác AED (ch-gn)
=> AB = AE (2 cạnh tương ứng)
*chứng minh DQ = CD
xét tam giác AEQ và tam giác ABC , có:
góc AEQ = góc ABC (= 90 độ)
AB = AE (câu a)
góc A là góc chung
=> tam giác AEQ = tam giác ABC (c-g-c)
=> QE = BC (1)
ta có: DC = BC - BD; DQ = QE - DE (2)
lại có: DB = DE (vì tam giác ABD = tam giác AED) (3)
=> TỪ (1) (2) (3) => DC = DQ
\(a)6x\left(2x-1\right)\\ =6x\cdot2x-6x\\ =12x^2-6x\\ b)\left(5x-3\right)\dfrac{2}{3}x\\ =5x\cdot\dfrac{2}{3}x-3\cdot\dfrac{2}{3}x\\ =\dfrac{10}{3}x^2-2x\\c)-5x^3\left(3x^2-7\right)\\ =-5x^3\cdot3x^2-5x^3\cdot\left(-7\right)\\ =-15x^5+35x^3\\ d)-3x\left(5x^2-2x-1\right)\\ =-3x\cdot5x^2-3x\cdot-2x-3x\cdot-1\\ =-15x^3+6x^2+3x\)
\(e)\left(3x-x^2+6\right)\dfrac{2}{3}x^2\\ =3x\cdot\dfrac{2}{3}x^2-x^2\cdot\dfrac{2}{3}x^2+6\cdot\dfrac{2}{3}x^2\\ =2x^3-\dfrac{2}{3}x^4+4x^2\\ f)-\dfrac{5}{6}x\left(\dfrac{2}{3}x^4+\dfrac{3}{14}x^3-\dfrac{2}{3}x^2\right)\\ =-\dfrac{5}{6}x\cdot\dfrac{2}{3}x^4-\dfrac{5}{6}x\cdot\dfrac{3}{14}x^3+\dfrac{2}{3}x^2\cdot\dfrac{5}{6}x\\ =-\dfrac{5}{9}x^5-\dfrac{5}{28}x^4+\dfrac{5}{9}x^3\\ g)\left(4x-3\right)\left(x+2\right)\\ =4x\left(x+2\right)-3\left(x+2\right)\\ =4x^2+8x-3x-6\\ =4x^2+5x-6\\ h)\left(5x+2\right)\left(-x^2+3x+1\right)\\ =5x\left(-x^2+3x+1\right)+2\left(-x^2+3x+1\right)\\ =-5x^3+15x^2+5x-2x^2+6x+2\\ =-5x^3+13x^2+11x+2\)