Đề tự luận SVIP

Hướng dẫn giải:

a.

+ TH1: $\left| x-1 \right|=0\Leftrightarrow x=1$, bất phương trình thỏa mãn.

+ TH2: $\left| x-1 \right|>0$

Khi đó $(*) \Leftrightarrow {{x}^{2}}-2x>0 \Leftrightarrow \left[ \begin{aligned} & x>2 \\ & x<0 \\ \end{aligned} \right.$.

Vậy tập nghiệm của bất phương trình là $S=\left( -\infty \,;\,0 \right)\cup \left( 2\,;\,+\infty \right)\cup \left\{ 1 \right\}$.

b.

$\left( x-1 \right)\left( {{x}^{2}}-5x+4 \right)<0$

Đặt $f\left( x \right)=\left( x-1 \right)\left( {{x}^{2}}-5x+4 \right)$

$x-1=0\Leftrightarrow x=1$ ${{x}^{2}}-5x+4=0\Leftrightarrow \left[ \begin{aligned} & x=1 \\ & x=4 \\ \end{aligned} \right.$

Bảng xét dấu:

Ta có $f\left( x \right)=\left( x-1 \right)\left( {{x}^{2}}-5x+4 \right)<0\Leftrightarrow x\in \left( -\infty ;1 \right)\cup \left( 1;+\infty \right)$.

Vậy $S=\left( -\infty ;1 \right)\cup \left( 1;4 \right)$.

Hướng dẫn giải:

a. $VT=1-\dfrac{{{\sin }^{3}}x}{\sin \,x+\cos x}-\dfrac{{{\cos }^{3}}x}{\sin \,x+\cos x}=1-\dfrac{\left( \sin \,x+\cos \,x \right)\left( {{\sin }^{2}}x+{{\cos }^{2}}x-\sin \,x\,\cos \,x \right)}{\sin \,x\,+\cos \,x}$ $=1-\left( {{\sin }^{2}}x+{{\cos }^{2}}x-\sin \,x\,\cos \,x \right)=\sin \,x\,\cos x=VP$.

b. $VT=\dfrac{2\,\cos x-{{\cos }^{2}}x}{\left( 2-\cos x \right)\left( 1+\cos x \right)}=\dfrac{\,\left( 2-\cos x \right)\cos x}{\left( 2-\cos x \right)\left( 1+\cos x \right)}=\dfrac{\cos x}{1+\cos x}=VP$.

Hướng dẫn giải:

Đặt $f\left( x \right)=\left( {{m}^{2}}+1 \right){{x}^{2}}+\left( 2m+1 \right)x-5$.

Ta có $\Delta ={{\left( 2m+1 \right)}^{2}}+20\left( {{m}^{2}}+1 \right)>0\,,\,\,\forall m\in \mathbb{R}$.

Suy ra tam thức luôn có hai nghiệm ${{x}_{1}}<{{x}_{2}}$.

Nên bất phương trình có tập nghiệm $S=\left( {{x}_{1}};\,{{x}_{2}} \right)$.

Yêu cầu bài toán trở thành tìm $m$ để phương trình$f\left( x \right)=0$ có hai nghiệm phân biệt thỏa mãn ${{x}_{1}}\le -1<1\le {{x}_{2}}$.

$\Leftrightarrow \left\{ \begin{aligned} & \left( {{x}_{1}}-1 \right)\left( {{x}_{2}}-1 \right)\le 0 \\ & \left( {{x}_{1}}+1 \right)\left( {{x}_{2}}+1 \right)\le 0 \\ \end{aligned} \right.\Leftrightarrow \left\{ \begin{aligned} & {{x}_{1}}.{{x}_{2}}-\left( {{x}_{1}}+{{x}_{2}} \right)+1\le 0 \\ & {{x}_{1}}.{{x}_{2}}+\left( {{x}_{1}}+{{x}_{2}} \right)+1\le 0 \\ \end{aligned} \right.\Leftrightarrow \left\{ \begin{aligned} & -\dfrac{5}{{{m}^{2}}+1}+\dfrac{2m+1}{{{m}^{2}}+1}+1\le 0 \\ & -\dfrac{5}{{{m}^{2}}+1}-\dfrac{2m+1}{{{m}^{2}}+1}+1\le 0 \\ \end{aligned} \right.$.

$\Leftrightarrow \left\{ \begin{aligned} & {{m}^{2}}+2m-3\le 0 \\ & {{m}^{2}}-2m-5\le 0 \\ \end{aligned} \right.\Leftrightarrow \left\{ \begin{aligned} & -3\le m\le 1 \\ & 1-\sqrt{6}\le m\le 1+\sqrt{6} \\ \end{aligned} \right.\Leftrightarrow 1-\sqrt{6}\le m\le 1$.

Hướng dẫn giải:

Đặt $\left( E \right):\dfrac{{{x}^{2}}}{{{a}^{2}}}+\dfrac{{{y}^{2}}}{{{b}^{2}}}=1,\,\,\left( a>b>0 \right)$.

Ta có $\left\{ \begin{aligned} & {{a}^{2}}=36 \\ & {{b}^{2}}=25 \\ \end{aligned} \right.\Rightarrow \left\{ \begin{aligned} & a=6 \\ & b=5 \\ \end{aligned} \right.\Rightarrow c=\sqrt{11}$.

Tiêu điểm: ${{F}_{1}}\left( -\sqrt{11};0 \right),\,\,{{F}_{2}}\left( \sqrt{11}\,;0 \right)$.

Tiêu cự: ${{F}_{1}}{{F}_{2}}=2c=2\sqrt{11}$.

Trục lớn: ${{A}_{1}}{{A}_{2}}=2a=12$. Trục bé: ${{B}_{1}}{{B}_{2}}=2b=10$.

Tâm sai: $e=\dfrac{c}{a}=\dfrac{\sqrt{11}}{6}$.

b.

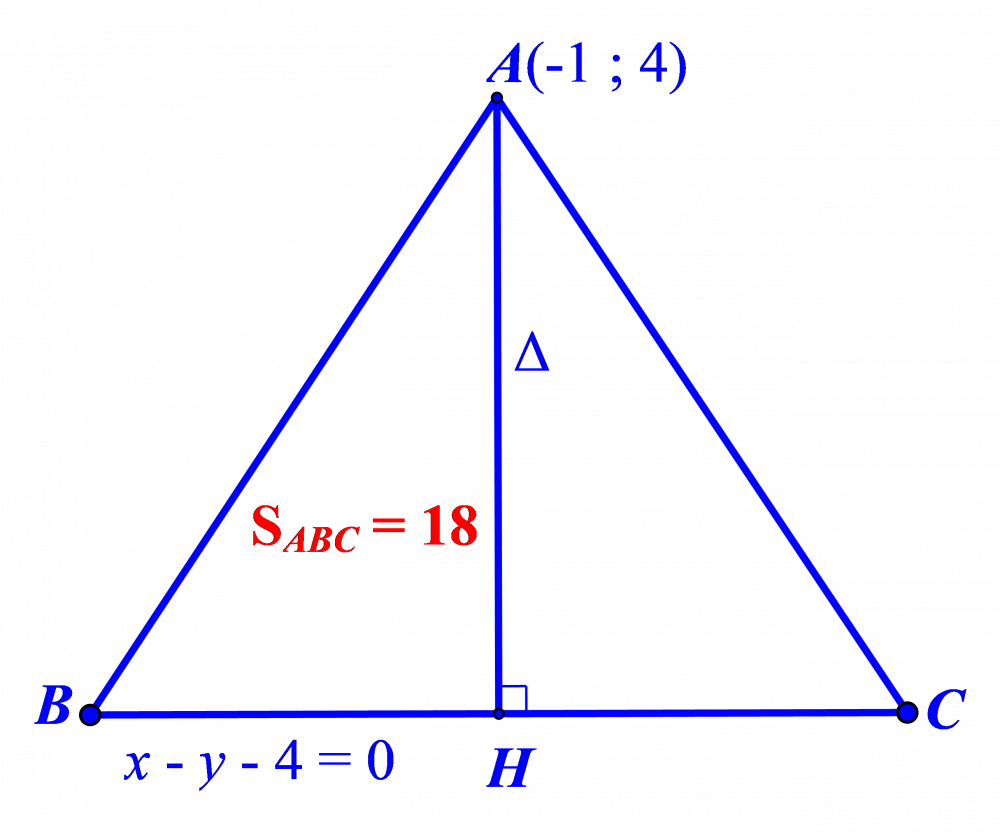
+ Gọi $H$ là trung điểm của $BC$

$\Rightarrow $ $H$ là hình chiếu vuông góc của $A$ lên $BC$.

+ Gọi $\Delta $ là đường thẳng qua $A$ và vuông góc với $BC$

$\Rightarrow $ phương trình $\Delta \,:x+y-3=0$.

+ Vì $H$ là giao điểm của $BC$ và $\Delta $

$\Rightarrow $ Tọa độ điểm $H$ là nghiệm của hệ phương trình $\left\{ \begin{aligned} & x+y-3=0 \\ & x-y-4=0 \\ \end{aligned} \right.\Rightarrow \left\{ \begin{aligned} & x=\dfrac{7}{2} \\ & y=-\dfrac{1}{2} \\ \end{aligned} \right.$

$\Rightarrow H\left( \dfrac{7}{2}\,;\,-\dfrac{1}{2} \right)$.

+ Ta có: $B\in BC\Rightarrow B\left( a\,;\,a-4 \right)$.

$C$ là điểm đối xứng của $B$ qua $H$

$\Rightarrow C\left( 7-a\,;\,3-a \right)$.

+ Theo giả thiết, diện tích tam giác $ABC$ là $S=\dfrac{1}{2}.BC.AH=18$ $\left( 1 \right)$ trong đó $\left\{ \begin{aligned} & \overrightarrow{AH}=\left( \dfrac{9}{2}\,;\,-\dfrac{9}{2} \right)\Rightarrow AH=\dfrac{9\sqrt{2}}{2} \\ & \overrightarrow{BC}=\left( 7-2a\,;\,7-2a \right)\Rightarrow BC=\left| 7-2a \right|.\sqrt{2} \\ \end{aligned} \right.$.

$\left( 1 \right)\Leftrightarrow \dfrac{1}{2}\left| 7-2a \right|\sqrt{2}\dfrac{9\sqrt{2}}{2}=18$

$\Leftrightarrow \left| 7-2a \right|=4\Leftrightarrow \left[ \begin{aligned} & 7-2a=4 \\ & 7-2a=-4 \\ \end{aligned} \right.\Leftrightarrow \left[ \begin{aligned} & a=\dfrac{3}{2} \\ & a=\dfrac{11}{2} \\ \end{aligned} \right.$.

Vậy $B\left( \dfrac{3}{2}\,;\,-\dfrac{5}{2} \right)$ và $C\left( \dfrac{11}{2}\,;\,\dfrac{3}{2} \right)$ hoặc $B\left( \dfrac{11}{2}\,;\,\dfrac{3}{2} \right)$ và $C\left( \dfrac{3}{2}\,;\,-\dfrac{5}{2} \right)$.

Hướng dẫn giải:

Theo bất đẳng thức Cosi ta có:

$\begin{aligned} & {{x}^{3}}+{{x}^{3}}+\dfrac{1}{3}\ge 3.\sqrt[3]{{{x}^{3}}.{{x}^{3}}.\dfrac{1}{3}}=\sqrt[3]{9}{{x}^{2}}\,\,\,\,\left( 1 \right) \\ & {{y}^{3}}+{{y}^{3}}+\dfrac{1}{3}\ge 3.\sqrt[3]{{{y}^{3}}.{{y}^{3}}.\dfrac{1}{3}}=\sqrt[3]{9}{{y}^{2}}\,\,\,\left( 2 \right) \\ & {{z}^{3}}+{{z}^{3}}+\dfrac{1}{3}\ge 3.\sqrt[3]{{{z}^{3}}.{{z}^{3}}.\dfrac{1}{3}}=\sqrt[3]{9}{{z}^{2}}\,\,\,\,\,\,\left( 3 \right) \\ \end{aligned}$

Cộng vế với vế của $\left( 1 \right),\left( 2 \right),\left( 3 \right)$ ta có $2\left( {{x}^{3}}+{{y}^{3}}+{{x}^{3}} \right)+1\ge \sqrt[3]{9}\left( {{x}^{2}}+{{y}^{2}}\,\,+{{z}^{2}} \right)$.

Theo giả thiết suy ra $S={{x}^{2}}+{{y}^{2}}+{{z}^{2}}\le \sqrt[3]{3}$.

Vậy giá trị lớn nhất của $S=\sqrt[3]{3}\Leftrightarrow x=y=z=\dfrac{1}{\sqrt[3]{3}}$.